What's The Square Root Of 33
listenit
Apr 02, 2025 · 5 min read
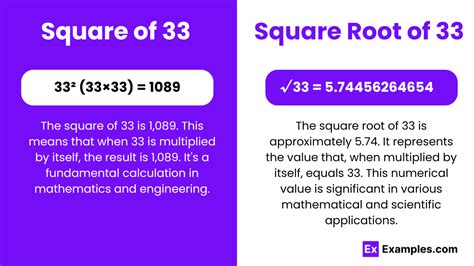
Table of Contents
What's the Square Root of 33? A Deep Dive into Irrational Numbers
The seemingly simple question, "What's the square root of 33?" opens a fascinating exploration into the world of mathematics, specifically the realm of irrational numbers. While a quick calculator search provides a decimal approximation, understanding the nature of √33 goes far beyond a simple numerical answer. This article delves into the intricacies of this specific square root, exploring its properties, calculation methods, and its broader significance within the mathematical landscape.
Understanding Square Roots and Irrational Numbers
Before we tackle the square root of 33 specifically, let's establish a foundational understanding of square roots and the classification of numbers. A square root of a number x is a value that, when multiplied by itself, equals x. For example, the square root of 9 (√9) is 3 because 3 * 3 = 9.
Not all numbers have neat, whole-number square roots. Numbers whose square roots are not whole numbers are often classified as irrational numbers. Irrational numbers cannot be expressed as a simple fraction (a ratio of two integers). Their decimal representations are non-terminating and non-repeating, meaning they go on forever without ever settling into a predictable pattern. The square root of 33 falls squarely into this category.
Calculating the Square Root of 33
While √33 doesn't have a precise, finite decimal representation, we can approximate its value using various methods.
1. Using a Calculator
The most straightforward approach is using a calculator. Most scientific calculators will provide a close approximation, usually around 5.74456. However, keep in mind this is just an approximation; the true value extends infinitely.
2. The Babylonian Method (or Heron's Method)
This iterative method provides increasingly accurate approximations. It starts with an initial guess and refines it through successive calculations.
- Step 1: Make an initial guess. Let's guess 6.
- Step 2: Improve the guess. Divide 33 by the initial guess (33/6 ≈ 5.5).
- Step 3: Average the guess and the result. Average 6 and 5.5: (6 + 5.5)/2 = 5.75.
- Step 4: Repeat steps 2 and 3. Divide 33 by 5.75 (33/5.75 ≈ 5.739), then average 5.75 and 5.739: (5.75 + 5.739)/2 ≈ 5.7445.
- Step 5: Continue iterating. Each iteration brings you closer to the actual value of √33.
The Babylonian method demonstrates a practical way to approximate the square root without relying solely on a calculator. The more iterations you perform, the more precise your approximation becomes.
3. Using a Numerical Method (e.g., Newton-Raphson Method)
More advanced numerical methods like the Newton-Raphson method offer even faster convergence to the actual value. These methods employ calculus and are generally implemented using computer programs.
The Significance of Irrational Numbers: Beyond √33
The fact that √33 is irrational isn't just a mathematical curiosity; it highlights a fundamental aspect of number systems. The discovery of irrational numbers challenged ancient Greek mathematicians' understanding of numbers and proportions. The existence of irrational numbers implies that there are gaps within the number line—points that cannot be precisely represented by ratios of integers.
Irrational Numbers in Geometry
Irrational numbers frequently arise in geometric contexts. For instance, the diagonal of a square with sides of length 1 is √2, an irrational number. Similarly, the ratio of a circle's circumference to its diameter (π) is another famous irrational number. The presence of irrational numbers in geometric calculations underscores the inherent limitations of representing certain lengths and ratios using simple fractions.
The Density of Irrational Numbers
While it might seem that rational numbers (fractions) are more common, the reality is quite different. Mathematicians have proven that irrational numbers are far more numerous than rational numbers. They are "dense" on the number line, meaning you can always find an irrational number between any two rational numbers.
Applications of Square Roots and Irrational Numbers
Square roots, including the irrational ones, have widespread applications across various fields:
- Physics: Calculations involving vectors, velocity, and acceleration frequently involve square roots.
- Engineering: Design and construction projects utilize square roots in structural calculations and geometric analysis.
- Computer Graphics: Rendering algorithms and simulations use square roots extensively.
- Finance: Calculating compound interest and determining investment returns often involve square roots.
- Statistics: Standard deviation, a crucial measure of data dispersion, involves calculating square roots.
Conclusion: Embracing the Approximation
The square root of 33, like many other irrational numbers, doesn't have a perfectly precise decimal representation. However, the pursuit of its approximation opens doors to exploring fundamental mathematical concepts, numerical methods, and the intriguing nature of irrational numbers. Whether you use a calculator for a quick estimate or delve into iterative methods for a deeper understanding, appreciating the approximate nature of √33 enriches our understanding of the number system and its role in diverse fields. Remember, even though we can't express it perfectly, the value of √33 is as real and meaningful as any other number on the number line. Its inherent irrationality simply reflects the richness and complexity of the mathematical universe.
Keywords:
square root of 33, irrational numbers, square root calculation, Babylonian method, Heron's method, Newton-Raphson method, numerical methods, approximation, mathematical concepts, geometry, applications of square roots, physics, engineering, computer graphics, finance, statistics, decimal representation, non-terminating decimal, non-repeating decimal.
Related Searches:
how to calculate square root, irrational numbers explained, square root approximation methods, applications of irrational numbers, history of irrational numbers, understanding square roots, what is an irrational number.
Latest Posts
Latest Posts
-
What Is The Difference Between Psychology And Physiology
Apr 03, 2025
-
What Is The Square Root Of One Fourth
Apr 03, 2025
-
A Quadrilateral With Two Right Angles
Apr 03, 2025
-
What Is The Measure Of Angle X
Apr 03, 2025
-
Where Is Most Of The Mass In An Atom Found
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about What's The Square Root Of 33 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
