What Is The Value Of X In The Figure
listenit
Mar 30, 2025 · 5 min read
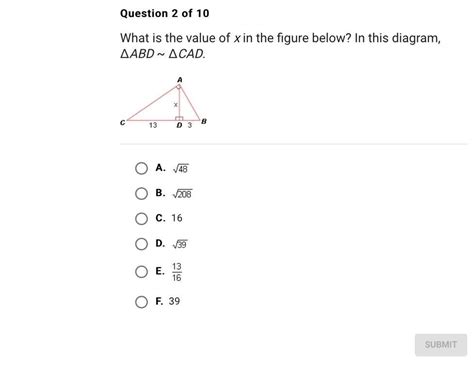
Table of Contents
What is the Value of x in the Figure? A Comprehensive Guide to Solving Geometric Problems
Determining the value of 'x' in a geometric figure is a fundamental skill in mathematics. It underpins numerous applications, from simple geometry problems to complex engineering calculations. This comprehensive guide will explore various techniques for solving for 'x' in different geometric contexts, focusing on clarity and providing a step-by-step approach for different scenarios. We'll cover crucial concepts, such as angles, triangles, circles, and polygons, equipping you with the knowledge to tackle a wide range of problems.
Understanding the Fundamentals: Angles and Their Relationships
Before diving into specific examples, let's refresh our understanding of fundamental geometric concepts. The value of 'x' often hinges on relationships between angles. Key concepts include:
1. Complementary Angles:
Complementary angles add up to 90 degrees. If you have two angles, A and B, and they are complementary, then:
A + B = 90°
If 'x' is part of a complementary angle pair, you can solve for it using this equation.
2. Supplementary Angles:
Supplementary angles add up to 180 degrees. For angles C and D:
C + D = 180°
This relationship is crucial when dealing with angles on a straight line or vertically opposite angles.
3. Vertically Opposite Angles:
When two lines intersect, the vertically opposite angles are equal. If angles E and F are vertically opposite:
E = F
This simplifies solving for 'x' if 'x' represents a vertically opposite angle.
4. Angles in a Triangle:
The sum of angles in any triangle always equals 180 degrees. For angles G, H, and I in a triangle:
G + H + I = 180°
This is a cornerstone for solving many geometric problems involving triangles.
5. Angles in a Polygon:
The sum of interior angles in a polygon with 'n' sides is given by:
(n - 2) * 180°
For example, a quadrilateral (4 sides) has (4-2) * 180° = 360° total interior angles. This formula is essential for solving problems involving polygons.
6. Isosceles Triangles:
In an isosceles triangle, two angles are equal. This equality often helps establish relationships that enable you to find 'x'.
7. Equilateral Triangles:
An equilateral triangle has all three angles equal to 60 degrees. Knowing this can greatly simplify problem-solving.
Solving for 'x' in Different Geometric Figures
Now, let's apply these concepts to various geometric figures and demonstrate how to find the value of 'x'.
Example 1: Solving for 'x' in a Triangle
Imagine a triangle with angles: x, (2x + 10)°, and (3x - 20)°. To find 'x', we use the fact that the sum of angles in a triangle equals 180 degrees:
x + (2x + 10) + (3x - 20) = 180
6x - 10 = 180
6x = 190
x = 190/6 = 95/3 ≈ 31.67°
Example 2: Solving for 'x' in Intersecting Lines
Consider two intersecting lines forming vertically opposite angles. One angle is 3x°, and the vertically opposite angle is 75°. Since vertically opposite angles are equal:
3x = 75
x = 75/3 = 25°
Example 3: Solving for 'x' in a Quadrilateral
Suppose we have a quadrilateral with angles: x, (x + 30)°, (2x - 40)°, and (3x + 10)°. The sum of angles in a quadrilateral is 360 degrees:
x + (x + 30) + (2x - 40) + (3x + 10) = 360
7x = 360
x = 360/7 ≈ 51.43°
Example 4: Solving for 'x' in a Circle
Circles introduce new relationships involving angles and arcs. For instance, if 'x' represents the angle subtended by an arc at the center of a circle, and another angle 'y' represents the angle subtended by the same arc at the circumference, then:
x = 2y
This means the angle at the center is twice the angle at the circumference. If you know either 'x' or 'y', you can easily solve for the other.
Example 5: Solving for x using Similar Triangles
Similar triangles have the same angles, although their sizes may differ. The ratios of corresponding sides are equal. This property is incredibly valuable in solving for 'x' when dealing with similar triangles. If we have two similar triangles with sides a, b, c and A, B, C respectively, then:
a/A = b/B = c/C
This allows us to set up equations to solve for an unknown side or angle (represented by 'x').
Example 6: Solving for 'x' in a Parallelogram
In a parallelogram, opposite angles are equal, and adjacent angles are supplementary. If 'x' is an angle in a parallelogram, and another angle is known, you can use these properties to solve for 'x'.
Advanced Techniques and Problem-Solving Strategies
Solving for 'x' in more complex geometric figures often requires a combination of techniques. Here are some advanced strategies:
-
Breaking Down Complex Figures: Divide a complex figure into simpler shapes (triangles, quadrilaterals, etc.). Solve for 'x' in the simpler shapes and then combine the results to solve the overall problem.
-
Using Auxiliary Lines: Sometimes, drawing an auxiliary line can create new relationships and simplify the problem.
-
Applying Trigonometric Functions: For problems involving right-angled triangles, trigonometric functions (sine, cosine, tangent) can be invaluable in finding 'x'.
-
Geometric Theorems: Familiarize yourself with various geometric theorems (e.g., Pythagorean theorem, Thales' theorem) to identify relationships and simplify the solution process.
-
Systematic Approach: A step-by-step approach, clearly outlining each step and the reasoning behind it, is crucial for avoiding mistakes and improving problem-solving skills.
Practical Applications and Real-World Examples
The ability to solve for 'x' in geometric figures has wide-ranging applications in various fields:
-
Engineering: Calculating angles and distances in structural design, surveying, and other engineering disciplines.
-
Architecture: Designing buildings and structures with precise angles and dimensions.
-
Computer Graphics: Creating realistic 3D models and animations.
-
Cartography: Creating accurate maps and projections.
-
Physics: Solving problems involving forces, vectors, and motion.
Conclusion
Solving for 'x' in geometric figures is a fundamental mathematical skill with widespread applications. By mastering the concepts presented in this guide, including angles, triangles, polygons, and circles, along with various problem-solving strategies, you will be well-equipped to tackle a wide range of geometric problems. Remember to practice consistently, and gradually work your way up to more complex problems. With dedication and a systematic approach, you will confidently determine the value of 'x' in even the most challenging geometric figures.
Latest Posts
Latest Posts
-
What Is The Name Of The Positively Charged Subatomic Particle
Apr 01, 2025
-
Why Does Bone Heal Faster Than Cartilage
Apr 01, 2025
-
What Is Lcm Of 5 And 6
Apr 01, 2025
-
How Many 1 3 Cups Equal 2 3 Cup
Apr 01, 2025
-
How To Find Complement And Supplement Of An Angle
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about What Is The Value Of X In The Figure . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
