Rectangle That Is Not A Parallelogram
listenit
Apr 04, 2025 · 5 min read
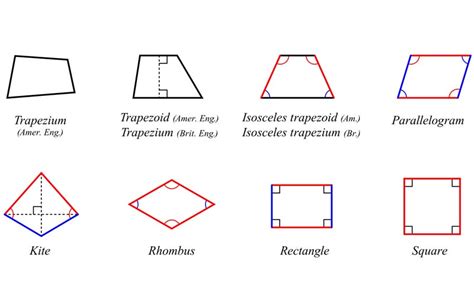
Table of Contents
A Rectangle That's Not a Parallelogram? Exploring the Paradox
The statement "a rectangle that is not a parallelogram" sounds inherently contradictory. After all, a rectangle is, by definition, a parallelogram with specific additional properties. However, delving into the nuances of geometric definitions and exploring different mathematical contexts reveals that this seeming paradox can be approached in several intriguing ways. This article will explore this intriguing concept, examining the traditional understanding of rectangles and parallelograms, and then venturing into alternative interpretations that might allow for a rectangle to exist outside the classification of parallelograms – albeit within specific, carefully defined frameworks.
Understanding the Standard Definitions
Before we explore the unconventional, let's solidify our understanding of the standard geometric definitions:
Parallelogram: A quadrilateral (a four-sided polygon) with opposite sides parallel. This means that the lines forming the opposite sides would never intersect if extended infinitely. Key properties include opposite sides being equal in length and opposite angles being equal in measure.
Rectangle: A parallelogram with four right angles (90-degree angles). This means all interior angles are exactly 90 degrees. Importantly, the definition explicitly states that a rectangle is a type of parallelogram. This inherent inclusion is the source of the apparent paradox.
Therefore, under standard Euclidean geometry, a rectangle cannot exist without also being a parallelogram. The core characteristics of a rectangle are built upon the foundational properties of a parallelogram. It's a subset, a more specific category within the broader category of parallelograms. Attempting to define a rectangle that isn't a parallelogram in this context would be like trying to define a square that's not a rectangle – it's logically inconsistent.
Exploring Potential Interpretations and Loopholes
To reconcile the apparent contradiction, we must consider contexts outside the strict rules of classical Euclidean geometry. Let's investigate several potential interpretations:
1. Non-Euclidean Geometries
Euclidean geometry, the geometry we commonly learn in school, operates under specific axioms and postulates. Non-Euclidean geometries, such as spherical or hyperbolic geometry, challenge these axioms. In these geometries, the rules governing parallel lines and angles differ significantly.
Imagine a rectangle drawn on the surface of a sphere. The "sides" of the rectangle would follow great circles (the shortest distance between two points on a sphere). While we might still call it a "rectangle" based on its visual resemblance in a localized view, the strict definition of parallel lines breaks down. The "sides" are not truly parallel in the Euclidean sense; they meet at the poles. Thus, under this interpretation, we could arguably have a shape that visually resembles a rectangle but fails to be a parallelogram in the Euclidean definition. However, it's important to note that this is due to the fundamental differences in the underlying geometric framework, not a violation within the framework itself.
2. Degenerate Cases and Limiting Situations
We can also consider degenerate cases or limiting situations. Imagine a rectangle with one side shrinking to an infinitesimal length. As the length approaches zero, the shape approaches a line segment. While this limiting shape would resemble a degenerate rectangle, its properties would deviate significantly from those of a parallelogram. It ceases to have well-defined opposite sides.
3. Ambiguity in Language and Definition
Sometimes, the paradox arises from ambiguity in the language used to define geometric shapes. A lax or imprecise definition of "rectangle" might open the door to misinterpretations. However, for mathematical rigor, we must rely on precise definitions, and under standard geometric definitions, the contradiction persists.
4. Alternative Axiomatic Systems
Consider the possibility of a completely different axiomatic system for geometry. We might create a system where the definitions of parallelograms and rectangles are modified, allowing for the existence of rectangles that do not fulfill the traditional parallelogram criteria. This approach would essentially define a new geometric system where the classical implications of the definitions are no longer valid. This is a more advanced theoretical exploration.
The Importance of Precise Definitions in Mathematics
The seemingly paradoxical statement highlights the crucial role of precise definitions in mathematics. Ambiguity and imprecise language can lead to logical contradictions and misunderstandings. Mathematical rigor demands clarity and careful articulation of concepts. The core issue is the inherent relationship between rectangles and parallelograms as established in classical Euclidean geometry. Any attempt to break this relationship requires a fundamental shift in the underlying mathematical framework.
Conclusion: A Matter of Perspective and Context
The question of a rectangle that is not a parallelogram is ultimately a matter of perspective and the context in which we define these geometric concepts. Within the framework of standard Euclidean geometry, the statement is a contradiction. However, exploring non-Euclidean geometries, degenerate cases, or the potential for entirely new axiomatic systems, allows us to approach the question from different angles and uncover interesting insights into the nature of geometric definitions and the flexibility of mathematical reasoning. The apparent paradox serves as a valuable lesson in the importance of precise language and the limitations of applying concepts outside their established framework.
While the initial statement seems counterintuitive, the investigation has demonstrated the richness and complexity hidden within seemingly simple geometric concepts. It encourages critical thinking and a deeper understanding of the fundamental principles underlying geometric definitions and their application in various mathematical contexts. This exploration underscores the need for careful consideration of definitions and the potential consequences of linguistic ambiguity, especially in fields requiring precision, like mathematics and computer science. The quest to reconcile a "rectangle that is not a parallelogram" pushes the boundaries of our geometric understanding and provides a fascinating case study in the interplay between definitions, axioms, and interpretations.
Latest Posts
Latest Posts
-
Anti Derivative Of Square Root Of X
Apr 05, 2025
-
Greatest Common Factor Of 24 And 36
Apr 05, 2025
-
What Is 3 4 5 As A Decimal
Apr 05, 2025
-
What Is The Range Of The Data Below
Apr 05, 2025
-
What Is The Electron Configuration Of Ga
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about Rectangle That Is Not A Parallelogram . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
