Anti Derivative Of Square Root Of X
listenit
Apr 05, 2025 · 6 min read
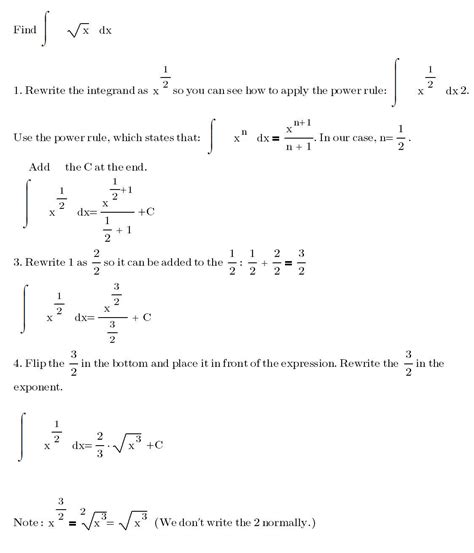
Table of Contents
Finding the Antiderivative of the Square Root of x: A Comprehensive Guide
The seemingly simple function √x, or x<sup>1/2</sup>, presents a fascinating challenge when we delve into its antiderivative. While differentiation often feels straightforward, integration can be trickier, demanding a deeper understanding of fundamental calculus concepts. This comprehensive guide will explore the antiderivative of √x, explaining the process meticulously, offering alternative approaches, and highlighting its applications in various fields.
Understanding Antiderivatives
Before diving into the specifics of √x, let's establish a solid foundation. The antiderivative, also known as the indefinite integral, is the inverse operation of differentiation. Where differentiation finds the instantaneous rate of change of a function, integration finds the function whose derivative is the given function. We denote the antiderivative of a function f(x) as ∫f(x)dx. The 'dx' signifies that we are integrating with respect to the variable x. Crucially, the antiderivative is not unique; adding any constant (C) to the antiderivative still results in a function whose derivative is f(x). This constant of integration, C, accounts for the numerous possible functions sharing the same derivative.
Calculating the Antiderivative of √x using the Power Rule
The most direct method for finding the antiderivative of √x is to apply the power rule of integration, a direct consequence of the power rule of differentiation. The power rule states:
∫x<sup>n</sup> dx = (x<sup>n+1</sup>)/(n+1) + C, where n ≠ -1
In our case, √x = x<sup>1/2</sup>, so n = 1/2. Substituting this into the power rule:
∫x<sup>1/2</sup> dx = (x<sup>(1/2)+1</sup>)/((1/2)+1) + C
Simplifying:
∫x<sup>1/2</sup> dx = (x<sup>3/2</sup>)/(3/2) + C
Further simplification gives:
∫√x dx = (2/3)x<sup>3/2</sup> + C
This is the antiderivative of √x. The (2/3) is the coefficient resulting from the reciprocal of (3/2), and C represents the constant of integration.
Verifying the Antiderivative through Differentiation
To confirm our result, let's differentiate (2/3)x<sup>3/2</sup> + C. Applying the power rule of differentiation:
d/dx [(2/3)x<sup>3/2</sup> + C] = (2/3) * (3/2)x<sup>(3/2)-1</sup> + 0
This simplifies to:
x<sup>1/2</sup> = √x
This confirms that (2/3)x<sup>3/2</sup> + C is indeed the antiderivative of √x.
Visualizing the Antiderivative
A graphical representation can provide valuable insight. The graph of √x is a curve starting at the origin and increasing monotonically. The antiderivative, (2/3)x<sup>3/2</sup> + C, represents a family of curves, each differing only by a vertical shift determined by the constant C. These curves represent the accumulation of area under the √x curve. Different values of C simply shift this area vertically without altering the shape.
Alternative Approaches and Techniques
While the power rule offers the most straightforward solution, alternative approaches can enhance our understanding.
Riemann Sums
Riemann sums, while computationally intensive, provide a fundamental understanding of integration. By dividing the area under the curve of √x into an increasing number of smaller rectangles, we can approximate the area, which approaches the exact value as the number of rectangles goes to infinity. Although not a practical method for finding the antiderivative directly, understanding Riemann sums strengthens the conceptual underpinnings of integration.
Numerical Integration Techniques
Numerical techniques such as the Trapezoidal Rule and Simpson's Rule offer approximate solutions for definite integrals (integrals with specified limits of integration). While they don't yield the antiderivative directly, they provide numerical approximations valuable in scenarios where finding an analytical solution proves difficult or impossible.
Applications of the Antiderivative of √x
The antiderivative of √x finds practical applications across diverse fields.
Physics: Calculating Displacement from Velocity
In physics, if √x represents the velocity of an object as a function of time, the antiderivative (2/3)x<sup>3/2</sup> + C represents the displacement of the object. The constant C represents the initial displacement at time t=0.
Engineering: Calculating Area and Volume
In engineering, the antiderivative finds use in calculating areas and volumes of curved shapes. For example, the area under the curve √x between two points can be determined using definite integration. The concept extends to volume calculations, particularly when dealing with solids of revolution.
Statistics: Probability Density Functions
In statistics, the antiderivative of a probability density function (PDF) yields the cumulative distribution function (CDF). If √x were part of a PDF, its antiderivative would play a crucial role in calculating probabilities and percentiles.
Economics: Marginal and Total Cost/Revenue
In economics, if √x represents a marginal cost function, its antiderivative represents the total cost function, providing valuable insights into the total costs associated with producing a certain quantity of goods. Similar interpretations apply to marginal and total revenue functions.
Definite Integrals and the Fundamental Theorem of Calculus
While this guide has focused on the indefinite integral (antiderivative), the definite integral, represented as ∫<sub>a</sub><sup>b</sup> √x dx, finds the area under the curve √x between the limits of integration 'a' and 'b'. The Fundamental Theorem of Calculus establishes a critical link between the definite and indefinite integrals. It states that the definite integral of a function is the difference between the values of its antiderivative at the upper and lower limits of integration.
Applying the Fundamental Theorem of Calculus to our example:
∫<sub>a</sub><sup>b</sup> √x dx = [(2/3)x<sup>3/2</sup>]<sub>a</sub><sup>b</sup> = (2/3)b<sup>3/2</sup> - (2/3)a<sup>3/2</sup>
This result provides a direct method for calculating the area under the curve √x between any two points.
Advanced Considerations
The antiderivative of √x, while straightforward for simple applications, can become more intricate within the context of multivariate calculus, where integration is performed across multiple dimensions. Techniques such as multiple integrals and integration in polar or cylindrical coordinates may become necessary for complex problems. The concept of line integrals, surface integrals, and volume integrals all build upon the fundamental principles of antiderivatives, extending the scope and applicability of the concepts discussed here.
Furthermore, the antiderivative of more complex functions involving √x, such as ∫√(x²+a²) dx or ∫√(ax+b) dx, require different integration techniques like trigonometric substitution or u-substitution.
Conclusion
The seemingly simple function √x offers a compelling illustration of the power and versatility of integration. While applying the power rule is straightforward, understanding the underlying concepts, visualizing the results, and appreciating the diverse applications underscores the importance of mastering integration techniques. From basic physics problems to sophisticated economic models, the ability to find the antiderivative of √x (and other functions) becomes an essential tool across a wide range of disciplines. This guide has strived to provide a thorough understanding of this seemingly simple yet profoundly significant mathematical concept. The journey from understanding the power rule to applying the fundamental theorem of calculus opens the door to a deeper appreciation of calculus and its applications. Remember, the constant practice and exploration of various integration methods are crucial to building a strong foundation in calculus.
Latest Posts
Latest Posts
-
Five Examples Of Combustion In Everyday Life
Apr 05, 2025
-
How Many Pi Bonds Are In A Triple Bond
Apr 05, 2025
-
How To Find Area Of Parallelogram With Vectors
Apr 05, 2025
-
Why Do Contour Lines Never Cross
Apr 05, 2025
-
Is Boron A Gas Solid Or Liquid
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about Anti Derivative Of Square Root Of X . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
