Difference Between Rational And Real Numbers
listenit
Apr 04, 2025 · 6 min read
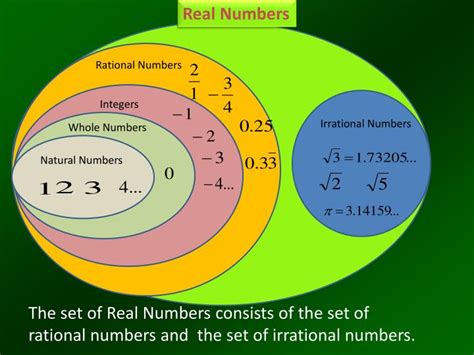
Table of Contents
Delving Deep into the Difference Between Rational and Real Numbers
The world of mathematics is vast and intricate, encompassing a wide array of number systems. Understanding the nuances between these systems is crucial for anyone aiming to grasp mathematical concepts fully. Two fundamental number systems frequently encountered are rational numbers and real numbers. While seemingly straightforward, the distinctions between them are often subtle yet incredibly important. This comprehensive guide will explore the differences between rational and real numbers, providing a clear understanding of their properties, characteristics, and applications.
What are Rational Numbers?
Rational numbers, denoted by Q, are numbers that can be expressed as a fraction p/q, where p and q are integers, and q is not equal to zero. This definition encapsulates a broad range of numbers, including:
-
Integers: Whole numbers, including positive integers (1, 2, 3...), negative integers (-1, -2, -3...), and zero (0). Integers can be expressed as fractions where the denominator is 1 (e.g., 3 = 3/1, -2 = -2/1).
-
Fractions: Numbers expressed as ratios of two integers, such as 1/2, 3/4, -2/5. These clearly fit the p/q definition.
-
Terminating Decimals: Decimals that have a finite number of digits after the decimal point. For example, 0.25 (which is 1/4), 0.75 (which is 3/4), and 0.125 (which is 1/8). These can always be converted into fractions.
-
Repeating Decimals: Decimals with a digit or group of digits that repeat infinitely. For example, 0.333... (which is 1/3), 0.142857142857... (which is 1/7), and 0.1666... (which is 1/6). Even though the decimal representation is infinite, these can also be expressed as a fraction.
Key Characteristics of Rational Numbers:
-
Expressible as a fraction: This is the defining characteristic. If a number can be written as a fraction of two integers (with a non-zero denominator), it is rational.
-
Finite or repeating decimal representation: Their decimal form either terminates after a finite number of digits or repeats a sequence of digits indefinitely.
-
Denseness: Between any two rational numbers, there exists another rational number. This property implies that rational numbers are densely packed on the number line.
What are Real Numbers?
Real numbers, denoted by R, encompass a far broader set of numbers than rational numbers. Real numbers include all rational numbers and all irrational numbers. This means the set of real numbers contains:
-
All Rational Numbers: As discussed above, this includes integers, fractions, terminating decimals, and repeating decimals.
-
Irrational Numbers: Numbers that cannot be expressed as a fraction of two integers. Their decimal representations are neither terminating nor repeating. Examples of irrational numbers include:
-
√2: The square root of 2, approximately 1.41421356... Its decimal expansion is infinite and non-repeating.
-
π (Pi): The ratio of a circle's circumference to its diameter, approximately 3.14159265... It's another famous example of an infinite, non-repeating decimal.
-
e (Euler's number): The base of the natural logarithm, approximately 2.71828... This transcendental number also has an infinite, non-repeating decimal expansion.
-
Transcendental Numbers: These are a special subset of irrational numbers that are not the root of any polynomial equation with rational coefficients. π and e are examples of transcendental numbers.
-
Key Characteristics of Real Numbers:
-
Completeness: The real number system is complete, meaning it contains all its limit points. This implies there are no "gaps" in the real number line.
-
Every point on the number line corresponds to a real number: This visually represents the completeness of the real number system. There is a one-to-one correspondence between points on the number line and real numbers.
-
Includes both rational and irrational numbers: This is the fundamental distinction between real and rational numbers; real numbers encompass all rational and irrational numbers.
The Crucial Differences: A Summary Table
| Feature | Rational Numbers (Q) | Real Numbers (R) |
|---|---|---|
| Definition | Numbers expressible as p/q, where p and q are integers, q ≠ 0 | All rational and irrational numbers |
| Decimal Form | Terminating or repeating decimals | Terminating, repeating, or non-repeating decimals |
| Examples | 1/2, -3, 0.75, 0.333... | 1/2, -3, 0.75, 0.333..., √2, π, e |
| Completeness | Not complete; has "gaps" | Complete; no "gaps" |
| Subset of | - | Contains all rational numbers |
Illustrative Examples and Applications
Let's solidify our understanding with a few examples:
Example 1: Is 2.71828... a rational or real number?
This is e, Euler's number. Its decimal representation is non-terminating and non-repeating, thus it's an irrational number, and therefore a real number.
Example 2: Is -5/3 a rational or real number?
-5/3 is a fraction where both the numerator and denominator are integers. Therefore, it's a rational number, and consequently also a real number.
Example 3: Is √9 a rational or real number?
√9 = 3, which is an integer. Integers are rational numbers, and all rational numbers are real numbers. Therefore, √9 is a rational number and a real number.
Applications in Real-World Scenarios:
The distinction between rational and real numbers has significant implications in various fields:
-
Computer Science: Computers can represent rational numbers with finite precision. However, representing irrational numbers precisely requires approximation techniques. This leads to limitations in computations involving irrational numbers like π.
-
Engineering and Physics: Many physical quantities are measured as real numbers, but their calculation often involves approximations, considering that truly precise measurement of irrational numbers is impossible in practice.
-
Geometry and Calculus: The concept of real numbers is fundamental to geometry (lengths, areas) and calculus (limits, derivatives, integrals). Irrational numbers appear frequently in geometrical calculations involving circles, and are indispensable in various calculus operations.
Exploring Further: Beyond the Basics
The concepts of rational and real numbers lay the groundwork for exploring more advanced mathematical ideas:
-
Complex Numbers: These numbers extend the real number system by introducing the imaginary unit i, where i² = -1. Complex numbers are expressed in the form a + bi, where a and b are real numbers.
-
Set Theory: The formal study of sets and their properties provides a rigorous framework for understanding the relationships between different number systems, including rational and real numbers.
Conclusion
The difference between rational and real numbers lies in their ability to be expressed as fractions. While all rational numbers are real numbers, the real number system also includes irrational numbers, which cannot be expressed as fractions of integers. This distinction, seemingly subtle, holds profound implications across various mathematical disciplines and real-world applications. Understanding this difference is fundamental to building a robust foundation in mathematics and its applications. By appreciating the properties and characteristics of both rational and real numbers, we unlock a deeper comprehension of the fascinating world of numbers and their significance in shaping our understanding of the universe.
Latest Posts
Latest Posts
-
What Is The Charge For Boron
Apr 04, 2025
-
How Many Electrons Are In Titanium
Apr 04, 2025
-
Two Thirds Of A Number Algebraic Expression
Apr 04, 2025
-
Is Photosynthesis A Chemical Or Physical Change
Apr 04, 2025
-
Convert 101 Degrees Fahrenheit To Celsius
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Difference Between Rational And Real Numbers . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
