Area Of A Circle With Radius 6
listenit
Apr 02, 2025 · 5 min read
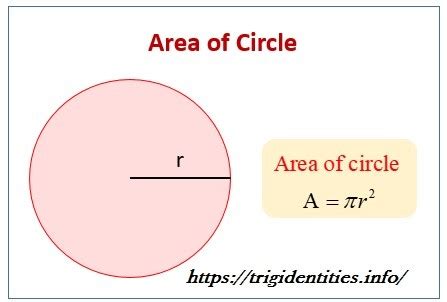
Table of Contents
Area of a Circle with Radius 6: A Comprehensive Guide
The area of a circle is a fundamental concept in geometry with widespread applications in various fields, from engineering and architecture to data science and computer graphics. This article delves deep into calculating the area of a circle, specifically when the radius is 6 units. We'll explore the formula, its derivation, practical applications, and even touch upon related geometrical concepts. We'll also cover how to effectively communicate this calculation in different contexts.
Understanding the Formula: πr²
The area of any circle is calculated using a simple yet elegant formula: A = πr². Where:
- A represents the area of the circle.
- π (pi) is a mathematical constant, approximately equal to 3.14159. It represents the ratio of a circle's circumference to its diameter. The value of π is irrational, meaning its decimal representation goes on forever without repeating.
- r represents the radius of the circle, which is the distance from the center of the circle to any point on the circle.
Calculating the Area with Radius 6
In our specific case, the radius (r) is 6 units. Let's plug this value into the formula:
A = π(6)² = π(36) = 36π
This is the exact area of the circle. However, for practical purposes, we often need a numerical approximation. Using a common approximation of π as 3.14159, we get:
A ≈ 36 * 3.14159 ≈ 113.097 square units
Therefore, the area of a circle with a radius of 6 units is approximately 113.097 square units. The units will depend on the unit of measurement used for the radius (e.g., square centimeters, square meters, square inches).
Derivation of the Area Formula: A Visual Approach
While the formula A = πr² is commonly accepted, understanding its derivation enhances comprehension. One way to visualize this is by dividing the circle into numerous, infinitesimally small sectors. Imagine slicing a pizza into a very large number of thin slices.
These sectors can then be rearranged to approximate a parallelogram. The base of this parallelogram is approximately half the circumference of the circle (½ * 2πr = πr), and the height is approximately equal to the radius (r).
The area of a parallelogram is base * height. Therefore, the approximate area of the circle becomes:
Area ≈ πr * r = πr²
As the number of sectors increases infinitely, the approximation becomes increasingly accurate, leading to the exact formula: A = πr².
Practical Applications of Circle Area Calculations
Calculating the area of a circle is crucial in numerous real-world scenarios:
1. Engineering and Construction:
-
Designing circular structures: Architects and engineers use the area formula to determine the material requirements for constructing circular buildings, roads, or bridges. Knowing the area helps in calculating the amount of concrete, asphalt, or other materials needed.
-
Pipe and conduit sizing: Determining the cross-sectional area of pipes and conduits is essential for calculating flow rates of liquids or gases. This is critical in designing efficient water supply systems, drainage systems, and ventilation systems.
2. Agriculture and Landscaping:
-
Irrigation system design: Farmers and landscapers use circle area calculations to determine the area covered by a circular irrigation system. This helps optimize water usage and ensure efficient coverage.
-
Planning planting areas: The area of a circle is crucial in determining the space needed for planting crops or trees in a circular pattern.
3. Data Science and Statistics:
-
Data visualization: Circles are frequently used in charts and graphs (like pie charts) to represent proportions or data distributions. The area of each circular segment represents a proportional part of the total data.
-
Probability and statistics: Circular areas play a role in certain probability distributions and statistical analyses.
4. Everyday Life:
-
Calculating pizza size: The area of a circle can help determine which pizza size offers the most value for money, considering the price per square inch.
-
Determining the coverage area of a sprinkler: Understanding the area covered by a sprinkler is vital for effective lawn watering.
Beyond the Basics: Related Geometrical Concepts
Understanding the area of a circle opens doors to exploring other related geometrical concepts:
1. Circumference:
The circumference (C) of a circle is the distance around it. It's related to the radius by the formula: C = 2πr. For a circle with a radius of 6, the circumference is approximately 37.699 units.
2. Diameter:
The diameter (d) of a circle is twice its radius: d = 2r. For a circle with a radius of 6, the diameter is 12 units.
3. Sector Area:
A sector is a portion of a circle enclosed by two radii and an arc. The area of a sector is a fraction of the circle's total area, determined by the central angle of the sector.
4. Segment Area:
A segment is a region bounded by a chord and an arc. Calculating the area of a segment involves subtracting the area of a triangle from the area of a sector.
Communicating the Calculation Effectively
Whether you're presenting this calculation in a scientific report, an engineering design document, or simply explaining it to a friend, clear communication is crucial. Here's how to do it effectively:
-
State the problem clearly: Begin by stating that you need to find the area of a circle with a radius of 6 units.
-
Show the formula: Write down the formula for the area of a circle: A = πr².
-
Substitute the values: Clearly show the substitution of the radius value (r = 6) into the formula: A = π(6)².
-
Calculate the area: Perform the calculation, showing each step: A = 36π ≈ 113.097 square units.
-
State the units: Always specify the units of measurement (square centimeters, square meters, etc.)
-
Use appropriate diagrams: A simple diagram showing the circle with its radius labeled enhances understanding.
-
Consider your audience: Tailor the complexity of your explanation to your audience's mathematical background.
Conclusion: Mastering Circle Area Calculations
The area of a circle with a radius of 6, approximately 113.097 square units, is a fundamental calculation with far-reaching applications. Understanding the formula, its derivation, and its practical uses is essential for anyone working with geometry or related fields. By mastering this concept and effectively communicating your calculations, you'll be well-equipped to tackle more complex geometrical problems and successfully apply this knowledge in various real-world scenarios. Remember the power of visualization and clear, step-by-step explanations to enhance your understanding and the clarity of your communication.
Latest Posts
Latest Posts
-
The Change In State From Liquid To Gas Is Called
Apr 03, 2025
-
How Is A Mole Similar To A Dozen
Apr 03, 2025
-
Which Is The Aqueous And Organic Layer
Apr 03, 2025
-
Is Soh Cah Toa Only For Right Triangles
Apr 03, 2025
-
How To Calculate The Heat Of Neutralization
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Area Of A Circle With Radius 6 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
