6 Of 15 Is What Percent
listenit
Mar 26, 2025 · 5 min read
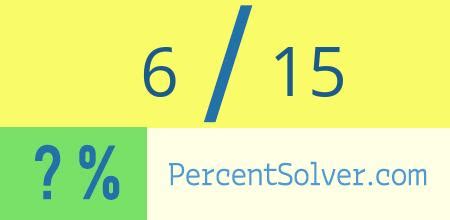
Table of Contents
6 out of 15 is What Percent? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous aspects of life, from calculating discounts and taxes to comprehending statistical data and analyzing financial reports. This article will delve deep into how to calculate percentages, focusing specifically on the question: "6 out of 15 is what percent?" We'll explore multiple methods, provide practical examples, and equip you with the knowledge to tackle similar percentage problems with confidence.
Understanding the Fundamentals of Percentages
A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per cent," meaning "out of 100." Therefore, 50% means 50 out of 100, which simplifies to 1/2 or 0.5. Understanding this basic concept is crucial for tackling percentage calculations.
Method 1: Using the Fraction Method
The most intuitive approach to solving "6 out of 15 is what percent?" involves converting the given numbers into a fraction and then transforming that fraction into a percentage.
-
Step 1: Form the Fraction: Express the problem as a fraction: 6/15.
-
Step 2: Simplify the Fraction (if possible): Both 6 and 15 are divisible by 3. Simplifying the fraction gives us 2/5. This step isn't mandatory but makes the subsequent calculation easier.
-
Step 3: Convert the Fraction to a Decimal: Divide the numerator (2) by the denominator (5): 2 ÷ 5 = 0.4
-
Step 4: Convert the Decimal to a Percentage: Multiply the decimal by 100 and add the "%" symbol: 0.4 x 100 = 40%.
Therefore, 6 out of 15 is 40%.
Method 2: Using the Proportion Method
The proportion method offers a slightly different approach to solving percentage problems, especially useful for more complex scenarios. It relies on setting up a proportion equation.
-
Step 1: Set up the Proportion: We know that 6 out of 15 is equivalent to 'x' out of 100. This can be written as a proportion:
6/15 = x/100
-
Step 2: Cross-Multiply: Cross-multiply the terms to solve for 'x':
15x = 600
-
Step 3: Solve for x: Divide both sides of the equation by 15:
x = 600/15 = 40
-
Step 4: Express as a Percentage: 'x' represents the percentage, so the answer is 40%.
Method 3: Using the Percentage Formula
A more direct formula-based approach can streamline the calculation. The formula is:
Percentage = (Part/Whole) * 100
-
Step 1: Identify the Part and the Whole: In our problem, the "part" is 6, and the "whole" is 15.
-
Step 2: Substitute into the Formula:
Percentage = (6/15) * 100
-
Step 3: Calculate:
Percentage = 0.4 * 100 = 40%
Real-World Applications of Percentage Calculations
Understanding how to calculate percentages is incredibly valuable in various real-life situations. Here are a few examples:
-
Sales and Discounts: If a store offers a 20% discount on an item priced at $50, you can easily calculate the discount amount and the final price.
-
Taxes: Calculating sales tax or income tax involves percentage calculations.
-
Grades and Scores: Understanding percentages is crucial for interpreting grades in school or scores on tests.
-
Financial Analysis: Analyzing financial statements, such as profit margins or return on investment, necessitates a strong understanding of percentages.
-
Statistical Data: Percentages are frequently used to represent data in charts and graphs, allowing for easier comprehension and comparison.
-
Surveys and Polls: Results from surveys and polls are often expressed as percentages to illustrate the proportion of respondents who chose a particular option.
-
Cooking and Baking: Adjusting recipes often involves using percentages to scale ingredients up or down.
Advanced Percentage Problems and Their Solutions
While the problem "6 out of 15 is what percent?" is relatively straightforward, let's explore some more complex percentage-related scenarios:
Scenario 1: Finding the Whole when the Percentage and Part are Known
Let's say you know that 30% of a certain number is 12. How do you find the total number?
-
Step 1: Set up an Equation: Let 'x' be the total number. We can set up the equation: 0.30x = 12
-
Step 2: Solve for x: Divide both sides by 0.30: x = 12 / 0.30 = 40. Therefore, the total number is 40.
Scenario 2: Finding the Percentage Increase or Decrease
Suppose a price increases from $25 to $30. What is the percentage increase?
-
Step 1: Calculate the Difference: The difference is $30 - $25 = $5
-
Step 2: Calculate the Percentage Increase: ($5/$25) * 100 = 20%. The price increased by 20%.
Scenario 3: Calculating Percentage Points
Percentage points refer to the arithmetic difference between two percentages. For example, if the interest rate rises from 5% to 8%, it's an increase of 3 percentage points, not 3%. This distinction is crucial in financial contexts.
Tips for Mastering Percentage Calculations
-
Practice regularly: The more you practice, the more comfortable you'll become with percentage calculations.
-
Use different methods: Try solving problems using different methods (fraction, proportion, formula) to find the approach that best suits your understanding.
-
Break down complex problems: Divide complex problems into smaller, manageable steps.
-
Check your work: Always double-check your calculations to ensure accuracy.
-
Utilize online resources: Numerous online calculators and tutorials can help you practice and reinforce your understanding.
-
Understand the context: Pay close attention to the context of the problem to ensure you're applying the correct method and interpreting the results accurately.
Conclusion
The question "6 out of 15 is what percent?" serves as an excellent starting point for understanding percentage calculations. By mastering the various methods outlined in this article – the fraction method, proportion method, and formula method – you'll be well-equipped to tackle a wide range of percentage problems encountered in various aspects of life. Remember to practice regularly, break down complex problems, and utilize available resources to solidify your understanding and build confidence in your ability to work with percentages. This fundamental skill will prove invaluable across diverse fields, from personal finance to professional settings.
Latest Posts
Latest Posts
-
What Is 65 As A Decimal
Mar 29, 2025
-
What Is The Lowest Common Multiple Of 7 And 5
Mar 29, 2025
-
How Many Electrons Can The F Orbital Hold
Mar 29, 2025
-
68 Grams Is How Many Ounces
Mar 29, 2025
-
What Is The Square Root Of Negative 4
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about 6 Of 15 Is What Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
