What Is The Square Root Of Negative 4
listenit
Mar 29, 2025 · 5 min read
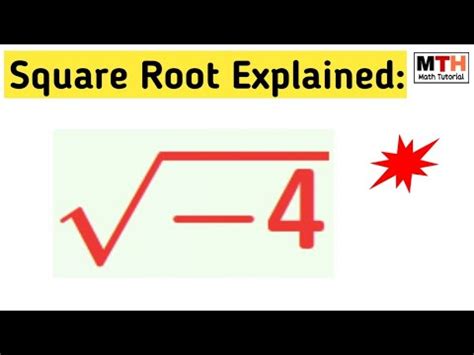
Table of Contents
What is the Square Root of Negative 4? Delving into Imaginary Numbers
The question, "What is the square root of negative 4?" might seem simple at first glance. After all, we all learned in school that the square root of a number is a value that, when multiplied by itself, equals the original number. However, the introduction of a negative number under the square root sign throws a significant wrench into this seemingly straightforward concept, leading us into the fascinating world of imaginary numbers.
Understanding the Basics: Real Numbers and Square Roots
Before diving into the complexities of negative square roots, let's briefly review the basics of real numbers and square roots. Real numbers encompass all the numbers we typically encounter, including positive and negative whole numbers, fractions, decimals, and irrational numbers like π (pi). The square root of a real number, denoted as √x, is a value that, when multiplied by itself, equals x. For example:
- √9 = 3 because 3 * 3 = 9
- √16 = 4 because 4 * 4 = 16
- √0.25 = 0.5 because 0.5 * 0.5 = 0.25
However, what happens when we try to find the square root of a negative number? This is where the concept of imaginary numbers becomes essential. No real number, when multiplied by itself, will ever result in a negative number. This is because the product of two positive numbers is positive, and the product of two negative numbers is also positive.
Introducing Imaginary Numbers: The "i"
To address this limitation, mathematicians introduced the concept of the imaginary unit, denoted by the letter "i". i is defined as the square root of -1:
√-1 = i
This might seem arbitrary, but it's a crucial definition that allows us to extend the concept of square roots to negative numbers. Once we have "i," we can express the square root of any negative number in terms of "i."
Calculating the Square Root of -4
Now, let's tackle the original question: What is the square root of -4? We can rewrite √-4 as follows:
√-4 = √(4 * -1) = √4 * √-1
Since √4 = 2 and √-1 = i, we can simplify this expression to:
√-4 = 2i
Therefore, the square root of negative 4 is 2i. It's not a real number; it's an imaginary number.
Complex Numbers: Combining Real and Imaginary
The combination of real and imaginary numbers forms what we call complex numbers. A complex number is expressed in the form:
a + bi
where "a" is the real part and "b" is the imaginary part. For example:
- 3 + 2i is a complex number (real part = 3, imaginary part = 2)
- -5 - i is a complex number (real part = -5, imaginary part = -1)
- 7i is a complex number (real part = 0, imaginary part = 7)
Notice that real numbers are simply complex numbers where the imaginary part (b) is zero, and imaginary numbers are complex numbers where the real part (a) is zero.
Operations with Imaginary and Complex Numbers
Just like real numbers, imaginary and complex numbers can be added, subtracted, multiplied, and divided. Here's a brief overview of these operations:
Addition and Subtraction:
To add or subtract complex numbers, simply add or subtract their real parts and their imaginary parts separately. For example:
(3 + 2i) + (1 - i) = (3 + 1) + (2 - 1)i = 4 + i
Multiplication:
When multiplying complex numbers, use the distributive property (FOIL method) and remember that i² = -1. For example:
(2 + i) * (1 - 3i) = 2 - 6i + i - 3i² = 2 - 5i - 3(-1) = 5 - 5i
Division:
Dividing complex numbers involves multiplying both the numerator and the denominator by the complex conjugate of the denominator. The complex conjugate of a + bi is a - bi. This eliminates the imaginary part from the denominator.
For example, to divide (3 + 2i) by (1 + i), we would perform the following steps:
(3 + 2i) / (1 + i) = [(3 + 2i) * (1 - i)] / [(1 + i) * (1 - i)]
= (3 - 3i + 2i - 2i²) / (1 - i² )
= (3 - i + 2) / (1 + 1)
= (5 - i) / 2
= 5/2 - i/2 or 2.5 - 0.5i
The Importance of Imaginary Numbers
While the concept of imaginary numbers might seem abstract, they have crucial applications in various fields, including:
-
Electrical Engineering: Imaginary numbers are essential for analyzing alternating current (AC) circuits. Impedance, a measure of opposition to the flow of current, is often represented as a complex number.
-
Quantum Mechanics: Imaginary numbers play a fundamental role in the mathematical formulation of quantum mechanics, where complex numbers describe the wave function of particles.
-
Signal Processing: Imaginary numbers are used extensively in digital signal processing (DSP) to represent and manipulate signals. The Fourier Transform, a cornerstone of signal processing, relies heavily on complex numbers.
-
Fluid Dynamics: Complex analysis and conformal mapping techniques involving complex numbers are invaluable tools for solving problems in fluid dynamics, particularly in areas like aerodynamics and hydrodynamics.
-
Fractals and Chaos Theory: The Mandelbrot set, a famous fractal, is defined using complex numbers, illustrating their significance in the study of complex systems and chaotic behavior.
Beyond the Basics: Higher Powers of i
Exploring the higher powers of i reveals an interesting pattern:
- i¹ = i
- i² = -1
- i³ = i² * i = -i
- i⁴ = i² * i² = (-1) * (-1) = 1
- i⁵ = i⁴ * i = 1 * i = i
- i⁶ = i⁴ * i² = 1 * (-1) = -1
This pattern repeats every four powers. Any higher power of i can be simplified to one of these four values (i, -1, -i, 1).
Conclusion: Embracing the Imaginary World
The square root of negative 4, 2i, is not just a mathematical curiosity. It's a gateway to a broader understanding of numbers and their applications. Imaginary numbers, while seemingly "imaginary," are essential tools in many advanced scientific and engineering fields. They illustrate the power of mathematical abstraction and how seemingly impossible concepts can unlock deeper insights into the world around us. Understanding imaginary numbers is not just about solving equations; it's about expanding our mathematical horizons and appreciating the elegance and power of complex numbers. They are not just abstract mathematical constructs; they are integral components of our understanding of the universe.
Latest Posts
Latest Posts
-
What Are The First 5 Multiples Of 7
Apr 01, 2025
-
What Is 8 10 As A Decimal
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about What Is The Square Root Of Negative 4 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
