5x 2y 10 In Slope Intercept Form
listenit
Apr 04, 2025 · 6 min read
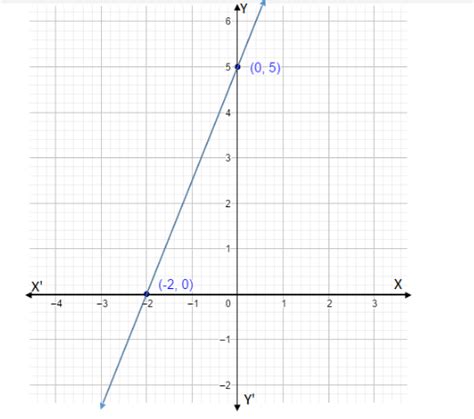
Table of Contents
5x + 2y = 10 in Slope-Intercept Form: A Comprehensive Guide
The equation 5x + 2y = 10 represents a linear relationship between two variables, x and y. While this is presented in standard form, converting it to slope-intercept form (y = mx + b) offers significant advantages in understanding its graphical representation and properties. This comprehensive guide will walk you through the process of converting 5x + 2y = 10 into slope-intercept form, exploring the meaning of the slope (m) and the y-intercept (b), and demonstrating how to graph the equation. We will also delve into practical applications and related concepts to ensure a thorough understanding.
Understanding Slope-Intercept Form (y = mx + b)
Before we begin the conversion, let's review the components of the slope-intercept form:
- y: Represents the dependent variable. Its value depends on the value of x.
- m: Represents the slope of the line. The slope describes the steepness and direction of the line. A positive slope indicates an upward trend from left to right, while a negative slope indicates a downward trend. The slope is calculated as the change in y divided by the change in x (rise over run).
- x: Represents the independent variable. Its value can be chosen freely.
- b: Represents the y-intercept. This is the point where the line crosses the y-axis (where x = 0).
Converting 5x + 2y = 10 to Slope-Intercept Form
The goal is to isolate y on one side of the equation. Here's the step-by-step process:
-
Subtract 5x from both sides: This moves the x term to the right side of the equation.
5x + 2y - 5x = 10 - 5xThis simplifies to:2y = -5x + 10 -
Divide both sides by 2: This isolates y, giving us the slope-intercept form.
2y / 2 = (-5x + 10) / 2This simplifies to:y = -\frac{5}{2}x + 5
Therefore, the equation 5x + 2y = 10 in slope-intercept form is y = -5/2x + 5.
Interpreting the Slope and y-intercept
Now that we have the equation in slope-intercept form, we can readily interpret its components:
-
Slope (m = -5/2): The slope is -5/2. This means that for every 2 units increase in x, y decreases by 5 units. The negative sign indicates a downward trend from left to right. The slope signifies the rate of change of y with respect to x.
-
y-intercept (b = 5): The y-intercept is 5. This means the line intersects the y-axis at the point (0, 5). This is the value of y when x is 0.
Graphing the Equation
Graphing the equation is straightforward using the slope and y-intercept:
-
Plot the y-intercept: Start by plotting the point (0, 5) on the y-axis.
-
Use the slope to find another point: The slope is -5/2. From the y-intercept (0, 5), move 2 units to the right (positive x direction) and 5 units down (negative y direction). This gives you the point (2, 0).
-
Draw the line: Draw a straight line through the two points (0, 5) and (2, 0). This line represents the equation 5x + 2y = 10.
Finding x-intercept
The x-intercept is the point where the line crosses the x-axis (where y = 0). To find it, substitute y = 0 into the original equation or the slope-intercept form:
Using the slope-intercept form:
0 = -5/2x + 5 5/2x = 5 x = 2
Therefore, the x-intercept is (2, 0).
Parallel and Perpendicular Lines
Understanding the slope allows us to determine relationships between lines.
-
Parallel Lines: Parallel lines have the same slope. Any line parallel to y = -5/2x + 5 will also have a slope of -5/2.
-
Perpendicular Lines: Perpendicular lines have slopes that are negative reciprocals of each other. The negative reciprocal of -5/2 is 2/5. Any line perpendicular to y = -5/2x + 5 will have a slope of 2/5.
Real-World Applications
Linear equations like 5x + 2y = 10 have numerous real-world applications:
-
Cost Analysis: Imagine x represents the number of units produced and y represents the total cost. The equation could model a scenario where the fixed cost is 5 (the y-intercept) and the cost per unit is 5/2.
-
Distance-Time Relationships: x could represent time and y could represent distance. The equation might model the distance traveled by an object with a certain speed and initial position.
-
Mixture Problems: x and y could represent the amounts of two different substances being mixed, with the equation representing a constraint on their total volume or concentration.
-
Supply and Demand: In economics, linear equations often model supply and demand curves. The equation could represent a simplified model of the relationship between the price of a good (x) and the quantity demanded (y).
Solving Systems of Equations
The equation 5x + 2y = 10 can be part of a system of equations. Solving a system of equations means finding the values of x and y that satisfy both equations simultaneously. This can be done using various methods like substitution, elimination, or graphical methods. For example, if we have another equation, such as x + y = 3, we can solve for x and y by substitution or elimination.
Further Exploration: Transformations and Variations
Let's consider some variations and transformations of the equation:
-
Changing the y-intercept: If we change the constant term in the equation (the 10), the y-intercept will change, resulting in a parallel line. For example, 5x + 2y = 20 would have a y-intercept of 10.
-
Changing the slope: Altering the coefficients of x and y will change the slope of the line. For example, 10x + 4y = 20 is equivalent to y = -5/2x + 5, showing the same line. However, 5x + 4y = 10 would have a different slope.
-
Non-linear transformations: Applying non-linear functions to the equation will result in a non-linear relationship.
Conclusion
Converting the equation 5x + 2y = 10 into slope-intercept form, y = -5/2x + 5, provides a clearer understanding of its properties. The slope and y-intercept offer valuable insights into the line's characteristics, including its steepness, direction, and the point where it intersects the y-axis. Graphing the equation, understanding parallel and perpendicular lines, and exploring real-world applications all contribute to a more comprehensive understanding of linear relationships. This foundation is crucial for further study in algebra, calculus, and various fields that rely on mathematical modeling. This guide has provided a detailed and comprehensive overview, covering the core concepts and extending to practical applications and further explorations. Mastering this concept builds a strong base for more advanced mathematical concepts.
Latest Posts
Latest Posts
-
How Many Electrons Does Carbon Have Available For Chemical Bonding
Apr 04, 2025
-
What Is It Called When Everyone Plays Together
Apr 04, 2025
-
Y Is Inversely Proportional To X
Apr 04, 2025
-
Is Baking Bread A Chemical Or Physical Change
Apr 04, 2025
-
What Is The Half Life Of Potassium 40
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about 5x 2y 10 In Slope Intercept Form . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
