Y Is Inversely Proportional To X
listenit
Apr 04, 2025 · 6 min read
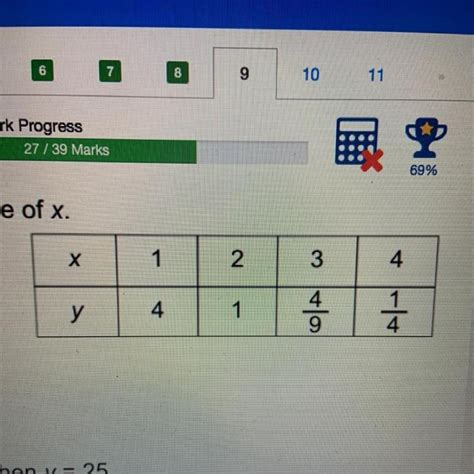
Table of Contents
Y is Inversely Proportional to X: A Comprehensive Guide
Understanding inverse proportionality is crucial in various fields, from physics and engineering to economics and everyday life. This in-depth guide will explore the concept of "y is inversely proportional to x," explaining its meaning, applications, and implications through clear examples and practical scenarios. We'll delve into the mathematical representation, graphical interpretation, and real-world applications of this fundamental relationship.
What Does "Y is Inversely Proportional to X" Mean?
In simple terms, inverse proportionality means that as one variable (x) increases, the other variable (y) decreases proportionally, and vice versa. Their product remains constant. This is different from direct proportionality, where both variables increase or decrease together.
Imagine you're traveling a fixed distance. If you increase your speed (x), the time it takes to travel (y) decreases. Conversely, if you decrease your speed, the travel time increases. This illustrates an inverse relationship. The constant in this case would be the fixed distance.
Mathematically, we represent this relationship as:
y ∝ 1/x
This reads as "y is inversely proportional to x." To make it an equation, we introduce a constant of proportionality, often denoted by 'k':
y = k/x
Where:
- y is the dependent variable.
- x is the independent variable.
- k is the constant of proportionality. This constant represents the fixed product of x and y.
Understanding the Constant of Proportionality (k)
The constant of proportionality, k, is a crucial element in understanding inverse proportionality. It represents the invariant product of x and y. Its value determines the specific relationship between x and y. A larger value of k indicates a stronger inverse relationship – a smaller change in x results in a larger change in y, and vice-versa.
For example, consider two inverse relationships:
- y = 2/x: Here, k = 2.
- y = 10/x: Here, k = 10.
In the second example, for any given x, y will be five times larger than in the first example. This highlights the significant role of k in shaping the relationship. Determining the value of k often involves using initial conditions or known data points.
Graphical Representation of Inverse Proportionality
Inverse proportionality is easily visualized using a graph. The graph of y = k/x is a rectangular hyperbola. The curve approaches but never touches the x and y axes. This is because y cannot be zero if x is finite, and x cannot be zero if y is finite.
Here's what you'll typically observe in an inverse proportionality graph:
- As x increases, y decreases: The curve descends from left to right.
- As x decreases, y increases: The curve ascends from right to left.
- The curve never touches either axis: This signifies the asymptotic nature of the relationship. As x approaches infinity, y approaches zero, and vice versa.
- The product xy remains constant (k): This is a key characteristic visible in the graph's shape.
Understanding the graphical representation helps to quickly visualize and interpret inverse relationships in different contexts.
Real-World Applications of Inverse Proportionality
Inverse proportionality appears in numerous real-world scenarios. Here are some notable examples:
1. Speed and Time:
As mentioned earlier, the relationship between speed and time for a fixed distance is inversely proportional. If you double your speed, the travel time is halved. This principle applies to various situations involving motion.
2. Pressure and Volume (Boyle's Law):
Boyle's Law in physics states that for a fixed amount of gas at a constant temperature, the pressure and volume are inversely proportional. Increasing the pressure on a gas decreases its volume, and vice versa. This is a cornerstone of thermodynamics.
3. Current and Resistance (Ohm's Law):
Ohm's Law demonstrates that for a fixed voltage, the current and resistance in a circuit are inversely proportional. Increasing the resistance decreases the current, and vice versa. This principle is fundamental to electrical engineering and circuit design.
4. Frequency and Wavelength:
In wave mechanics, the frequency and wavelength of a wave are inversely proportional. A higher frequency wave has a shorter wavelength, and vice versa. This is vital in understanding electromagnetic radiation and sound waves.
5. Price and Demand (Economics):
In economics, the price and demand of a product often exhibit an inverse relationship. If the price of a product increases, the demand for it generally decreases, and vice versa (holding other factors constant). This is a simplification, but an important concept in microeconomics.
6. Number of Workers and Time to Complete a Task:
If you need to complete a project, the number of workers and the time to finish it are inversely proportional. More workers means less time, and fewer workers mean more time. This principle applies across many work-related scenarios.
Solving Problems Involving Inverse Proportionality
Many problems involve identifying and solving for unknown variables in inverse proportions. Here's a step-by-step approach:
- Identify the inverse relationship: Determine which variables are inversely proportional.
- Establish the equation: Write the equation in the form y = k/x.
- Find the constant of proportionality (k): Use known values of x and y to solve for k.
- Solve for the unknown: Once k is known, substitute the known value of one variable to solve for the unknown variable.
Example: If y is inversely proportional to x, and y = 6 when x = 2, find the value of y when x = 3.
- Equation: y = k/x
- Find k: 6 = k/2 => k = 12
- Solve for y: y = 12/3 = 4
Therefore, when x = 3, y = 4.
Distinguishing Between Direct and Inverse Proportionality
It's crucial to differentiate between direct and inverse proportionality. The key difference lies in how the variables relate:
- Direct Proportionality: As x increases, y increases proportionally (y = kx). The graph is a straight line passing through the origin.
- Inverse Proportionality: As x increases, y decreases proportionally (y = k/x). The graph is a rectangular hyperbola.
Understanding this distinction is essential for accurately modeling and solving problems involving proportional relationships.
Advanced Concepts and Extensions
The concept of inverse proportionality extends beyond simple relationships. Here are some advanced considerations:
- Joint Inverse Proportionality: A variable might be inversely proportional to the product of several other variables (e.g., y = k/(xz)).
- Combined Variations: A variable could be directly proportional to one variable and inversely proportional to another (e.g., y = kx/z).
- Calculus and Inverse Proportionality: Derivatives and integrals can be applied to analyze the rates of change in inversely proportional relationships.
These advanced concepts require a stronger mathematical foundation but offer powerful tools for understanding complex systems.
Conclusion: Mastering Inverse Proportionality
Understanding inverse proportionality is a critical skill in various fields. By grasping its mathematical representation, graphical interpretation, and real-world applications, you can effectively model and solve problems involving this fundamental relationship. This guide provides a comprehensive overview, from the basic concepts to more advanced applications, equipping you with the knowledge to confidently tackle various scenarios involving inverse proportions. Remember to always carefully identify the relationship between variables and correctly apply the principles outlined here to achieve accurate results.
Latest Posts
Latest Posts
-
What Is Five Divided By Zero
Apr 05, 2025
-
Convert 75 Degrees Fahrenheit To Celsius
Apr 05, 2025
-
3 Gallons Equals How Many Pints
Apr 05, 2025
-
What Is 2 1 8 As A Decimal
Apr 05, 2025
-
66 As A Fraction In Simplest Form
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about Y Is Inversely Proportional To X . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
