Y Varies Jointly As X And Z
listenit
Apr 01, 2025 · 5 min read
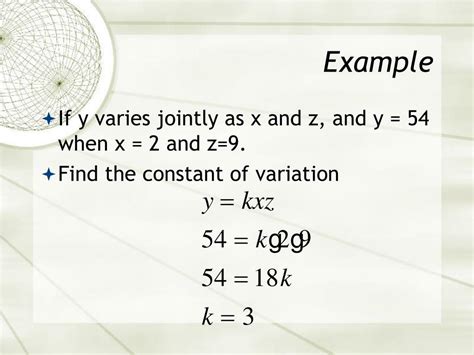
Table of Contents
Y Varies Jointly as X and Z: A Comprehensive Guide
Understanding variations, particularly joint variations, is crucial in various fields, from physics and engineering to economics and statistics. This comprehensive guide delves deep into the concept of "y varies jointly as x and z," explaining its meaning, providing practical examples, tackling potential problems, and offering tips for mastering this concept.
What Does "Y Varies Jointly as X and Z" Mean?
The statement "y varies jointly as x and z" signifies a direct proportional relationship between the variable 'y' and the product of variables 'x' and 'z'. In simpler terms, if x or z increases, y increases proportionally, and vice versa. This relationship can be mathematically represented as:
y = kxz
where 'k' is a constant of proportionality. This constant represents the rate at which y changes in relation to the product of x and z. Its value remains consistent throughout the problem. Finding the value of 'k' is often the key to solving joint variation problems.
Understanding the Constant of Proportionality (k)
The constant 'k' plays a vital role in establishing the precise relationship between y, x, and z. It acts as a scaling factor. A larger value of 'k' indicates a steeper increase in y for given increases in x and z. Conversely, a smaller 'k' implies a gentler increase. Determining the value of 'k' usually requires knowing the values of y, x, and z in a specific instance. Once 'k' is known, the equation becomes a powerful tool for predicting the value of y for any given combination of x and z.
How to Solve Problems Involving Joint Variation
Solving problems involving joint variation typically involves these steps:
-
Identify the variables: Clearly identify which variables are involved – the dependent variable (y) and the independent variables (x and z).
-
Establish the equation: Write the general equation for joint variation: y = kxz.
-
Find the constant of proportionality (k): Use a given set of values for y, x, and z to solve for 'k'. Substitute the known values into the equation and solve algebraically for 'k'.
-
Write the specific equation: Substitute the value of 'k' back into the general equation (y = kxz) to obtain the specific equation for the given problem. This equation now accurately represents the relationship between the variables for this specific scenario.
-
Solve for the unknown: Use the specific equation to find the value of the unknown variable, given the values of the other variables.
Examples to Illustrate Joint Variation
Let's explore some practical examples to solidify your understanding.
Example 1: Simple Joint Variation
The volume (V) of a cylinder varies jointly as the square of its radius (r) and its height (h). If V = 100π cubic units when r = 5 units and h = 4 units, find the volume when r = 6 units and h = 8 units.
-
Variables: V (dependent), r and h (independent).
-
Equation: V = kr²h
-
Find k: 100π = k(5²)(4) => k = π/1
-
Specific Equation: V = πr²h
-
Solve for unknown: V = π(6²)(8) = 288π cubic units
Example 2: More Complex Scenario
The cost (C) of building a house varies jointly as the number of rooms (n) and the square footage (s). If it costs $200,000 to build a 5-room house with 2000 square feet, how much will it cost to build a 4-room house with 2500 square feet?
-
Variables: C (dependent), n and s (independent).
-
Equation: C = kns
-
Find k: 200000 = k(5)(2000) => k = 20
-
Specific Equation: C = 20ns
-
Solve for unknown: C = 20(4)(2500) = $200,000
Example 3: Real-World Application - Force, Mass, and Acceleration
Newton's second law of motion states that the force (F) acting on an object is jointly proportional to its mass (m) and its acceleration (a). If a force of 10 Newtons causes a 2kg mass to accelerate at 5 m/s², what force is required to accelerate a 3kg mass at 7 m/s²?
-
Variables: F (dependent), m and a (independent).
-
Equation: F = kma
-
Find k: 10 = k(2)(5) => k = 1
-
Specific Equation: F = ma
-
Solve for unknown: F = (3)(7) = 21 Newtons
Troubleshooting Common Mistakes
Many students struggle with joint variation problems. Here are some common mistakes to avoid:
-
Confusing direct and inverse variation: Joint variation is a type of direct variation. Don't confuse it with inverse variation, where y decreases as x increases.
-
Incorrectly calculating 'k': Always double-check your calculations when solving for the constant of proportionality. A slight error here will drastically affect the final answer.
-
Forgetting to use the specific equation: Once 'k' is found, remember to substitute it back into the general equation to get the specific equation for the given problem. Using the general equation with an incorrect 'k' will yield the wrong results.
-
Misinterpreting the problem statement: Carefully read the problem statement to correctly identify the dependent and independent variables and the relationships between them.
Advanced Concepts and Applications
Joint variation forms the foundation for understanding many more complex relationships. It's crucial in:
-
Physics: Many physical laws, like Newton's Law of Gravitation and Coulomb's Law, involve joint variation.
-
Engineering: Designing structures and calculating stresses and strains often requires understanding joint variations.
-
Economics: Analyzing factors affecting production costs or consumer demand can involve joint variations.
-
Statistics: Joint variation plays a role in statistical models that investigate the relationships between multiple variables.
Tips for Mastering Joint Variation
-
Practice regularly: Solving many problems is the best way to master joint variation.
-
Visualize the relationship: Use graphs or diagrams to visualize how changes in x and z affect y.
-
Break down complex problems: Divide complex problems into smaller, manageable steps.
-
Check your answers: Always verify your solutions to ensure accuracy.
Conclusion
Understanding joint variation is a fundamental skill in various disciplines. By carefully following the steps outlined, mastering the concept of the constant of proportionality, and practicing consistently, you can confidently tackle any joint variation problem. Remember to approach each problem systematically, paying close attention to detail and ensuring your calculations are accurate. With diligent effort, joint variation will become a manageable and even enjoyable mathematical concept.
Latest Posts
Latest Posts
-
How Many Grams In 8 Kilograms
Apr 02, 2025
-
12x 4y 20 Solve For Y
Apr 02, 2025
-
Most Reactive Group On The Periodic Table
Apr 02, 2025
-
How To Solve Multi Step Inequalities
Apr 02, 2025
-
Percent Composition Of Mg No3 2
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Y Varies Jointly As X And Z . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
