What Percentage Is 27 Out Of 35
listenit
May 24, 2025 · 5 min read
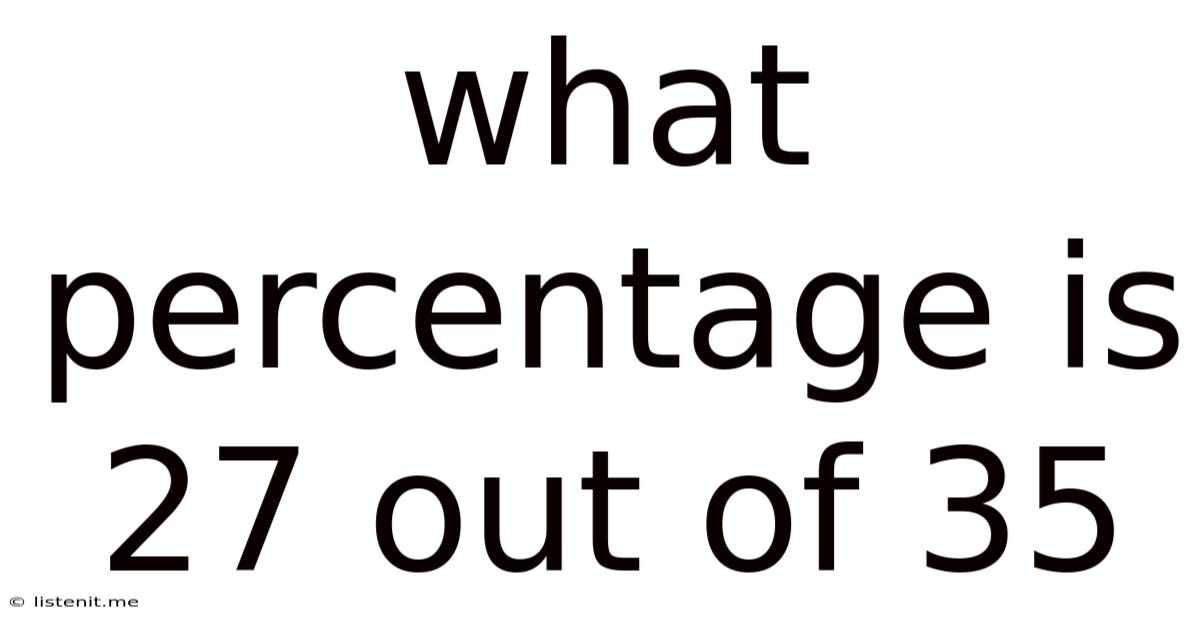
Table of Contents
What Percentage is 27 out of 35? A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill applicable across numerous fields, from academic studies and financial analysis to everyday life scenarios. Understanding how to determine what percentage 27 out of 35 represents is not only valuable in itself but also serves as a stepping stone to mastering more complex percentage problems. This comprehensive guide will explore this specific calculation, delve into the underlying principles, and provide you with the tools to tackle similar percentage problems with confidence.
Understanding Percentages: The Basics
Before diving into the calculation of 27 out of 35 as a percentage, let's solidify our understanding of percentages themselves. A percentage is simply a fraction expressed as a portion of 100. The symbol "%" represents "per hundred," indicating the ratio of a part to a whole expressed as a hundredth.
For instance, 50% means 50 out of 100, or 50/100, which simplifies to 1/2. This illustrates the inherent connection between percentages, fractions, and decimals. They are all different ways of expressing the same proportional relationship.
Calculating the Percentage: 27 out of 35
Now, let's address the core question: what percentage is 27 out of 35? The process involves three simple steps:
Step 1: Set up the Fraction:
The first step is to express the given numbers as a fraction. In this case, we have 27 as the part and 35 as the whole. This translates to the fraction 27/35.
Step 2: Convert the Fraction to a Decimal:
To convert a fraction to a decimal, we simply divide the numerator (the top number) by the denominator (the bottom number). Using a calculator or performing long division, we get:
27 ÷ 35 ≈ 0.7714
Step 3: Convert the Decimal to a Percentage:
Finally, to convert the decimal to a percentage, multiply the decimal by 100 and add the percent symbol (%).
0.7714 × 100 ≈ 77.14%
Therefore, 27 out of 35 is approximately 77.14%.
Different Methods for Calculating Percentages
While the method described above is the most straightforward, there are other approaches you can use to calculate percentages. Understanding these alternative methods can enhance your problem-solving skills and allow you to choose the most efficient method depending on the specific problem.
Method 2: Using Proportions
Proportions offer another elegant way to solve percentage problems. We can set up a proportion as follows:
27/35 = x/100
Here, 'x' represents the unknown percentage we're trying to find. To solve for 'x', we cross-multiply:
35x = 2700
Then, divide both sides by 35:
x = 2700 ÷ 35 ≈ 77.14
Again, we arrive at the same answer: approximately 77.14%.
Method 3: Using a Calculator with Percentage Function
Most calculators have a dedicated percentage function. Simply enter 27, press the division symbol (/), enter 35, and then press the percentage (%) button. The calculator will directly output the percentage, saving you the manual conversion steps.
Applying Percentage Calculations in Real-World Scenarios
The ability to calculate percentages is incredibly valuable in numerous real-world contexts. Here are a few examples:
-
Academic Performance: Calculating your grade in a course often involves determining the percentage of correct answers on a test or the weighted average of assignments.
-
Financial Management: Understanding percentages is crucial for calculating interest rates, discounts, taxes, tips, and investment returns.
-
Data Analysis: Percentages are frequently used to represent proportions within datasets, making it easier to visualize and interpret data.
-
Sales and Marketing: Sales professionals use percentages to track conversion rates, calculate discounts, and analyze sales performance.
-
Everyday Life: Calculating discounts at a store, determining the tip at a restaurant, or understanding the nutritional information on food packaging all involve percentage calculations.
Beyond the Basics: More Complex Percentage Problems
While the 27 out of 35 example is relatively straightforward, understanding percentages extends to more complex scenarios. These might include:
-
Calculating percentage increase or decrease: This involves determining the percentage change between two values. For instance, if a price increases from $50 to $60, the percentage increase is calculated as ((60-50)/50) * 100 = 20%.
-
Finding the original value after a percentage change: If a product is discounted by 20% and now costs $80, the original price can be calculated using reverse percentage calculations.
-
Solving problems involving multiple percentages: Situations where multiple percentage changes occur sequentially require careful consideration of the order of operations and may necessitate applying the percentages iteratively.
Mastering Percentage Calculations: Tips and Practice
To truly master percentage calculations, consistent practice is key. Here are some tips to enhance your understanding and proficiency:
-
Start with simple examples: Begin by practicing with easy calculations before moving on to more complex problems.
-
Use different methods: Experiment with various calculation methods to find the approach that works best for you.
-
Check your work: Always verify your answers to ensure accuracy.
-
Solve real-world problems: Apply your knowledge to real-life scenarios to reinforce your understanding and see the practical value of percentage calculations.
-
Utilize online resources: Numerous online resources, including interactive exercises and tutorials, can provide additional practice and support.
Conclusion: The Importance of Percentage Understanding
Understanding percentages is a vital skill with widespread applications across various aspects of life. The ability to confidently calculate and interpret percentages is not merely a mathematical skill but a practical tool that empowers informed decision-making in both personal and professional contexts. By mastering this fundamental concept, you equip yourself with a valuable tool for navigating the numerical world around you, from analyzing data to making financial decisions. The seemingly simple question, "What percentage is 27 out of 35?" serves as a gateway to a deeper appreciation of the significance and versatility of percentage calculations. Continue practicing, explore diverse applications, and watch your proficiency grow.
Latest Posts
Latest Posts
-
24 Is What Percent Of 27
May 24, 2025
-
What Percent Is 3 Out Of 16
May 24, 2025
-
What Is The Greatest Common Factor Of 18 And 9
May 24, 2025
-
3 To The Second Power Equals
May 24, 2025
-
How Many 3x6 Tiles In A Square Foot
May 24, 2025
Related Post
Thank you for visiting our website which covers about What Percentage Is 27 Out Of 35 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.