24 Is What Percent Of 27
listenit
May 24, 2025 · 4 min read
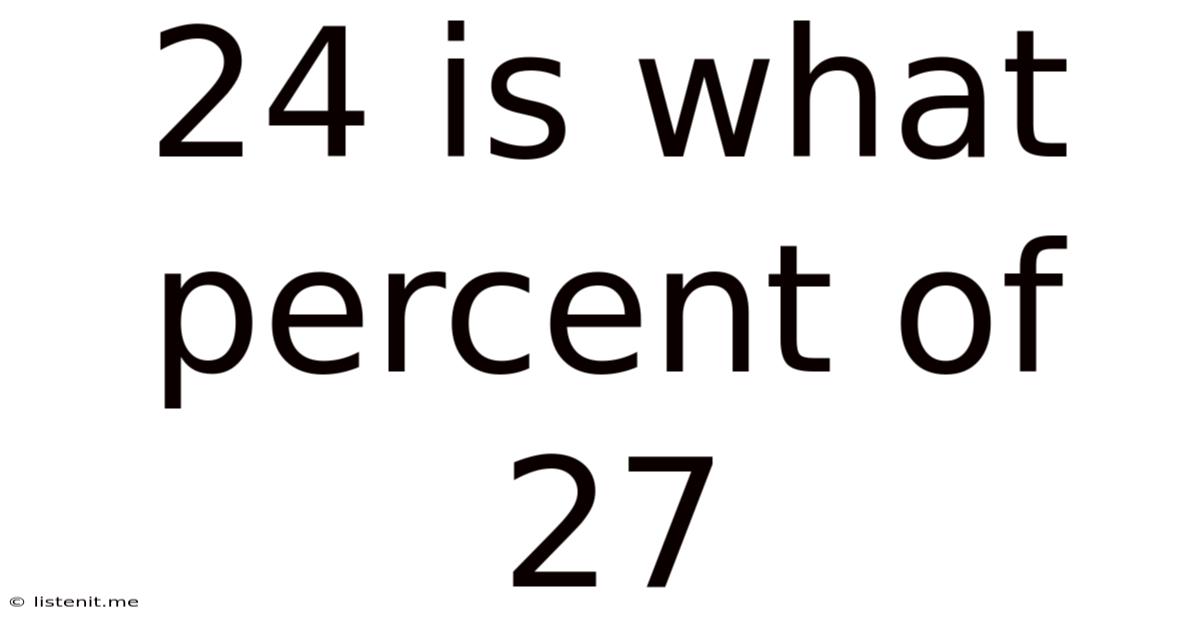
Table of Contents
24 is What Percent of 27? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill with widespread applications in everyday life, from calculating discounts and taxes to analyzing data and understanding statistics. This article will delve deep into the question, "24 is what percent of 27?", providing a step-by-step solution, exploring different calculation methods, and offering practical examples to solidify your understanding of percentage calculations. We'll also explore the broader context of percentages and their importance in various fields.
Understanding Percentages: The Basics
A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" is derived from the Latin words "per centum," meaning "out of a hundred." Percentages are a convenient way to represent proportions and make comparisons. For instance, saying "25%" is equivalent to saying "25 out of 100," or the fraction 25/100, which simplifies to 1/4.
Calculating "24 is What Percent of 27?"
To determine what percentage 24 represents of 27, we can employ several methods. Let's explore the most common approaches:
Method 1: Using the Formula
The fundamental formula for calculating percentages is:
(Part / Whole) * 100% = Percentage
In our case:
- Part: 24
- Whole: 27
Substituting these values into the formula:
(24 / 27) * 100% = Percentage
Calculating the fraction:
24 / 27 ≈ 0.8889
Multiplying by 100%:
0.8889 * 100% ≈ 88.89%
Therefore, 24 is approximately 88.89% of 27.
Method 2: Using Proportions
We can also solve this problem using proportions. We can set up a proportion where 'x' represents the percentage we're looking for:
24/27 = x/100
To solve for 'x', we cross-multiply:
27x = 2400
Now, divide both sides by 27:
x = 2400 / 27 ≈ 88.89
Therefore, x ≈ 88.89%, confirming our previous result.
Method 3: Using a Calculator
Most calculators have a percentage function. Simply enter "24 ÷ 27 * 100" and the calculator will directly provide the percentage, which is approximately 88.89%.
Rounding and Precision
In the above calculations, we've rounded the percentage to two decimal places (88.89%). The level of precision required depends on the context. In some situations, rounding to the nearest whole number (89%) might be sufficient, while in others, more decimal places may be necessary for accuracy.
Real-World Applications of Percentage Calculations
Understanding percentage calculations is crucial in numerous real-world scenarios. Here are some examples:
1. Finance and Budgeting:
- Interest rates: Calculating interest earned on savings accounts or interest paid on loans.
- Discounts and sales tax: Determining the final price of an item after applying discounts and sales tax.
- Investment returns: Measuring the performance of investments over time.
- Budgeting: Allocating funds for different expenses based on percentages of income.
2. Data Analysis and Statistics:
- Representing proportions: Showing the proportion of a specific category within a larger dataset.
- Analyzing trends: Identifying trends and patterns in data by representing changes as percentages.
- Calculating probabilities: Expressing the likelihood of an event occurring as a percentage.
3. Everyday Life:
- Tips and gratuities: Calculating the appropriate tip amount at restaurants.
- Comparing prices: Determining which product offers better value for money.
- Understanding nutritional information: Interpreting the percentage of daily recommended values on food labels.
Expanding Your Understanding: Further Percentage Concepts
Beyond basic percentage calculations, several related concepts enhance your quantitative skills:
Percentage Increase and Decrease:
These calculations determine the percentage change between two values. The formula for percentage increase is:
[(New Value - Old Value) / Old Value] * 100%
Similarly, the formula for percentage decrease is:
[(Old Value - New Value) / Old Value] * 100%
For example, if a product's price increases from $20 to $25, the percentage increase is:
[(25 - 20) / 20] * 100% = 25%
Percentage Points:
Percentage points represent the arithmetic difference between two percentages. For example, if the unemployment rate increases from 5% to 8%, it has increased by 3 percentage points, not 3%. This distinction is crucial for accurate interpretation of data.
Compound Interest:
Compound interest is the interest earned on both the principal amount and accumulated interest. Understanding compound interest is essential for long-term financial planning.
Conclusion: Mastering Percentages for Success
Understanding percentage calculations is an invaluable skill applicable to various aspects of life. From managing personal finances to interpreting data effectively, mastering percentages empowers you to make informed decisions and navigate the quantitative world with confidence. This article provided a detailed explanation of how to calculate "24 is what percent of 27," offering various methods and real-world examples to solidify your understanding. Remember to practice regularly to sharpen your skills and become proficient in percentage calculations. By incorporating these concepts into your daily life and professional endeavors, you’ll find that your quantitative literacy significantly improves, ultimately leading to better outcomes in all areas. The ability to confidently calculate and interpret percentages is a key skill for success in the modern world.
Latest Posts
Latest Posts
-
What Is The Greatest Common Factor Of 85 And 51
May 25, 2025
-
What Is 8 Weeks Ago From Today
May 25, 2025
-
How To Do The Love Calculator On Paper
May 25, 2025
-
What Is The Greatest Common Factor Of 4 And 16
May 25, 2025
-
Write The Prime Factorization Of 6
May 25, 2025
Related Post
Thank you for visiting our website which covers about 24 Is What Percent Of 27 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.