What Percent Of 200 Is 5
listenit
Mar 28, 2025 · 4 min read
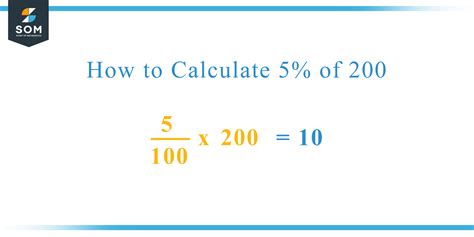
Table of Contents
What Percent of 200 is 5? A Deep Dive into Percentage Calculations
Finding what percentage a number represents of another is a fundamental concept in mathematics with widespread applications in various fields, from finance and statistics to everyday life. This article will delve into the question, "What percent of 200 is 5?" We will not only provide the answer but also explore the underlying principles, different calculation methods, and practical examples to solidify your understanding of percentage calculations.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The word "percent" literally means "per hundred" or "out of one hundred." Therefore, a percentage represents a proportion or ratio out of a whole, expressed as a value between 0 and 100.
For example, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5. Understanding this fundamental concept is crucial for solving percentage problems.
Calculating the Percentage: Method 1 – The Formula Approach
The most straightforward way to determine what percentage 5 is of 200 is using the basic percentage formula:
(Part / Whole) * 100 = Percentage
In our case:
- Part: 5 (the number we want to express as a percentage)
- Whole: 200 (the total number)
Substituting these values into the formula:
(5 / 200) * 100 = 2.5%
Therefore, 5 is 2.5% of 200.
Calculating the Percentage: Method 2 – Proportion Method
Another method involves setting up a proportion. We can represent the problem as follows:
5/200 = x/100
Here, 'x' represents the percentage we need to find. To solve for 'x', we cross-multiply:
5 * 100 = 200 * x
500 = 200x
x = 500 / 200
x = 2.5
Thus, 5 is 2.5% of 200. This method clearly demonstrates the relationship between the part, the whole, and the percentage.
Real-World Applications of Percentage Calculations
Understanding percentage calculations has practical implications across numerous domains:
1. Finance and Investments:
- Interest Rates: Banks and financial institutions use percentages to calculate interest on loans and savings accounts. For example, a 5% interest rate on a $1000 loan means you'll pay $50 in interest annually.
- Investment Returns: Investors track their returns on investments using percentages. A 10% return on a $5000 investment signifies a profit of $500.
- Discounts and Sales Tax: Retailers use percentages to offer discounts and calculate sales taxes. A 20% discount on a $50 item reduces the price to $40.
2. Statistics and Data Analysis:
- Data Representation: Percentages are frequently used to represent data in charts, graphs, and reports. They provide a clear and concise way to compare different proportions.
- Probability and Chance: Percentages are crucial in expressing probabilities and chances of events occurring. For example, a 75% chance of rain indicates a high probability of precipitation.
- Statistical Surveys: Survey results are often presented using percentages to summarize responses from a sample population.
3. Everyday Life:
- Tips and Gratuities: Calculating tips in restaurants commonly involves using percentages (e.g., 15% or 20% of the bill).
- Cooking and Baking: Recipes frequently use percentages to represent the proportions of different ingredients.
- Shopping and Budgeting: Understanding percentages helps consumers compare prices, track spending, and manage their budgets effectively.
Further Exploration of Percentage Problems
While the problem "What percent of 200 is 5?" provides a straightforward introduction to percentage calculations, more complex problems can involve finding the whole, the part, or the percentage itself, given two other values. Let's look at these variations:
Finding the Part (Given Percentage and Whole):
If you know the percentage and the whole, you can find the part using this formula:
(Percentage / 100) * Whole = Part
For example, what is 15% of 300?
(15 / 100) * 300 = 45
Therefore, 15% of 300 is 45.
Finding the Whole (Given Percentage and Part):
If you know the percentage and the part, you can find the whole using this formula:
(Part / Percentage) * 100 = Whole
For example, if 20% of a number is 10, what is the number?
(10 / 20) * 100 = 50
Therefore, the number is 50.
Advanced Percentage Concepts
As your understanding of percentages grows, you may encounter more sophisticated concepts such as:
- Compound Interest: This involves earning interest on both the principal amount and accumulated interest over time.
- Percentage Change: This measures the relative change between two values, expressed as a percentage. It is calculated using the formula:
[(New Value - Old Value) / Old Value] * 100. - Percentage Points: This represents the absolute difference between two percentages. For example, an increase from 10% to 15% is a 5 percentage point increase, not a 50% increase.
Conclusion
Understanding percentages is a critical skill applicable to numerous aspects of life. This article has demonstrated how to calculate percentages, explored various methods, and highlighted the significance of percentage calculations in diverse fields. By mastering these techniques, you can confidently tackle percentage problems, analyze data effectively, and make informed decisions in various situations. Remember to practice regularly, and you'll quickly become proficient in working with percentages. From simple calculations like finding what percent of 200 is 5 to more complex scenarios, the foundational understanding presented here will serve as a robust base for your continued learning and application of this essential mathematical concept.
Latest Posts
Latest Posts
-
In Which Layer Does Weather Occur
Mar 31, 2025
-
What Is The Opposite Of 9
Mar 31, 2025
-
How Many Neutrons Does Carbon 13 Have
Mar 31, 2025
-
How Do You Find The Mass Of A Cube
Mar 31, 2025
-
What Color Of Light Has The Highest Energy
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about What Percent Of 200 Is 5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
