What Percent Is 1 Out Of 20
listenit
Mar 31, 2025 · 4 min read
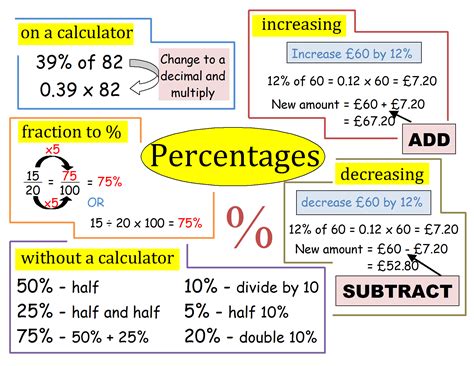
Table of Contents
What Percent is 1 out of 20? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous aspects of life, from calculating discounts and taxes to comprehending statistical data and analyzing financial reports. This comprehensive guide delves into the question, "What percent is 1 out of 20?", providing not just the answer but a detailed explanation of the underlying principles and various methods for solving similar percentage problems. We'll explore different approaches, offering practical examples and tips to solidify your understanding.
Understanding Percentages: The Basics
A percentage represents a fraction of 100. It indicates a proportion relative to a whole, often expressed using the "%" symbol. For instance, 50% means 50 out of 100, or one-half. Understanding this fundamental concept is crucial for accurately calculating percentages.
The core formula for calculating percentages is:
(Part / Whole) * 100 = Percentage
Where:
- Part: Represents the specific portion you're interested in.
- Whole: Represents the total amount or the complete quantity.
- Percentage: The resulting value expressed as a percentage.
Calculating "What Percent is 1 out of 20?"
Let's apply this formula to answer our core question: "What percent is 1 out of 20?"
In this case:
- Part = 1
- Whole = 20
Substituting these values into our formula:
(1 / 20) * 100 = 5%
Therefore, 1 out of 20 is 5%.
Alternative Methods for Percentage Calculation
While the basic formula is straightforward, several alternative methods can help you calculate percentages efficiently, particularly when dealing with more complex scenarios.
Method 1: Using Decimals
This method involves converting the fraction to a decimal and then multiplying by 100.
- Convert the fraction to a decimal: 1 / 20 = 0.05
- Multiply the decimal by 100: 0.05 * 100 = 5%
Method 2: Using Proportions
This method utilizes the concept of ratios and proportions. We can set up a proportion to solve for the unknown percentage:
1/20 = x/100
Cross-multiplying, we get:
20x = 100
Solving for x:
x = 100 / 20 = 5%
Method 3: Using a Calculator
Modern calculators simplify percentage calculations. Simply input the fraction (1/20) and multiply by 100 to get the percentage directly.
Practical Applications of Percentage Calculations
Understanding percentage calculations isn't just about solving mathematical problems; it's a crucial skill applicable in numerous real-world scenarios. Here are a few examples:
-
Discounts: If a store offers a 20% discount on an item priced at $100, the discount amount is (20/100) * $100 = $20. The final price would be $100 - $20 = $80.
-
Taxes: Calculating sales tax involves determining the percentage of the price. For example, a 6% sales tax on a $50 item is (6/100) * $50 = $3.
-
Grade Calculations: Understanding percentages is essential for interpreting grades in school. If you scored 18 out of 20 on a test, your percentage score is (18/20) * 100 = 90%.
-
Financial Analysis: Percentages are used extensively in financial reports to express growth rates, profit margins, and investment returns.
-
Data Analysis: Percentage changes are frequently employed to represent variations in data sets, such as population growth, economic indicators, and market trends.
Solving More Complex Percentage Problems
The principles discussed above can be extended to solve more complex percentage problems. Let's look at a few examples:
Example 1: What percent is 7 out of 35?
Using the formula: (7/35) * 100 = 20%
Example 2: 15% of what number is 9?
Let the unknown number be 'x'. We can set up the equation:
(15/100) * x = 9
Solving for x:
x = (9 * 100) / 15 = 60
Therefore, 15% of 60 is 9.
Example 3: What is 25% more than 80?
First, calculate 25% of 80: (25/100) * 80 = 20
Then, add this amount to the original number: 80 + 20 = 100
Therefore, 25% more than 80 is 100.
Tips for Mastering Percentage Calculations
-
Practice regularly: The key to mastering any skill is consistent practice. Solve various percentage problems to build your proficiency.
-
Understand the concepts: Don't just memorize formulas; understand the underlying logic and principles of percentages.
-
Use multiple methods: Employ different methods (decimals, proportions, calculators) to solve problems and verify your answers.
-
Break down complex problems: Decompose complex problems into smaller, manageable steps.
-
Check your work: Always verify your answers to ensure accuracy.
Conclusion: Percentages in Everyday Life
Understanding percentages is an indispensable life skill. From everyday shopping to complex financial analyses, the ability to calculate and interpret percentages empowers you to make informed decisions and navigate numerical information effectively. By mastering the fundamental principles and practicing regularly, you can confidently tackle any percentage problem you encounter. Remember the core formula, experiment with different methods, and always strive for accuracy. The ability to work fluently with percentages opens doors to a deeper comprehension of the numerical world around us.
Latest Posts
Latest Posts
-
How Many Square Yards In A Square Mile
Apr 01, 2025
-
Ca Oh 2 Strong Or Weak
Apr 01, 2025
-
What Are Three Parts Of Atp Molecule
Apr 01, 2025
-
How Many Right Angles Does A Quadrilateral Have
Apr 01, 2025
-
Is Magnesium A Gas Liquid Or Solid
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 1 Out Of 20 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
