What Is The Reciprocal Of 1.25
listenit
Apr 01, 2025 · 5 min read
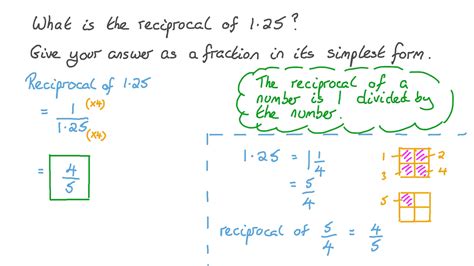
Table of Contents
What is the Reciprocal of 1.25? A Deep Dive into Reciprocals and Their Applications
The question, "What is the reciprocal of 1.25?" might seem deceptively simple. However, understanding reciprocals goes beyond a simple calculation; it unlocks a deeper understanding of mathematical relationships and finds practical applications in various fields. This comprehensive guide will not only answer this question but also explore the concept of reciprocals, their properties, and their significance in mathematics and beyond.
Understanding Reciprocals: The Inverse Relationship
A reciprocal, also known as a multiplicative inverse, is a number that, when multiplied by the original number, results in 1. In simpler terms, it's the number you need to multiply a given number by to get the multiplicative identity, which is 1.
Finding the Reciprocal: To find the reciprocal of any number (except 0 – the reciprocal of 0 is undefined), you simply divide 1 by that number.
Therefore, the reciprocal of 1.25 is calculated as:
1 ÷ 1.25 = 0.8
So, the answer to our initial question is: The reciprocal of 1.25 is 0.8.
Beyond the Calculation: Exploring the Properties of Reciprocals
The concept of reciprocals extends beyond simple calculations and possesses several crucial properties:
-
Multiplicative Inverse: As mentioned earlier, the defining property is its multiplicative inverse nature. Any number multiplied by its reciprocal always equals 1 (excluding 0).
-
Commutative Property: The order in which you multiply a number and its reciprocal doesn't affect the result. For example, 1.25 × 0.8 = 0.8 × 1.25 = 1.
-
Reciprocal of a Reciprocal: The reciprocal of a reciprocal is the original number. The reciprocal of 0.8 is 1.25.
-
Reciprocal of Fractions: Finding the reciprocal of a fraction involves simply swapping the numerator and the denominator. For instance, the reciprocal of 2/3 is 3/2.
-
Reciprocal of Decimal Numbers: As demonstrated with 1.25, finding the reciprocal of a decimal involves dividing 1 by the decimal number. This often requires converting the decimal to a fraction for easier calculation.
Practical Applications of Reciprocals: Where Reciprocals Matter
Reciprocals aren't just abstract mathematical concepts; they have significant practical applications in several areas:
1. Physics and Engineering:
-
Calculating Resistance in Parallel Circuits: In electrical engineering, when resistors are connected in parallel, the reciprocal of the total resistance is equal to the sum of the reciprocals of the individual resistances. This calculation is crucial for circuit design and analysis.
-
Lens Optics: In optics, the reciprocal of the focal length of a lens is directly related to its power. Understanding reciprocals is vital for calculating lens power and image formation.
-
Wave Phenomena: The frequency (f) and the period (T) of a wave are reciprocals of each other (f = 1/T). This relationship is fundamental in understanding wave behavior in various fields like acoustics and electromagnetism.
2. Finance and Economics:
-
Compound Interest Calculations: While not directly using reciprocals, calculating compound interest often involves exponents and reciprocals are implicitly present in the formulas due to the inverse relationship between interest rates and the time it takes to double an investment.
-
Return on Investment (ROI): Although not directly expressed as a reciprocal, understanding the inverse relationship between investment and return is crucial for financial analysis. Higher investment may result in higher returns, representing a form of an inverse relationship, although not a true reciprocal.
3. Computer Science:
-
Floating-Point Arithmetic: Computers use floating-point arithmetic to represent real numbers. Understanding reciprocals is essential for efficient implementation of arithmetic operations in computer systems.
-
Matrix Inversions: In linear algebra, the inverse of a matrix (if it exists) is its reciprocal, where multiplication results in an identity matrix. Matrix inversions are crucial in many computational algorithms and are used extensively in computer graphics and simulations.
4. Everyday Applications:
-
Recipe Scaling: If you need to halve or double a recipe, you are essentially using reciprocals to adjust ingredient quantities. To half a recipe, you multiply each ingredient amount by 1/2 (the reciprocal of 2).
-
Rate and Time: If a machine produces 5 units per hour (a rate), the reciprocal 1/5 represents the time in hours it takes to produce 1 unit. This relationship simplifies many rate-related problems.
Expanding the Concept: Reciprocals and Negative Numbers
The concept of reciprocals also extends to negative numbers. The reciprocal of a negative number is also negative. For example, the reciprocal of -2 is -1/2 or -0.5. The key property of a multiplicative inverse remains – when multiplied by the original number, the result is always 1.
Handling Reciprocals with Different Number Systems
Understanding reciprocals is important across different number systems:
-
Integers: The reciprocal of an integer is a fraction (unless it's ±1).
-
Fractions: The reciprocal is simply the fraction with the numerator and denominator swapped.
-
Decimals: Convert the decimal to a fraction to easily find its reciprocal, then simplify if needed.
Addressing Potential Pitfalls: The Case of Zero
One crucial point to remember is that zero does not have a reciprocal. There's no number that, when multiplied by zero, will result in 1. This is because any number multiplied by zero always equals zero. This is why the reciprocal of 0 is undefined.
Advanced Concepts: Reciprocals and Complex Numbers
For those interested in more advanced mathematics, the concept of reciprocals extends to complex numbers. A complex number is a number of the form a + bi, where 'a' and 'b' are real numbers and 'i' is the imaginary unit (√-1). The reciprocal of a complex number is calculated by dividing the conjugate of the complex number by the square of its magnitude.
Conclusion: The Importance of Understanding Reciprocals
The seemingly simple question, "What is the reciprocal of 1.25?" opens the door to a rich exploration of a fundamental mathematical concept with far-reaching applications. Understanding reciprocals is not just about performing calculations; it is about comprehending inverse relationships, analyzing mathematical structures, and solving practical problems in diverse fields. From engineering to finance, from computer science to everyday life, the concept of reciprocals plays a significant role, highlighting its importance in various aspects of our world. The ability to understand and apply the concept of reciprocals is a testament to one's mathematical proficiency and a valuable skill in various scientific, technological, and practical endeavors. The more we understand this fundamental concept, the better equipped we are to tackle more complex mathematical challenges and find innovative solutions to real-world problems.
Latest Posts
Latest Posts
-
How Many Atoms Are In O2
Apr 03, 2025
-
What Are The Subunits Of Nucleic Acids
Apr 03, 2025
-
Circumference Of A Circle With Diameter Of 8
Apr 03, 2025
-
What Is The Correct Formula For Aluminum Oxide
Apr 03, 2025
-
How Do Outliers Affect The Mean
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about What Is The Reciprocal Of 1.25 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
