What Is 5 To The Power Of 1
listenit
Apr 05, 2025 · 5 min read
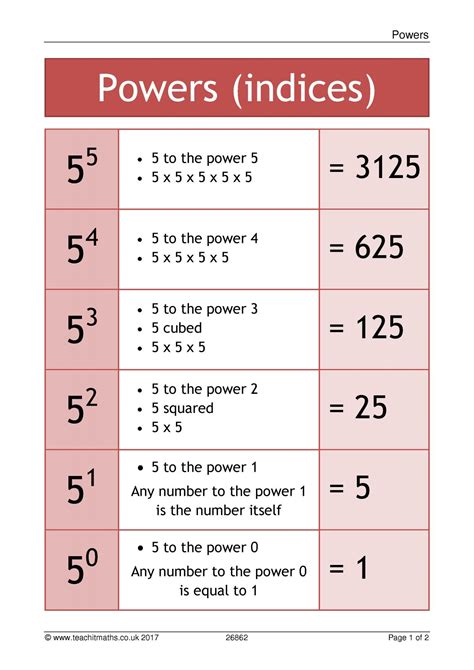
Table of Contents
What is 5 to the Power of 1? A Deep Dive into Exponents and Their Applications
The seemingly simple question, "What is 5 to the power of 1?" opens a door to a vast and fascinating world of mathematics: exponents. While the answer itself is straightforward (5), understanding the underlying principles unlocks a deeper appreciation for exponential functions, their properties, and their widespread applications in various fields. This article will not only answer the question directly but will also explore the broader context of exponents, their rules, and their real-world relevance.
Understanding Exponents: The Basics
Before diving into the specifics of 5 to the power of 1, let's establish a solid foundation in understanding exponents. An exponent, also known as a power or index, represents how many times a number (the base) is multiplied by itself. It's written as a superscript to the right of the base. For example, in the expression 5³, 5 is the base and 3 is the exponent. This means 5 multiplied by itself three times: 5 x 5 x 5 = 125.
Key Terminology:
- Base: The number being multiplied repeatedly.
- Exponent: The number indicating how many times the base is multiplied by itself.
- Power: Another term for exponent.
Solving 5 to the Power of 1
Now, let's address the core question: What is 5 to the power of 1 (5¹)? This expression signifies that the base, 5, is multiplied by itself one time. Therefore, 5¹ = 5. This seemingly trivial calculation highlights a crucial property of exponents: any number raised to the power of 1 is equal to itself.
This fundamental property is crucial for understanding more complex exponential operations and lays the groundwork for many mathematical concepts.
Expanding the Understanding: Exponents Beyond 1
While 5¹ is simple, understanding exponents extends far beyond this basic case. Let's examine different scenarios:
-
Exponents greater than 1: As mentioned earlier, 5² (5 to the power of 2) is 5 x 5 = 25, and 5³ (5 to the power of 3) is 5 x 5 x 5 = 125. The pattern continues for higher exponents.
-
Exponent of 0: Any non-zero number raised to the power of 0 is equal to 1. This might seem counterintuitive, but it's consistent with the patterns established by other exponents. For example, consider the pattern: 5³ = 125, 5² = 25, 5¹ = 5. Notice that each time the exponent decreases by 1, the result is divided by 5. Following this pattern, 5⁰ = 5 / 5 = 1.
-
Negative Exponents: A negative exponent indicates the reciprocal of the base raised to the positive power. For example, 5⁻¹ = 1/5¹, which is 1/5 or 0.2. Similarly, 5⁻² = 1/5² = 1/25 = 0.04.
-
Fractional Exponents: Fractional exponents represent roots. For example, 5^(1/2) is the square root of 5, and 5^(1/3) is the cube root of 5. This demonstrates the connection between exponents and roots, showing the power of a unified mathematical concept.
Rules of Exponents: Mastering the Operations
Manipulating exponential expressions requires understanding the rules governing their operations. These rules are fundamental for simplifying complex calculations and solving equations.
-
Product Rule: When multiplying two expressions with the same base, add the exponents: aᵐ x aⁿ = a⁽ᵐ⁺ⁿ⁾. For example, 5² x 5³ = 5⁽²⁺³⁾ = 5⁵ = 3125.
-
Quotient Rule: When dividing two expressions with the same base, subtract the exponents: aᵐ / aⁿ = a⁽ᵐ⁻ⁿ⁾. For example, 5⁵ / 5² = 5⁽⁵⁻²⁾ = 5³ = 125.
-
Power Rule: When raising an expression with an exponent to another power, multiply the exponents: (aᵐ)ⁿ = a⁽ᵐⁿ⁾. For example, (5²)³ = 5⁽²ˣ³⁾ = 5⁶ = 15625.
-
Power of a Product Rule: The power of a product is the product of the powers: (ab)ᵐ = aᵐbᵐ. For example, (5x)² = 5²x² = 25x².
-
Power of a Quotient Rule: The power of a quotient is the quotient of the powers: (a/b)ᵐ = aᵐ/bᵐ. For example, (5/2)² = 5²/2² = 25/4 = 6.25.
Real-World Applications of Exponents
The seemingly simple concept of exponents has far-reaching implications in numerous fields. Here are some examples:
-
Compound Interest: Understanding exponential growth is crucial for calculating compound interest, a fundamental concept in finance. The formula for compound interest incorporates exponents to model the growth of an investment over time.
-
Population Growth: Exponential functions are used to model population growth in biology and ecology. The exponential growth model helps predict future population sizes based on current growth rates.
-
Radioactive Decay: Exponents play a critical role in describing radioactive decay, a process that follows an exponential decay model. This is essential in fields like nuclear physics and medicine.
-
Computer Science: Exponents are fundamental in computer science, impacting areas like algorithm complexity and data storage. The efficiency of algorithms is often expressed using Big O notation, which involves exponential functions.
-
Physics: Exponents are ubiquitous in physics, appearing in formulas describing various phenomena, such as the inverse square law of gravity and light intensity.
-
Engineering: Exponential functions are used in various engineering disciplines to model and analyze processes such as signal amplification, heat transfer, and fluid dynamics.
Beyond the Basics: Exponential Functions and Their Graphs
The concept of exponents extends beyond simple numerical calculations to encompass exponential functions. An exponential function is a function of the form f(x) = aˣ, where 'a' is a positive constant (the base) and 'x' is the exponent. The graph of an exponential function exhibits characteristic exponential growth or decay depending on the value of the base. When the base is greater than 1, the graph shows exponential growth; when the base is between 0 and 1, the graph shows exponential decay.
Understanding these graphical representations is crucial for visualizing exponential growth and decay processes in real-world scenarios.
Conclusion: The Significance of 5 to the Power of 1
While the answer to "What is 5 to the power of 1?" is simply 5, the journey to reach that answer has unveiled a wealth of information about exponents, their properties, rules, and far-reaching applications. From basic calculations to complex models, exponents form a cornerstone of mathematics and are essential for understanding and modeling numerous phenomena in the natural world and various human endeavors. This deep dive into the seemingly simple question showcases the power of fundamental mathematical concepts and their significant impact on diverse fields. The seemingly simple calculation provides a gateway to a broader understanding of a crucial mathematical concept with significant real-world applications. The power of understanding exponents goes far beyond the simple calculation, highlighting its significance in diverse fields and problem-solving.
Latest Posts
Latest Posts
-
Write 2 1 2 As An Improper Fraction
Apr 06, 2025
-
For Which Of The Mixtures Will Ag2so4 S Precipitate
Apr 06, 2025
-
Is The Square Root Of 16 A Rational Number
Apr 06, 2025
-
Which Rock Type Is Most Likely To Contain Fossils
Apr 06, 2025
-
What Is One Way That Rna Differs From Dna
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about What Is 5 To The Power Of 1 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
