What Is 30 Percent Of 18
listenit
May 24, 2025 · 5 min read
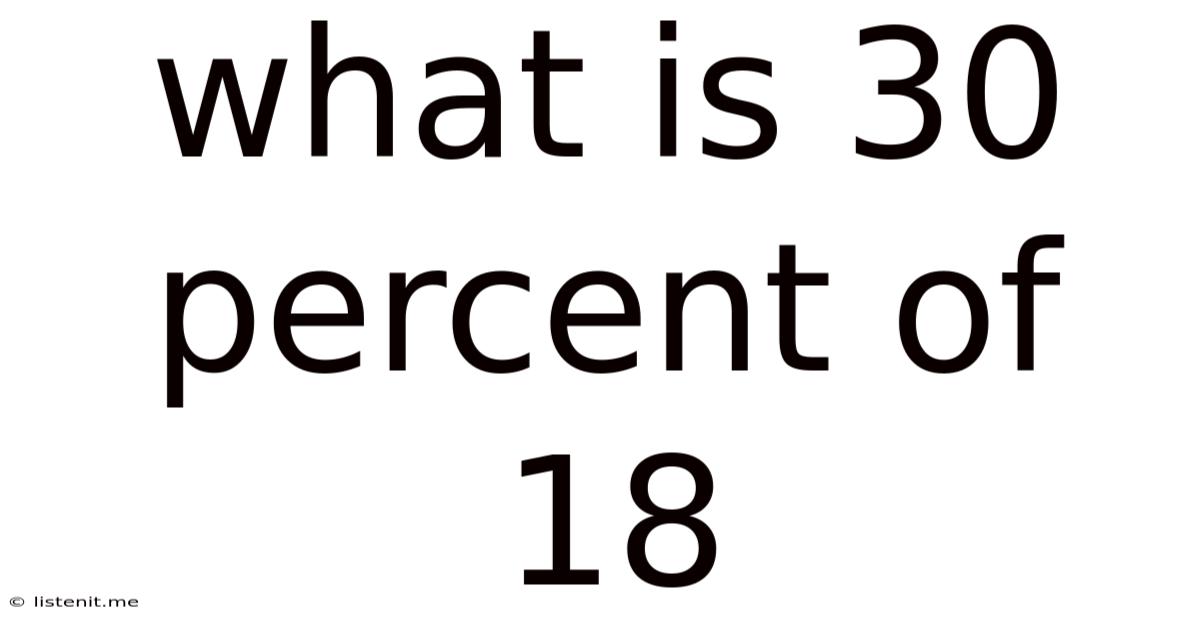
Table of Contents
What is 30 Percent of 18? A Comprehensive Guide to Percentages
Calculating percentages is a fundamental skill in many areas of life, from everyday budgeting and shopping to more complex financial calculations and scientific analyses. Understanding how to find a percentage of a number is crucial for making informed decisions and solving a wide range of problems. This in-depth guide will not only answer the question, "What is 30 percent of 18?" but will also equip you with the knowledge and techniques to tackle similar percentage calculations with ease.
Understanding Percentages
Before we dive into the calculation, let's solidify our understanding of percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per hundred" or "out of 100." For example, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5.
Method 1: Using the Decimal Equivalent
This is perhaps the most straightforward method for calculating percentages. The core idea is to convert the percentage into its decimal equivalent and then multiply it by the number.
Step 1: Convert the Percentage to a Decimal
To convert a percentage to a decimal, divide the percentage by 100. In our case, 30% becomes:
30% ÷ 100 = 0.3
Step 2: Multiply the Decimal by the Number
Now, multiply the decimal equivalent (0.3) by the number you want to find the percentage of (18):
0.3 × 18 = 5.4
Therefore, 30% of 18 is 5.4.
This method is efficient and easily adaptable to various percentage calculations. It's the preferred method for many due to its simplicity and direct application.
Method 2: Using the Fraction Equivalent
Percentages can also be expressed as fractions. This method offers a deeper understanding of the underlying mathematical principles.
Step 1: Convert the Percentage to a Fraction
To convert a percentage to a fraction, write the percentage as the numerator and 100 as the denominator. For 30%, this is:
30/100
Step 2: Simplify the Fraction (if possible)
We can simplify this fraction by dividing both the numerator and the denominator by their greatest common divisor, which is 10:
30/100 = 3/10
Step 3: Multiply the Fraction by the Number
Now, multiply the simplified fraction (3/10) by the number (18):
(3/10) × 18 = 54/10
Step 4: Convert the Result to a Decimal (if necessary)
Finally, convert the resulting fraction to a decimal by dividing the numerator by the denominator:
54/10 = 5.4
Therefore, 30% of 18 is 5.4. This method, while slightly longer, reinforces the connection between percentages, fractions, and decimals.
Method 3: Using Proportions
This method uses the concept of proportions to solve percentage problems. It's particularly helpful for visualizing the relationship between the parts and the whole.
Step 1: Set up a Proportion
We can set up a proportion to represent the problem:
x / 18 = 30 / 100
Where 'x' represents 30% of 18.
Step 2: Solve for x
To solve for 'x', we can cross-multiply:
100x = 30 × 18
100x = 540
x = 540 / 100
x = 5.4
Therefore, 30% of 18 is 5.4. The proportion method is a versatile technique applicable to a broader range of percentage problems.
Real-World Applications of Percentage Calculations
Understanding how to calculate percentages is essential in numerous real-world scenarios:
- Shopping and Discounts: Calculating discounts on sale items. For example, finding the final price of an item discounted by 20%.
- Finance and Budgeting: Determining interest earned on savings accounts, calculating taxes, and managing personal finances.
- Scientific Data Analysis: Expressing experimental results as percentages, calculating error margins, and analyzing statistical data.
- Business and Economics: Analyzing market share, calculating profit margins, and forecasting future trends.
- Everyday Life: Determining tips in restaurants, calculating percentages of ingredients in recipes, and understanding statistical information presented in news reports.
Beyond the Basics: More Complex Percentage Calculations
While this article focused on finding 30% of 18, the techniques discussed are applicable to a wide range of percentage problems, including:
- Finding the percentage increase or decrease: This involves calculating the difference between two values and expressing it as a percentage of the original value.
- Calculating the original value: If you know the percentage and the resulting value, you can work backward to find the original amount.
- Solving problems involving multiple percentages: This might involve calculating successive discounts or compound interest.
Mastering Percentage Calculations: Tips and Tricks
- Practice regularly: The more you practice, the more comfortable and efficient you'll become with percentage calculations.
- Use a calculator: For more complex calculations, a calculator can significantly speed up the process.
- Understand the underlying concepts: A strong grasp of the underlying principles of percentages, fractions, and decimals is crucial for efficient problem-solving.
- Check your work: Always double-check your answers to ensure accuracy.
Conclusion: The Power of Percentages
The ability to calculate percentages is a valuable skill with far-reaching applications. Whether you're dealing with simple discounts or complex financial analyses, a solid understanding of percentages will empower you to make informed decisions and navigate a wide range of situations confidently. We've explored multiple methods for calculating 30% of 18, illustrating the flexibility and adaptability of these fundamental mathematical concepts. By mastering these techniques and understanding the real-world applications, you'll be well-equipped to handle any percentage problem that comes your way. Remember, consistent practice is key to building proficiency and confidence in this crucial skill.
Latest Posts
Latest Posts
-
Find The Greatest Common Factor Of 50 25 And 100
May 24, 2025
-
18 Is What Percent Of 76
May 24, 2025
-
What Is 30 Percent Off Of 80 Dollars
May 24, 2025
-
What Day Will It Be 14 Days From Now
May 24, 2025
-
3 5 Divided By 5 6
May 24, 2025
Related Post
Thank you for visiting our website which covers about What Is 30 Percent Of 18 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.