18 Is What Percent Of 76
listenit
May 24, 2025 · 4 min read
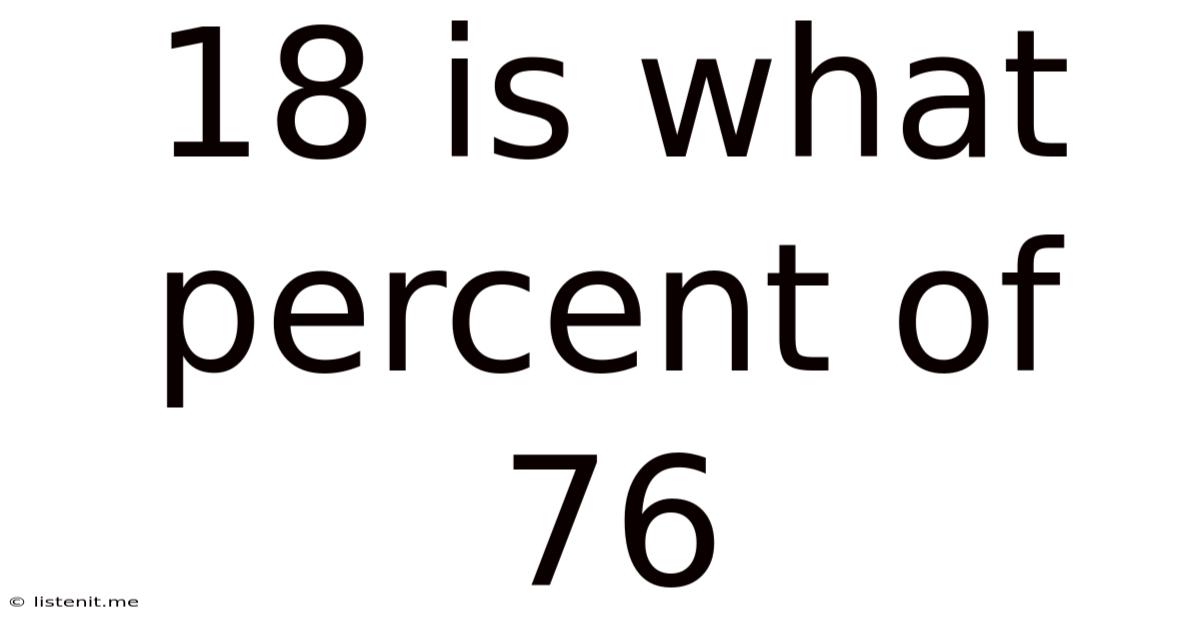
Table of Contents
18 is What Percent of 76? A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill with broad applications in various aspects of life, from everyday budgeting and shopping to complex financial analysis and scientific research. Understanding how to determine what percentage one number represents of another is crucial for making informed decisions and interpreting data accurately. This comprehensive guide will not only answer the question "18 is what percent of 76?" but also equip you with the knowledge and strategies to tackle similar percentage problems with confidence.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "per hundred." For example, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5. Percentages are used extensively to represent proportions, rates, and changes.
Key Concepts:
- The Whole: This is the total amount or the number you are considering as the base. In our problem, 76 is the whole.
- The Part: This is the portion of the whole you are interested in expressing as a percentage. In our problem, 18 is the part.
- The Percentage: This is the value that represents the part as a fraction of the whole, expressed as a percentage (%).
Calculating "18 is What Percent of 76?"
There are several ways to calculate what percentage 18 represents of 76. Let's explore the most common methods:
Method 1: Using the Formula
The basic formula for calculating percentages is:
(Part / Whole) x 100% = Percentage
Applying this to our problem:
(18 / 76) x 100% = Percentage
-
Divide the part by the whole: 18 / 76 ≈ 0.2368
-
Multiply the result by 100: 0.2368 x 100% ≈ 23.68%
Therefore, 18 is approximately 23.68% of 76.
Method 2: Using Proportions
We can also solve this problem using proportions. We can set up a proportion as follows:
18/76 = x/100
Where 'x' represents the percentage we are trying to find.
To solve for 'x', we cross-multiply:
18 * 100 = 76 * x
1800 = 76x
x = 1800 / 76
x ≈ 23.68
Therefore, 18 is approximately 23.68% of 76.
Method 3: Using a Calculator
Most calculators have a percentage function that simplifies the calculation. Simply enter "18 ÷ 76 x 100" and the calculator will provide the answer directly.
Practical Applications of Percentage Calculations
Understanding percentage calculations has widespread practical applications in various fields:
1. Finance and Budgeting:
- Calculating interest rates: Interest on loans, savings accounts, and investments is expressed as a percentage.
- Determining discounts and sales tax: Sales discounts and taxes are commonly calculated as percentages of the original price.
- Analyzing financial statements: Financial statements like income statements and balance sheets use percentages to represent various financial ratios and proportions.
- Tracking investment returns: Investment returns are frequently expressed as percentage gains or losses.
2. Science and Research:
- Representing experimental results: Scientific data often involves expressing results as percentages to show the proportion of a specific outcome.
- Calculating statistical probabilities: Probabilities are frequently expressed as percentages.
- Analyzing survey data: Survey results are often summarized using percentages to show the distribution of responses.
3. Everyday Life:
- Calculating tips: Calculating restaurant tips involves determining a percentage of the total bill.
- Understanding nutrition labels: Nutritional information on food labels often uses percentages to represent the recommended daily intake of various nutrients.
- Comparing prices: Percentage calculations can help compare the relative prices of different products or services.
- Calculating grades: Many grading systems express scores as percentages.
Expanding Your Percentage Calculation Skills
Mastering percentage calculations involves understanding the underlying concepts and applying the appropriate methods. Here are some tips to improve your skills:
- Practice regularly: The more you practice, the more comfortable and efficient you will become at calculating percentages.
- Use different methods: Try using different approaches (formula, proportions, calculator) to reinforce your understanding.
- Understand the context: Pay close attention to the context of the problem to ensure you are using the correct numbers as the "part" and the "whole."
- Check your work: Always double-check your calculations to ensure accuracy.
- Learn to estimate: Developing the ability to estimate percentages can help you quickly assess the reasonableness of your answers.
Beyond the Basics: More Complex Percentage Problems
While the problem "18 is what percent of 76?" is a relatively straightforward percentage calculation, many real-world applications involve more complex scenarios. These might include:
- Calculating percentage change: This involves finding the percentage increase or decrease between two values. For example, finding the percentage increase in sales from one year to the next.
- Calculating percentage points: This is a different concept than percentage change and refers to the difference between two percentages.
- Working with compound percentages: This involves calculating percentages of percentages, often seen in compound interest calculations.
Conclusion: Mastering Percentages for Success
The ability to accurately and efficiently calculate percentages is an essential skill with far-reaching applications across numerous fields. By understanding the fundamental concepts, mastering various calculation methods, and practicing regularly, you can build a strong foundation for tackling even the most complex percentage problems with confidence. This not only enhances your problem-solving abilities but also equips you with a critical skillset for navigating everyday life and succeeding in various professional endeavors. Remember the core formula – (Part / Whole) x 100% – and you’ll be well on your way to mastering the world of percentages.
Latest Posts
Latest Posts
-
30 Out Of 41 As A Percentage
May 24, 2025
-
4 2 9 As An Improper Fraction
May 24, 2025
-
When Is 45 Minutes From Now
May 24, 2025
-
What Is The Gcf Of 8 And 14
May 24, 2025
-
What Is The Greatest Common Factor Of 90 And 135
May 24, 2025
Related Post
Thank you for visiting our website which covers about 18 Is What Percent Of 76 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.