What Is 2 To The Negative 3rd Power
listenit
Mar 27, 2025 · 5 min read
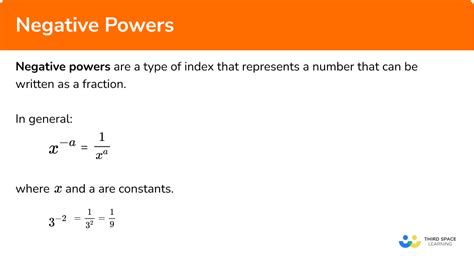
Table of Contents
What is 2 to the Negative 3rd Power? A Comprehensive Guide
Understanding exponents, especially negative ones, can be a stumbling block for many. This comprehensive guide will demystify the concept of "2 to the negative 3rd power," explaining not only the answer but also the underlying principles and broader applications of negative exponents. We'll explore the mechanics, provide practical examples, and delve into related mathematical concepts to ensure a solid grasp of this fundamental algebraic concept.
Understanding Exponents
Before tackling negative exponents, let's solidify our understanding of positive exponents. An exponent, also known as a power or index, indicates how many times a base number is multiplied by itself. For example:
- 2³ = 2 x 2 x 2 = 8 (2 to the power of 3, or 2 cubed)
- 5² = 5 x 5 = 25 (5 to the power of 2, or 5 squared)
- 10¹ = 10 (10 to the power of 1, which is simply 10 itself)
The base number is the number being multiplied, and the exponent tells us how many times to perform the multiplication.
Introducing Negative Exponents
The concept of negative exponents might initially seem counterintuitive, but it's a crucial part of mathematics. A negative exponent essentially signifies a reciprocal. This means it inverts the base number, turning it into a fraction. Specifically:
a⁻ⁿ = 1 / aⁿ
This formula is the key to understanding negative exponents. The negative exponent becomes positive when the base is inverted.
Calculating 2 to the Negative 3rd Power
Now, let's apply this knowledge to our central question: "What is 2 to the negative 3rd power?" Using the formula above, we can break this down step-by-step:
2⁻³ = 1 / 2³
First, we deal with the positive exponent part: 2³. This is 2 x 2 x 2 = 8.
Therefore:
2⁻³ = 1 / 8
So, 2 to the negative 3rd power is equal to 1/8 or 0.125.
Why Negative Exponents Work This Way
The consistent mathematical framework of exponents is built upon specific rules. The rule for negative exponents is not arbitrarily chosen, but rather stems from the fundamental principles of exponent manipulation. Consider the following:
If we multiply two numbers with the same base but different exponents, we add the exponents:
aᵐ x aⁿ = aᵐ⁺ⁿ
Let's apply this rule to a case involving a positive and a negative exponent:
2³ x 2⁻³ = 2³⁺⁽⁻³⁾ = 2⁰
Any number (except zero) raised to the power of zero equals 1. Therefore:
2³ x 2⁻³ = 1
From this, we can infer the relationship:
2⁻³ = 1 / 2³
This demonstration shows how the definition of a negative exponent aligns perfectly with established exponent rules, creating a coherent and logical system.
Practical Applications of Negative Exponents
Negative exponents aren't just abstract mathematical concepts; they have practical applications across various fields:
-
Scientific Notation: Negative exponents are extensively used in scientific notation to represent extremely small numbers. For example, the speed of light in meters per second (approximately 3 x 10⁸ m/s) can be expressed as 300,000,000 m/s and using the metric system, with its prefixes like milli (10⁻³), micro (10⁻⁶), nano (10⁻⁹), etc., negative exponents are critical for representing values on a vast scale.
-
Chemistry: In chemistry, negative exponents frequently appear in calculations involving molarity, concentration, and equilibrium constants. These constants often involve very small numbers, and negative exponents provide a compact and efficient way to express them.
-
Physics: Similar to chemistry, physics relies heavily on negative exponents in expressing various quantities, especially when dealing with subatomic particles and incredibly small scales of measurement.
-
Finance: Compounding interest and discounted cash flows commonly utilize negative exponents when calculating present values of future payments. These calculations require considering the time value of money, a concept where future values are discounted to their present-day worth.
-
Computer Science: Negative exponents appear in algorithms and data structures, particularly those dealing with time and space complexity analysis. These analyses are crucial in assessing the efficiency of computer programs and algorithms.
Expanding the Understanding: Fractional Exponents
The concept of negative exponents extends further into the realm of fractional exponents (exponents that are fractions). Fractional exponents represent roots and powers combined. For instance:
a^(m/n) = ⁿ√(aᵐ)
This means that a raised to the power of m/n is the nth root of a raised to the power of m. Negative fractional exponents combine the principles of both negative and fractional exponents.
For instance, let's consider:
2^(-2/3)
This is equivalent to:
1 / 2^(2/3) = 1 / ³√(2²) = 1 / ³√4
This demonstrates the seamless integration of negative and fractional exponents within the overall framework of exponent rules.
Mastering Exponents: Tips and Tricks
Here are some tips to help solidify your understanding and proficiency with exponents:
-
Practice Regularly: Consistent practice is key to mastering any mathematical concept, including exponents. Work through various problems with different bases and exponents.
-
Use Online Resources: Several websites and educational platforms offer interactive exercises and tutorials on exponents. These resources can provide valuable practice and feedback.
-
Break Down Complex Problems: Tackle complex exponent problems by breaking them into smaller, manageable steps. This approach allows for a clearer understanding of each part of the problem and reduces potential for errors.
-
Understand the Rules: Memorize and internalize the fundamental rules of exponents. This will act as your foundational knowledge, allowing you to confidently tackle complex scenarios.
-
Check Your Work: Always check your answers. Use a calculator or alternative methods to verify your calculations and ensure accuracy.
Conclusion
Understanding "2 to the negative 3rd power" isn't merely about knowing the answer (1/8); it's about grasping the fundamental principles of negative exponents, their relationship to reciprocals, and their widespread applications in various fields. By mastering these concepts, you'll enhance your mathematical skills and open the door to a deeper understanding of advanced mathematical topics. Remember the core concept: a negative exponent flips the base into a fraction, turning a potentially complicated calculation into a straightforward process of inversion and then exponentiation. Through diligent practice and the application of the principles explained here, mastering negative exponents will become a significant achievement in your mathematical journey.
Latest Posts
Latest Posts
-
What Is 39 6 Celsius In Fahrenheit
Mar 30, 2025
-
44 Is 55 Of What Number
Mar 30, 2025
-
How Does Price Discrimination Benefit Producers And Consumers
Mar 30, 2025
-
Which Element Is The Most Metallic
Mar 30, 2025
-
Do Srtrong Bases Completely Dissociate In Water
Mar 30, 2025
Related Post
Thank you for visiting our website which covers about What Is 2 To The Negative 3rd Power . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
