What Is 11/25 As A Percent
listenit
Mar 28, 2025 · 5 min read
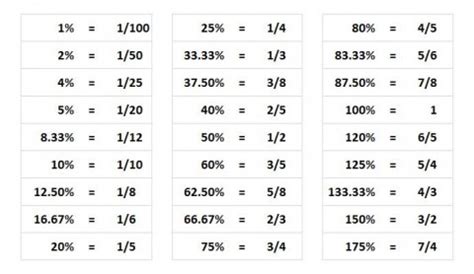
Table of Contents
What is 11/25 as a Percent? A Comprehensive Guide to Fraction-to-Percentage Conversion
Converting fractions to percentages is a fundamental skill in mathematics with broad applications in everyday life, from calculating discounts and tips to understanding statistics and financial data. This comprehensive guide will delve into the process of converting the fraction 11/25 into a percentage, exploring various methods and offering insights into the underlying mathematical principles. We'll also look at practical applications and related concepts to solidify your understanding.
Understanding Fractions and Percentages
Before we dive into the conversion, let's refresh our understanding of fractions and percentages.
Fractions: A fraction represents a part of a whole. It's expressed as a ratio of two numbers, the numerator (top number) and the denominator (bottom number). The numerator indicates the number of parts you have, and the denominator indicates the total number of parts in the whole. For example, in the fraction 11/25, 11 represents the number of parts and 25 represents the total number of equal parts.
Percentages: A percentage is a way of expressing a fraction or decimal as a proportion of 100. The percentage symbol (%) signifies "per hundred." Essentially, a percentage tells you what part of 100 something represents. For instance, 50% means 50 out of 100, or half.
Converting 11/25 to a Percentage: Method 1 - Using Decimal Conversion
This is arguably the most straightforward method. It involves two steps:
-
Convert the fraction to a decimal: To do this, divide the numerator (11) by the denominator (25):
11 ÷ 25 = 0.44
-
Convert the decimal to a percentage: Multiply the decimal by 100 and add the percentage symbol (%):
0.44 × 100 = 44%
Therefore, 11/25 is equal to 44%.
Understanding the Logic
This method works because a percentage is essentially a fraction with a denominator of 100. By dividing the numerator by the denominator, we find the equivalent decimal representation. Multiplying by 100 then scales this decimal to represent the equivalent proportion out of 100, giving us the percentage.
Converting 11/25 to a Percentage: Method 2 - Finding an Equivalent Fraction
This method utilizes the principle of equivalent fractions – fractions that represent the same value, even though they have different numerators and denominators. Our goal is to convert the denominator of 11/25 to 100.
-
Determine the scaling factor: To change the denominator from 25 to 100, we need to multiply it by 4 (100 ÷ 25 = 4).
-
Apply the scaling factor to both the numerator and the denominator: To maintain the same value, we must multiply both the numerator and the denominator by the same factor:
(11 × 4) / (25 × 4) = 44/100
-
Express as a percentage: A fraction with a denominator of 100 can be directly expressed as a percentage. The numerator becomes the percentage value:
44/100 = 44%
The Importance of Equivalent Fractions
This method reinforces the concept of equivalent fractions, a crucial concept in understanding rational numbers and their manipulation. The principle of multiplying both the numerator and the denominator by the same number doesn't change the fraction's inherent value, only its representation.
Practical Applications of Fraction-to-Percentage Conversion
The ability to convert fractions to percentages is valuable in many real-world scenarios:
-
Calculating Discounts: A store offers a 11/25 discount on an item. Converting this fraction to a percentage (44%) makes it easier to understand the actual discount amount.
-
Understanding Financial Data: Financial reports often use fractions to represent ratios like profit margins or debt-to-equity ratios. Converting these to percentages provides a clearer picture of financial health.
-
Analyzing Statistics: Statistical data frequently involves fractions. Converting them to percentages allows for easier comparison and interpretation of results.
-
Calculating Grades: In some grading systems, your grade might be expressed as a fraction of points earned versus total possible points. Converting to a percentage gives you a readily understandable grade.
-
Cooking and Baking: Recipes sometimes use fractions to represent ingredient quantities. Converting to percentages can be helpful for scaling recipes up or down.
Beyond 11/25: Generalizing the Conversion Process
The methods described above can be applied to convert any fraction to a percentage. The core principles remain the same:
-
Convert the fraction to a decimal: Divide the numerator by the denominator.
-
Convert the decimal to a percentage: Multiply the decimal by 100 and add the percentage symbol.
Alternatively, you can find an equivalent fraction with a denominator of 100 by identifying the appropriate scaling factor.
Troubleshooting Common Mistakes
While the process is relatively straightforward, a few common mistakes can occur:
-
Incorrect division: Ensure you accurately divide the numerator by the denominator when converting to a decimal.
-
Forgetting to multiply by 100: Remember to multiply the decimal by 100 to express it as a percentage.
-
Incorrect scaling factor: When using the equivalent fraction method, double-check that you've calculated the correct scaling factor to reach a denominator of 100.
Advanced Concepts and Further Exploration
For those seeking a deeper understanding, exploring the following concepts can enhance your mathematical skills:
-
Ratio and Proportion: Understanding ratios and proportions provides a more comprehensive framework for working with fractions and percentages.
-
Decimal Places and Rounding: In some cases, you might need to round the decimal to a specific number of decimal places before converting it to a percentage.
-
Percentage Increase and Decrease: Learn how to calculate percentage changes, which involves understanding how to determine the percentage difference between two values.
-
Working with Percentages in Spreadsheets and Programming: Explore how to use functions in software like Microsoft Excel or programming languages to automate fraction-to-percentage conversions.
Conclusion
Converting 11/25 to a percentage (44%) is a straightforward process involving either decimal conversion or finding an equivalent fraction with a denominator of 100. Mastering this conversion is crucial for various practical applications, from everyday calculations to advanced mathematical analyses. By understanding the underlying principles and practicing the methods outlined in this guide, you can confidently tackle fraction-to-percentage conversions and enhance your mathematical proficiency. Remember to practice regularly and explore related concepts to solidify your understanding and expand your mathematical skills.
Latest Posts
Latest Posts
-
How Many Even Numbers Are On A Dice
Mar 31, 2025
-
What Do Plants Have In Common With Animals
Mar 31, 2025
-
What Is 5 16 In Decimal Form
Mar 31, 2025
-
What Is The Square Root Of 70
Mar 31, 2025
-
What Is The Current Model Of The Atom
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about What Is 11/25 As A Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
