What Is The Square Root Of 70
listenit
Mar 31, 2025 · 5 min read
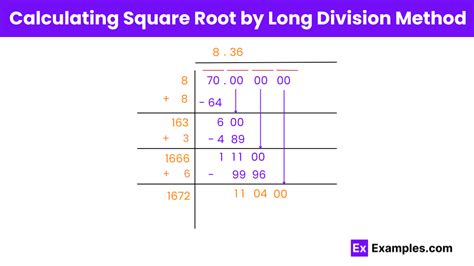
Table of Contents
What is the Square Root of 70? A Deep Dive into Calculation and Applications
The seemingly simple question, "What is the square root of 70?" opens a door to a fascinating exploration of mathematical concepts, calculation methods, and real-world applications. While a simple calculator provides a decimal approximation, understanding the underlying principles offers a much richer understanding. This article will delve into various methods of calculating the square root of 70, exploring its significance in different fields and showcasing its practical uses.
Understanding Square Roots
Before we tackle the square root of 70 specifically, let's establish a fundamental understanding of square roots. The square root of a number is a value that, when multiplied by itself, equals the original number. For instance, the square root of 9 is 3 because 3 * 3 = 9. This is often represented mathematically as √9 = 3.
However, not all numbers have perfect square roots – integers that result in a whole number when squared. 70 falls into this category. Its square root is an irrational number, meaning it cannot be expressed as a simple fraction and its decimal representation continues infinitely without repeating.
Calculating the Square Root of 70
Several methods exist for calculating the square root of 70, each with its own level of complexity and accuracy:
1. Using a Calculator: The Quickest Method
The simplest approach is using a calculator. Most standard calculators have a square root function (√). Simply input 70 and press the square root button. You'll obtain an approximation like 8.3666. This is the most common method due to its speed and ease of use, perfect for everyday calculations.
2. The Babylonian Method (or Heron's Method): An Iterative Approach
This ancient method provides a progressively more accurate approximation through iterative refinement. It starts with an initial guess and refines it through successive calculations.
Steps:
- Make an initial guess: Let's start with 8, as 8² = 64, which is close to 70.
- Improve the guess: Divide 70 by the guess and average the result with the guess: (70/8 + 8)/2 = 8.375
- Repeat: Use the new guess (8.375) and repeat the process: (70/8.375 + 8.375)/2 ≈ 8.3666
- Continue: Repeating this process will yield increasingly accurate approximations. The more iterations, the closer the result gets to the actual square root.
The Babylonian method demonstrates a powerful iterative approach to finding approximations, highlighting the concept of converging towards a solution.
3. The Long Division Method: A Manual Approach
For those who enjoy a more manual approach, the long division method for finding square roots provides a step-by-step calculation. While more time-consuming, it offers a deeper understanding of the underlying process. This method is somewhat intricate and better explained through a visual demonstration, but the core concept involves successively grouping digits and subtracting perfect squares to arrive at the approximation. This method is less practical for everyday calculations given the availability of calculators but provides valuable insight into the mathematics involved.
4. Using Logarithms: A Less Common Approach
Logarithms can also be used to calculate square roots. The formula involves utilizing the logarithm property that log(√x) = (1/2)log(x). By finding the logarithm of 70, dividing it by 2, and then finding the antilogarithm, one can arrive at the square root. While mathematically sound, this method is less intuitive and less efficient than other methods for this specific task.
The Irrational Nature of √70
It is crucial to emphasize that the square root of 70 is an irrational number. This means its decimal representation is non-terminating and non-repeating. The approximations calculated using the methods above are just that – approximations. The true value of √70 stretches infinitely, making it impossible to represent it precisely using a finite number of digits.
Applications of Square Roots
Understanding square roots is essential in various fields:
1. Geometry: Calculating Distances and Areas
Square roots are fundamental in geometry, particularly when dealing with the Pythagorean theorem (a² + b² = c²), used to calculate the length of the hypotenuse in right-angled triangles. Many geometric calculations involving distances, areas, and volumes necessitate the use of square roots.
2. Physics: Velocity and Acceleration
In physics, square roots frequently appear in formulas related to velocity, acceleration, and energy calculations. For instance, calculating the final velocity in free fall involves the square root of the acceleration due to gravity and the distance fallen.
3. Statistics: Standard Deviation
Standard deviation, a crucial measure of data dispersion in statistics, relies heavily on square roots. Calculating the standard deviation involves summing squared differences, then finding the square root of the result, providing a meaningful measure of the spread of data points around the mean.
4. Engineering: Structural Calculations
Engineers use square roots extensively in structural calculations to determine stresses, strains, and deflections in various structures. These calculations ensure structural integrity and safety.
Conclusion: Beyond the Numerical Answer
While the approximate value of the square root of 70, around 8.3666, is readily available through a calculator, the journey to understanding how this value is obtained provides a deeper appreciation for mathematical concepts and their applications in the real world. The various methods outlined above, from simple calculator use to iterative approaches like the Babylonian method, showcase different perspectives on problem-solving and highlight the power of numerical approximation. Understanding the irrational nature of √70, along with its significant applications across diverse fields, solidifies its importance in mathematics and beyond. Therefore, the answer to "What is the square root of 70?" is not simply a number, but a gateway to a wealth of mathematical concepts and practical applications.
Latest Posts
Latest Posts
-
How Many Right Angles Does A Quadrilateral Have
Apr 01, 2025
-
Is Magnesium A Gas Liquid Or Solid
Apr 01, 2025
-
Where In The Chloroplast Do The Light Reactions Take Place
Apr 01, 2025
-
What Is All The Colors Combined
Apr 01, 2025
-
How Does Friction Force Affect Motion
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about What Is The Square Root Of 70 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
