Least Common Multiple Of 25 And 35
listenit
Apr 05, 2025 · 5 min read
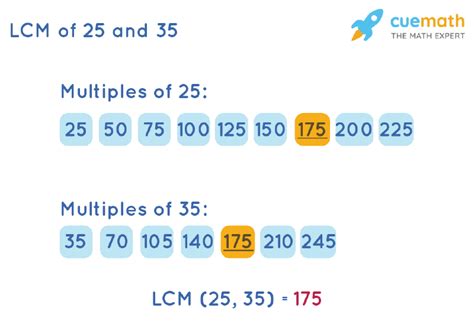
Table of Contents
Finding the Least Common Multiple (LCM) of 25 and 35: A Deep Dive
The least common multiple (LCM) is a fundamental concept in mathematics, particularly in number theory and arithmetic. Understanding how to find the LCM is crucial for various applications, from simplifying fractions to solving problems involving cyclical events. This article will delve deep into finding the LCM of 25 and 35, exploring different methods and providing a comprehensive understanding of the underlying principles. We’ll also touch on the broader significance of LCMs and their practical uses.
Understanding Least Common Multiple (LCM)
Before we tackle the specific problem of finding the LCM of 25 and 35, let's solidify our understanding of the concept itself. The least common multiple of two or more integers is the smallest positive integer that is a multiple of all the integers. In simpler terms, it's the smallest number that all the given numbers divide into evenly.
For instance, let's consider the numbers 2 and 3. The multiples of 2 are 2, 4, 6, 8, 10, 12... and the multiples of 3 are 3, 6, 9, 12, 15... The common multiples of 2 and 3 are 6, 12, 18... and the smallest of these is 6. Therefore, the LCM of 2 and 3 is 6.
Methods for Finding the LCM
There are several methods to calculate the LCM of two or more numbers. We'll explore the most common and effective techniques:
1. Listing Multiples Method
This method is straightforward but can be time-consuming for larger numbers. We list the multiples of each number until we find the smallest common multiple.
Let's apply this to 25 and 35:
- Multiples of 25: 25, 50, 75, 100, 125, 150, 175, 200...
- Multiples of 35: 35, 70, 105, 140, 175, 210...
Notice that 175 appears in both lists. Therefore, the LCM of 25 and 35 is 175. This method works well for smaller numbers but becomes less efficient as the numbers increase.
2. Prime Factorization Method
This is a more efficient method, particularly for larger numbers. It involves finding the prime factorization of each number and then constructing the LCM using the highest powers of all prime factors present.
- Prime factorization of 25: 5² (25 = 5 x 5)
- Prime factorization of 35: 5 x 7
To find the LCM, we take the highest power of each prime factor present in either factorization:
- Highest power of 5: 5² = 25
- Highest power of 7: 7¹ = 7
LCM(25, 35) = 5² x 7 = 25 x 7 = 175
This method is generally preferred for its efficiency and scalability.
3. Greatest Common Divisor (GCD) Method
This method leverages the relationship between the LCM and the greatest common divisor (GCD) of two numbers. The GCD is the largest number that divides both numbers evenly. The formula relating LCM and GCD is:
LCM(a, b) = (a x b) / GCD(a, b)
First, we need to find the GCD of 25 and 35. Using the Euclidean algorithm (a common method for finding the GCD):
- Divide the larger number (35) by the smaller number (25): 35 ÷ 25 = 1 with a remainder of 10.
- Replace the larger number with the smaller number (25) and the smaller number with the remainder (10): 25 ÷ 10 = 2 with a remainder of 5.
- Repeat: 10 ÷ 5 = 2 with a remainder of 0.
The last non-zero remainder is the GCD, which is 5.
Now, we can use the formula:
LCM(25, 35) = (25 x 35) / 5 = 875 / 5 = 175
This method is also efficient and demonstrates the interconnectedness of LCM and GCD.
Applications of LCM
The concept of LCM has wide-ranging applications across various fields:
1. Fraction Arithmetic
Finding a common denominator when adding or subtracting fractions requires finding the LCM of the denominators. For example, to add 1/25 and 1/35, we find the LCM of 25 and 35 (which is 175), and then express both fractions with a denominator of 175 before adding them.
2. Cyclical Events
LCM is useful in problems involving events that repeat at regular intervals. For instance, if two buses leave a station at different intervals, the LCM of those intervals will tell you when both buses will depart simultaneously again.
3. Scheduling and Time Management
Consider scenarios involving tasks that need to be performed at regular intervals. Finding the LCM of these intervals helps determine when these tasks will coincide. This is important in project management and scheduling.
4. Gear Ratios and Mechanical Engineering
In mechanical systems, gear ratios often involve finding the LCM to determine the synchronization of rotating components.
5. Music Theory
LCM is used in music theory to find the least common multiple of the periods of different musical rhythms. This is crucial for harmonizing rhythms.
Expanding on the LCM Concept
The methods described above can be extended to find the LCM of more than two numbers. For the prime factorization method, you simply include all prime factors from all numbers, taking the highest power of each. For the GCD method, you can extend it iteratively, finding the LCM of two numbers at a time.
Conclusion: The Importance of Understanding LCM
The least common multiple is a fundamental mathematical concept with significant practical implications across diverse fields. Understanding the different methods for calculating the LCM, such as the prime factorization method and the GCD method, is essential for efficiently solving problems involving multiples, fractions, and cyclical events. The ability to calculate the LCM empowers individuals to tackle a wide range of challenges, from simple arithmetic to complex engineering problems. Mastering this concept lays a strong foundation for further exploration in mathematics and its applications. Remember, practice is key to mastering the concept and applying it effectively in different contexts. Try working through various examples, starting with small numbers and gradually progressing to larger ones to reinforce your understanding.
Latest Posts
Latest Posts
-
How To Find Perpendicular Slope Of A Line
Apr 05, 2025
-
Greatest Common Factor Of 18 And 15
Apr 05, 2025
-
Find The Zeros Of A Function Algebraically
Apr 05, 2025
-
Does Ethylene Glycol Have Ion Dipole Forces
Apr 05, 2025
-
What Is Freezing In Celsius Degrees
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about Least Common Multiple Of 25 And 35 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
