Is The Distance Formula Derived From The Pythagorean Theorem
listenit
Mar 30, 2025 · 5 min read
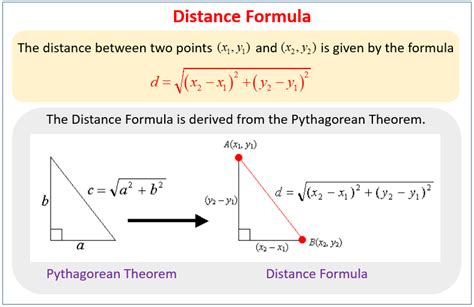
Table of Contents
Is the Distance Formula Derived from the Pythagorean Theorem? A Deep Dive
The distance formula, a cornerstone of coordinate geometry, allows us to calculate the distance between any two points in a Cartesian coordinate system. But where does this seemingly simple formula originate? The answer lies in the elegant and powerful Pythagorean theorem. This article will delve into a comprehensive exploration of the relationship between the distance formula and the Pythagorean theorem, demonstrating how the latter forms the fundamental basis of the former. We will move beyond a simple explanation, exploring the underlying mathematical principles and considering its applications in various fields.
Understanding the Pythagorean Theorem
Before diving into the distance formula, let's solidify our understanding of the Pythagorean theorem. This fundamental theorem of geometry states that in a right-angled triangle, the square of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides (called legs or cathetus). Mathematically, it's represented as:
a² + b² = c²
where:
- a and b are the lengths of the legs of the right-angled triangle.
- c is the length of the hypotenuse.
This theorem has been known for millennia and holds profound significance in mathematics and beyond. Its applications extend far beyond simple triangle calculations, influencing fields like engineering, architecture, and computer graphics.
The Visual Representation: Understanding the Geometry
Imagine a right-angled triangle with legs of length 'a' and 'b', and a hypotenuse of length 'c'. The Pythagorean theorem visually represents the relationship between these sides. If you were to construct squares on each side of the triangle, the area of the square on the hypotenuse (c²) would be equal to the sum of the areas of the squares on the legs (a² + b²). This visual representation offers an intuitive understanding of the theorem's essence.
Deriving the Distance Formula from the Pythagorean Theorem
Now, let's explore how the Pythagorean theorem forms the foundation of the distance formula. Consider two points in a Cartesian coordinate system: Point A with coordinates (x₁, y₁) and Point B with coordinates (x₂, y₂). To find the distance between these two points, we can use the Pythagorean theorem.
-
Construct a Right-Angled Triangle: Imagine drawing a right-angled triangle with the line segment AB as the hypotenuse. The legs of this triangle are formed by the horizontal and vertical distances between the points.
-
Calculate the Lengths of the Legs: The horizontal leg's length is the difference between the x-coordinates of the two points: |x₂ - x₁|. Similarly, the vertical leg's length is the difference between the y-coordinates: |y₂ - y₁|. The absolute value ensures we obtain a positive length.
-
Applying the Pythagorean Theorem: Now, we can apply the Pythagorean theorem to this right-angled triangle:
d² = (x₂ - x₁)² + (y₂ - y₁)²
where 'd' represents the distance between points A and B.
-
Solving for the Distance: Taking the square root of both sides of the equation, we arrive at the distance formula:
d = √[(x₂ - x₁)² + (y₂ - y₁)²]
This formula elegantly provides a direct method to calculate the distance between any two points in a two-dimensional Cartesian plane, purely derived from the Pythagorean theorem.
Extending the Distance Formula to Three Dimensions
The elegance of the Pythagorean theorem and its application in deriving the distance formula extend seamlessly to three-dimensional space. Consider two points A(x₁, y₁, z₁) and B(x₂, y₂, z₂) in a three-dimensional Cartesian coordinate system. Following a similar approach:
-
Construct a Right-Angled Triangle (in 3D): We can conceptually construct a right-angled triangle in three dimensions. This involves visualizing the line segment AB as the hypotenuse and the differences in x, y, and z coordinates forming the legs.
-
Apply the Pythagorean Theorem (repeatedly): We apply the Pythagorean theorem twice. First, find the distance in the xy-plane (using the 2D distance formula):
dxy = √[(x₂ - x₁)² + (y₂ - y₁)²]
Then, consider this distance and the difference in the z-coordinate as legs of another right-angled triangle, applying the Pythagorean theorem again to find the distance in three dimensions:
d = √[dxy² + (z₂ - z₁)²] = √[(x₂ - x₁)² + (y₂ - y₁)² + (z₂ - z₁)²]
Therefore, the distance formula in three dimensions becomes:
d = √[(x₂ - x₁)² + (y₂ - y₁)² + (z₂ - z₁)²]
This demonstrates the versatility of the Pythagorean theorem in extending the distance calculation into higher dimensions.
Applications of the Distance Formula
The distance formula's applications are widespread across numerous fields:
-
Geometry: Calculating the lengths of sides in geometric shapes, determining the distance between points on a graph, and solving geometric problems.
-
Physics: Determining the distance traveled by an object, calculating the shortest distance between two points in a physical space, and modeling projectile motion.
-
Computer Graphics: Used extensively in rendering algorithms, game development (calculating distances between objects and characters), and simulations (determining proximity and collisions).
-
Navigation: Used in GPS systems to calculate the distance between two geographical locations (with appropriate adjustments for the Earth's curvature).
-
Machine Learning and Data Science: Calculating distances between data points in feature space is fundamental to various machine learning algorithms (e.g., k-nearest neighbors, clustering algorithms).
Beyond Cartesian Coordinates: Exploring Other Coordinate Systems
While we've focused primarily on Cartesian coordinates, it's important to note that the distance formula can be adapted for other coordinate systems. For example, in polar coordinates, the distance calculation involves a different approach but still relies on underlying geometric principles. The specific formula will vary depending on the coordinate system used.
Conclusion: The Enduring Power of the Pythagorean Theorem
The distance formula, a seemingly straightforward tool in coordinate geometry, rests firmly on the foundation of the Pythagorean theorem. This deep connection demonstrates the enduring power and relevance of the Pythagorean theorem, highlighting its significance not just as a historical landmark in mathematics but as a living and vital component of modern mathematical tools. Its applications extend far beyond simple geometric problems, permeating various scientific and technological fields. By understanding the derivation of the distance formula from the Pythagorean theorem, we gain a deeper appreciation for the elegance and utility of these fundamental mathematical concepts. The ability to apply these principles in different scenarios underscores their ongoing importance in various disciplines, proving their timeless relevance and enduring power. From the simple act of measuring distance between points to complex calculations in computer graphics and machine learning, the Pythagorean theorem continues to be an invaluable cornerstone of mathematical understanding.
Latest Posts
Latest Posts
-
If The Earth Was Not Tilted What Would Happen
Apr 01, 2025
-
How To Find Mass Of Planet
Apr 01, 2025
-
What Is 4 5 In A Fraction
Apr 01, 2025
-
Which Element Is Found In All Organic Compounds
Apr 01, 2025
-
Is Supporting Combustion A Physical Property
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Is The Distance Formula Derived From The Pythagorean Theorem . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
