Is 5 7 A Rational Number
listenit
Mar 28, 2025 · 4 min read
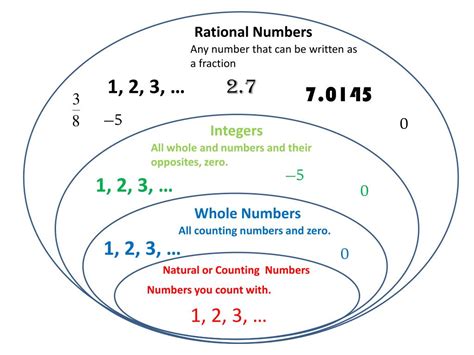
Table of Contents
Is 5/7 a Rational Number? A Deep Dive into Rational and Irrational Numbers
The question, "Is 5/7 a rational number?" seems simple at first glance. However, understanding the answer fully requires a deeper exploration of what defines rational and irrational numbers. This comprehensive guide will not only answer this specific question definitively but also equip you with a robust understanding of these fundamental number types. We'll delve into their definitions, explore examples, and discuss the implications of this classification.
Understanding Rational Numbers
A rational number is any number that can be expressed as a fraction p/q, where 'p' and 'q' are integers (whole numbers, including zero), and 'q' is not equal to zero. This seemingly simple definition has profound implications. The key is the ability to represent the number precisely as a ratio of two integers.
Examples of Rational Numbers:
- Integers: All integers are rational numbers. For example, 5 can be expressed as 5/1, -3 as -3/1, and 0 as 0/1.
- Fractions: The most obvious examples are common fractions like 1/2, 3/4, and -2/5. These are already in the p/q form.
- Terminating Decimals: Decimals that end after a finite number of digits are rational. For example, 0.75 can be written as 3/4, and 0.125 as 1/8.
- Repeating Decimals: Decimals that have a repeating pattern of digits are also rational. For example, 0.333... (one-third) can be expressed as 1/3, and 0.142857142857... (1/7) is a repeating decimal representation of a rational number.
The ability to convert a decimal to a fraction (and vice-versa) is a crucial aspect of identifying rational numbers. If a decimal terminates or repeats, it's rational. If it neither terminates nor repeats, it's irrational (more on that below).
Understanding Irrational Numbers
Irrational numbers are numbers that cannot be expressed as a fraction p/q, where p and q are integers and q is not zero. This means they cannot be written as a ratio of two integers. Their decimal representations are non-terminating and non-repeating; they go on forever without ever settling into a repeating pattern.
Examples of Irrational Numbers:
- √2 (the square root of 2): This is a classic example. It cannot be expressed precisely as a fraction. Its decimal representation is approximately 1.41421356..., continuing infinitely without a repeating pattern.
- π (pi): The ratio of a circle's circumference to its diameter, pi is approximately 3.14159..., but this is only an approximation. Its decimal representation goes on forever without repeating.
- e (Euler's number): This is a fundamental mathematical constant approximately equal to 2.71828..., with a non-terminating, non-repeating decimal expansion.
- The Golden Ratio (φ): Approximately 1.618..., also has a non-terminating, non-repeating decimal representation.
Is 5/7 a Rational Number? The Definitive Answer
Now, let's return to our original question: Is 5/7 a rational number? The answer is a resounding yes.
The number 5/7 is already expressed in the form p/q, where p = 5 and q = 7. Both 5 and 7 are integers, and q (7) is not zero. Therefore, it perfectly fits the definition of a rational number.
Its decimal representation is approximately 0.714285714285..., which is a repeating decimal. This further confirms its rational nature, as repeating decimals are always rational.
Proof and Further Exploration: Converting Fractions to Decimals
To solidify our understanding, let's explore how we can convert 5/7 into a decimal to illustrate the repeating pattern. We can perform long division:
0.7142857...
7 | 5.0000000
-4.9
----
0.10
-0.07
----
0.030
-0.028
----
0.0020
-0.0014
----
0.00060
-0.00056
----
0.000040
-0.000035
----
0.0000050...
As you can see, the division process continues indefinitely, producing the repeating block 714285. This repeating decimal representation is characteristic of rational numbers.
Distinguishing Rational from Irrational: A Practical Approach
While the theoretical definitions are crucial, a practical approach for determining if a number is rational often involves examining its decimal representation. If it terminates or repeats, it's rational. If it neither terminates nor repeats, it's irrational. However, this method relies on our ability to observe the entire decimal expansion, which is often impossible for irrational numbers due to their infinite non-repeating nature.
For fractions, the process is straightforward: if it's a fraction of two integers (with a non-zero denominator), it's rational.
The Importance of Understanding Rational and Irrational Numbers
Understanding the distinction between rational and irrational numbers is fundamental in mathematics and many related fields. This classification impacts:
- Algebra: Solving equations and working with different number systems.
- Calculus: Understanding limits, continuity, and the behavior of functions.
- Geometry: Working with measurements and calculations involving circles and other shapes.
- Computer Science: Representing numbers in computer systems and handling precision issues.
Conclusion: 5/7 is a Rational Number, and Why it Matters
To reiterate, 5/7 is undeniably a rational number. Its representation as a fraction of two integers and its repeating decimal expansion firmly place it within this category. The ability to confidently identify and classify numbers like 5/7 is essential for a solid foundation in mathematics and its numerous applications. This distinction is not just a theoretical exercise; it has practical implications across diverse fields, highlighting the importance of understanding the nuances of rational and irrational numbers. By mastering this concept, you enhance your mathematical capabilities and strengthen your understanding of the building blocks of numerical systems.
Latest Posts
Latest Posts
-
What Is 6 To The Zeroth Power
Mar 31, 2025
-
Which Intermolecular Force Is The Weakest
Mar 31, 2025
-
How To Calculate Molar Mass Of A Gas
Mar 31, 2025
-
The Weaker The Acid The Stronger The Conjugate Base
Mar 31, 2025
-
How Many Neutrons Does Barium Have
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about Is 5 7 A Rational Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
