In Order For The Parallelogram To Be A Rhombus X
listenit
Mar 29, 2025 · 6 min read
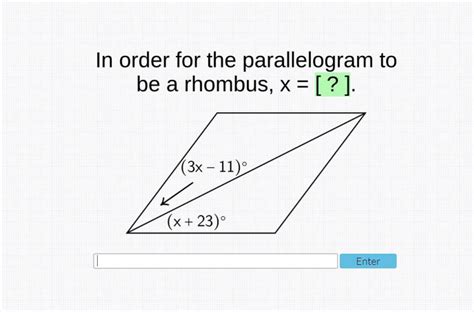
Table of Contents
In Order for a Parallelogram to be a Rhombus: Exploring the Defining Properties
A parallelogram, a quadrilateral with opposite sides parallel, is a fundamental shape in geometry. But within the family of parallelograms lies a special case: the rhombus. Understanding what distinguishes a rhombus from a general parallelogram is crucial for various mathematical applications. This article delves deep into the necessary and sufficient conditions for a parallelogram to be classified as a rhombus, exploring the geometric properties that define this unique quadrilateral.
Understanding the Parallelogram
Before we delve into the specifics of a rhombus, let's solidify our understanding of its parent shape: the parallelogram. A parallelogram possesses several key characteristics:
- Opposite sides are parallel: This is the defining characteristic. Lines extending from opposite sides will never intersect.
- Opposite sides are congruent: The lengths of opposite sides are equal.
- Opposite angles are congruent: The measures of opposite angles are equal.
- Consecutive angles are supplementary: The sum of any two consecutive angles is 180 degrees.
- Diagonals bisect each other: The diagonals intersect at their midpoints.
These properties are fundamental and apply to all parallelograms, including rhombuses, rectangles, and squares. However, rhombuses possess additional defining characteristics that set them apart.
The Defining Properties of a Rhombus
A rhombus is a special type of parallelogram where all four sides are congruent. This simple yet powerful addition transforms the parallelogram into a rhombus, enriching its geometric properties. Let's explore how this congruence affects the shape:
-
Equal Side Lengths: The most straightforward characteristic is that all four sides have the same length. This is the primary differentiator between a general parallelogram and a rhombus. While a parallelogram only requires opposite sides to be equal, a rhombus demands all sides to be equal.
-
Diagonals are Perpendicular Bisectors: In a rhombus, the diagonals not only bisect each other (a property inherited from the parallelogram), but they also intersect at right angles (90 degrees). This perpendicular bisecting property is unique to rhombuses and is a powerful tool for solving geometric problems.
-
Diagonals Bisect Angles: The diagonals of a rhombus also bisect the angles at each vertex. Each diagonal divides the corresponding angle into two congruent angles. This property stems directly from the equal side lengths and the perpendicular bisectors.
-
Area Calculation: The area of a rhombus can be calculated using different formulas, reflecting its unique properties. The most common formulas are:
-
A = s² sin θ: Where 's' is the length of a side and 'θ' is one of the interior angles. This formula highlights the relationship between side length and angle.
-
A = ½ d₁ d₂: Where 'd₁' and 'd₂' are the lengths of the two diagonals. This formula leverages the perpendicular bisecting nature of the diagonals.
-
Proving a Parallelogram is a Rhombus: Key Methods
Several methods can be used to prove that a given parallelogram is also a rhombus. These methods utilize the defining properties we've discussed:
1. Proving All Sides are Congruent:
This is the most direct method. If you can demonstrate that all four sides of a parallelogram are of equal length using geometric theorems, postulates, or measurements, you have conclusively proven it's a rhombus. This might involve using congruent triangles, distance formulas, or other techniques depending on the context of the problem.
2. Proving Diagonals are Perpendicular:
Showing that the diagonals intersect at a right angle is another effective method. If you can demonstrate that the diagonals are perpendicular bisectors of each other within a parallelogram, the parallelogram is necessarily a rhombus. This often involves using slope calculations in coordinate geometry or applying angle theorems in geometric proofs.
3. Proving Diagonals Bisect Angles:
Demonstrating that the diagonals bisect the angles at each vertex provides a third route to proving a parallelogram is a rhombus. This method requires showing that the angles formed by the intersection of the diagonals are congruent. Similar to the previous method, this might involve using angle relationships within triangles or other geometric principles.
4. Using Coordinate Geometry:
When working with parallelograms defined by coordinates, you can utilize the distance formula to calculate the lengths of all four sides. If all four sides have equal lengths, the parallelogram is a rhombus. Similarly, the slope formula can help determine if the diagonals are perpendicular, offering another avenue for proving the rhombus classification.
Real-World Applications of Rhombuses
Rhombuses, despite their seemingly simple definition, appear frequently in real-world scenarios and various applications:
-
Crystals: The structure of many crystals naturally forms rhombus shapes, reflecting the underlying molecular arrangements.
-
Art and Design: Artists and designers utilize rhombuses to create visual patterns and textures, capitalizing on their symmetry and aesthetic appeal.
-
Engineering: Certain engineering designs, particularly those involving trusses and frameworks, leverage the strength and stability of rhombus structures. The rigid nature of a rhombus makes it a suitable choice for load-bearing applications.
-
Tessellations: Rhombuses can be used to create tessellations, which are repeating patterns that cover a plane without gaps or overlaps. This property is utilized in various artistic and design contexts.
-
Kite: A kite is a special case of a quadrilateral where two pairs of adjacent sides are congruent. If a kite has all four sides congruent, then it becomes a rhombus.
Differentiating Rhombuses from Other Parallelograms
It's important to distinguish a rhombus from other parallelograms, particularly rectangles and squares:
-
Rectangle: A rectangle is a parallelogram with four right angles. While a square is both a rhombus and a rectangle, a rhombus is not necessarily a rectangle (unless it also has right angles).
-
Square: A square is a special case of both a rhombus and a rectangle. It possesses all the properties of both, having four congruent sides and four right angles.
The Venn diagram illustrating the relationship between parallelograms, rhombuses, rectangles, and squares effectively summarizes these distinctions. A square is a subset of both rhombuses and rectangles, highlighting its unique status.
Advanced Concepts and Further Exploration
For those interested in further exploration of rhombus geometry, several advanced concepts warrant investigation:
-
Vectors and Rhombuses: Vectors can be used to represent the sides of a rhombus, allowing for a more algebraic approach to analyzing its properties. The dot product can be employed to determine perpendicularity of diagonals.
-
Transformations of Rhombuses: Exploring how transformations, such as rotations, reflections, and translations, affect the properties of a rhombus provides a deeper understanding of its geometric characteristics.
-
Rhombuses in Higher Dimensions: The concept of a rhombus can be extended to higher dimensional spaces, offering a fascinating area of mathematical exploration.
Conclusion
Understanding the conditions for a parallelogram to be a rhombus requires a thorough grasp of its defining characteristics. While inheriting properties from parallelograms, the congruence of all four sides fundamentally distinguishes a rhombus. By mastering the various methods for proving a parallelogram is a rhombus and appreciating its real-world applications, we can fully appreciate the unique position this shape holds within the broader world of geometry. The interplay between its sides, angles, and diagonals offers a rich area of study, with further exploration leading to a deeper understanding of geometric relationships and mathematical principles.
Latest Posts
Latest Posts
-
What Is Square Root Of 65
Mar 31, 2025
-
N 6 N 7 9 2
Mar 31, 2025
-
What Are The Three Parts Of Atp Molecule
Mar 31, 2025
-
What Is The Percentage Of 0 125
Mar 31, 2025
-
How Are Clastic Sedimentary Rocks Classified
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about In Order For The Parallelogram To Be A Rhombus X . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
