How To Integrate 1 X 2 2
listenit
Mar 31, 2025 · 4 min read
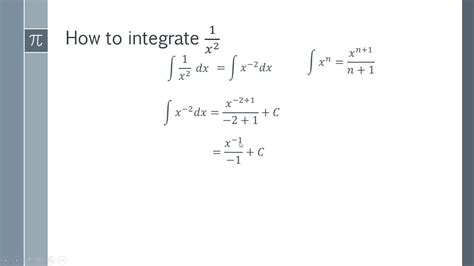
Table of Contents
How to Integrate 1/x^2: A Comprehensive Guide
Integrating functions is a cornerstone of calculus, crucial for solving numerous problems in physics, engineering, and other fields. While many integrals are straightforward, some present unique challenges. One such integral, deceptively simple in appearance, is the definite integral of 1/x². This guide will comprehensively explore this integration, covering various methods, applications, and potential pitfalls.
Understanding the Integral: ∫ 1/x² dx
The integral ∫ 1/x² dx represents the area under the curve of the function f(x) = 1/x² from a lower limit to an upper limit. This function, also written as x⁻², is a power function with a negative exponent. Understanding this property is key to successfully integrating it.
Key Properties of 1/x²:
- Always Positive: For all x ≠ 0, 1/x² is always positive. This means the area under the curve will always be positive.
- Asymptotic Behavior: The function has a vertical asymptote at x = 0. This means the function approaches infinity as x approaches 0. This has implications for definite integrals, as we'll discuss later.
- Inverse Square Relationship: The value of the function decreases rapidly as x increases, following an inverse square relationship.
Method 1: Power Rule of Integration
The most straightforward method for integrating 1/x² is by using the power rule of integration, a fundamental technique in calculus. The power rule states:
∫ xⁿ dx = (xⁿ⁺¹)/(n+1) + C (where n ≠ -1 and C is the constant of integration)
Applying the power rule to our function:
∫ x⁻² dx = (x⁻²⁺¹)/(-2+1) + C = (x⁻¹)/(-1) + C = -1/x + C
Therefore, the indefinite integral of 1/x² is -1/x + C.
Method 2: Recognizing the Derivative
Another approach involves recognizing that 1/x² is the derivative of a known function. Recall that the derivative of -1/x is 1/x². Therefore, the integral is simply the antiderivative:
d/dx (-1/x) = 1/x²
Hence, ∫ 1/x² dx = -1/x + C
Dealing with Definite Integrals
When dealing with definite integrals, we need to evaluate the integral between specific limits. Let's consider the definite integral:
∫ₐᵇ (1/x²) dx
Using the indefinite integral we derived:
[-1/x]ₐᵇ = (-1/b) - (-1/a) = (1/a) - (1/b)
Important Note: This definite integral is only valid if both 'a' and 'b' are non-zero and neither limit includes zero. The presence of the vertical asymptote at x = 0 makes integration across this point impossible. Attempting to integrate across x=0 will result in an undefined or infinite value.
Examples of Definite Integrals
Let's illustrate with a few examples:
Example 1:
∫₁² (1/x²) dx = (-1/2) - (-1/1) = 1/2
Example 2:
∫₂⁵ (1/x²) dx = (-1/5) - (-1/2) = 3/10
Example 3: (Illustrating the problem with the asymptote)
∫₋₁¹ (1/x²) dx is undefined. The function is not integrable across x = 0 because the area under the curve becomes infinite at the asymptote.
Applications of the Integral of 1/x²
The integral of 1/x² finds applications in various scientific and engineering contexts:
-
Newton's Law of Universal Gravitation: The gravitational force between two objects is inversely proportional to the square of the distance between them. Integrals involving 1/x² appear when calculating gravitational potential energy or work done against gravitational forces.
-
Electrostatics: Similar to gravity, electrostatic forces also follow an inverse square law. Integrals of 1/x² are used in calculating electric potential and work done by electric fields.
-
Fluid Dynamics: In certain fluid flow scenarios, the velocity or pressure might have an inverse square relationship with distance. Integrating 1/x² can be useful in analyzing such systems.
-
Probability and Statistics: The integral of 1/x² can appear in probability density functions related to certain distributions.
Common Mistakes to Avoid
-
Forgetting the Constant of Integration (C): Always remember to include the constant of integration 'C' when evaluating indefinite integrals.
-
Improper Handling of the Asymptote: Avoid attempting to integrate across the vertical asymptote at x = 0. The integral will be undefined or infinite in such cases.
-
Incorrect Application of the Power Rule: Double-check your application of the power rule, particularly with negative exponents.
Advanced Considerations: Improper Integrals
If you need to evaluate the integral near the asymptote, you'll need to use the concept of improper integrals. This involves evaluating the integral as a limit:
lim (a→0⁺) ∫ₐᵇ (1/x²) dx
or
lim (b→∞) ∫ₐᵇ (1/x²) dx
These limits may converge to a finite value or diverge to infinity, depending on the integration limits. The evaluation of improper integrals requires a deeper understanding of limit theory.
Conclusion
Integrating 1/x² is a fundamental calculus problem with practical applications across many disciplines. By understanding the power rule, recognizing the derivative, and carefully handling definite and improper integrals, one can confidently solve problems involving this seemingly simple yet powerful function. Remember to always be mindful of the asymptote at x = 0 to avoid errors. This comprehensive guide has equipped you with the knowledge and techniques to tackle these integrals effectively. Practice is key to mastering this integral and solidifying your understanding of calculus principles.
Latest Posts
Latest Posts
-
A Bond In Which Electrons Are Shared Unequally
Apr 01, 2025
-
Greatest Common Factor Of 32 And 36
Apr 01, 2025
-
Least Common Multiple Of 4 And 30
Apr 01, 2025
-
The Principle Of Probability Can Be Used To
Apr 01, 2025
-
Y Varies Jointly As X And Z
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about How To Integrate 1 X 2 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
