How To Find The Mass Of A Planet
listenit
Mar 28, 2025 · 6 min read
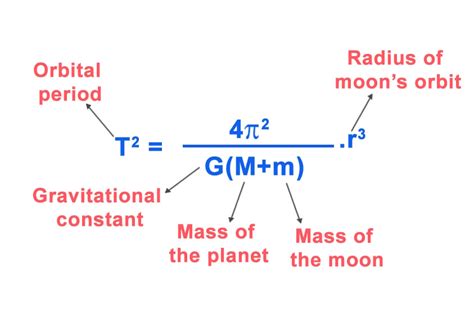
Table of Contents
How to Find the Mass of a Planet: A Comprehensive Guide
Determining the mass of a planet, a seemingly daunting task, is a cornerstone of planetary science. Understanding a planet's mass is crucial for comprehending its formation, evolution, and overall characteristics. This comprehensive guide explores various methods used by astronomers and scientists to achieve this, ranging from simple observations to sophisticated techniques involving complex calculations and space missions.
Understanding Gravitational Influence: The Foundation of Planetary Mass Measurement
The primary method for determining a planet's mass hinges on Newton's Law of Universal Gravitation. This law states that every particle attracts every other particle in the universe with a force which is directly proportional to the product of their masses and inversely proportional to the square of the distance between their centers. Mathematically, this is expressed as:
F = G * (m1 * m2) / r²
Where:
- F represents the gravitational force
- G is the gravitational constant (a fundamental constant in physics)
- m1 and m2 are the masses of the two objects
- r is the distance between the centers of the two objects
This simple yet powerful equation forms the basis for many techniques used to calculate planetary masses. By observing the gravitational effects a planet exerts on other objects, we can deduce its mass.
Methods for Determining Planetary Mass
Several methods exist for measuring a planet's mass, each with its own advantages and limitations depending on the planet's characteristics and our observational capabilities.
1. Using Kepler's Third Law and Orbital Dynamics: For Planets with Moons or Orbiting Objects
Kepler's Third Law of Planetary Motion provides a straightforward method for determining the mass of a planet if it possesses a moon or another object orbiting it. The law states that the square of the orbital period of a planet is directly proportional to the cube of the semi-major axis of its orbit. However, a modified version incorporating Newton's Law of Universal Gravitation allows us to determine the mass:
P² = (4π²/G(M+m)) * a³
Where:
- P is the orbital period of the moon or orbiting object
- a is the semi-major axis of the orbit
- M is the mass of the planet
- m is the mass of the moon or orbiting object
- G is the gravitational constant
This equation is particularly useful because we can measure P and a through astronomical observation. Since the mass of the moon (m) is typically significantly smaller than the planet's mass (M), it can often be neglected in the equation, simplifying the calculation. This method has been extensively used to determine the masses of planets in our solar system with moons, such as Jupiter, Saturn, and Mars.
2. Observing Perturbations in the Orbits of Other Celestial Bodies: For Planets Without Moons
Planets without readily observable moons can still influence the orbits of other celestial bodies, causing slight perturbations. By meticulously tracking these orbital variations, astronomers can infer the mass of the perturbing planet. This method requires precise and long-term observational data, often relying on sophisticated computer models to account for the gravitational influences of multiple bodies. This technique has been applied to detect and characterize exoplanets and to refine the mass estimates of planets in our solar system.
The accuracy of this method relies heavily on the precision of the observations and the sophistication of the computational models used to analyze the data. Small errors in observation can lead to significant uncertainties in the calculated mass.
3. Using Stellar Occultations: A Unique Approach for Exoplanets
Stellar occultations occur when a planet passes in front of its star, causing a slight dimming in the star's light. While this primarily reveals information about the planet's size, the precise timing and nature of the dimming, coupled with other data about the star-planet system, can provide insights into the planet's mass. This approach is particularly useful for exoplanets, and its effectiveness is enhanced when combined with radial velocity measurements (explained below).
This method works best for transiting exoplanets, which are those whose orbits pass directly between their star and our line of sight. The information gained helps constrain the planet's density, providing clues to its composition and potentially offering a more accurate mass estimate when combined with other techniques.
4. Radial Velocity Method: Measuring the "Wobble" of a Star
The radial velocity method is widely used in exoplanet detection. A planet orbiting a star exerts a gravitational pull on the star, causing the star to "wobble" slightly in its position. This wobble can be detected by observing the Doppler shift in the star's light. By analyzing the amplitude and period of the wobble, astronomers can determine the planet's mass. However, it's important to note that this method provides the minimum mass of the planet, as the inclination of the planet's orbit affects the observed wobble.
The accuracy of this method depends on the precision of the spectroscopic measurements and the knowledge of the star's mass and distance. It's most effective for detecting massive planets close to their stars, as these planets induce larger wobbles that are easier to measure.
5. Combining Methods for Enhanced Accuracy: The Power of Synergy
Often, the most accurate estimates of planetary mass are obtained by combining multiple techniques. For instance, combining radial velocity measurements with transit observations can significantly improve the precision of the mass determination, allowing for a more comprehensive understanding of the planet's properties. This synergistic approach leverages the strengths of individual methods while mitigating their limitations, leading to refined and more reliable mass estimates.
Challenges and Limitations in Measuring Planetary Mass
While significant advancements have been made in measuring planetary masses, several challenges remain:
-
Distance: The vast distances to many planets make precise observations challenging. The further away a planet is, the weaker its gravitational influence, making it harder to detect and measure.
-
Orbital Inclination: The angle of a planet's orbit relative to our line of sight influences the observed gravitational effects. An edge-on orbit will yield different results compared to a face-on orbit. This is particularly relevant for the radial velocity method.
-
Multiple Body Interactions: In many systems, multiple celestial bodies interact gravitationally, making it complex to isolate the gravitational influence of a single planet and accurately calculate its mass.
-
Observational Errors: Even with advanced telescopes and instruments, observational errors are inevitable. These errors can propagate through the calculations, leading to uncertainties in the final mass estimate.
The Future of Planetary Mass Measurement
Ongoing advancements in observational technology and computational techniques continue to improve our ability to measure planetary masses with greater precision. Future missions, such as advanced space-based telescopes and dedicated exoplanet-hunting missions, promise to revolutionize our understanding of planetary systems beyond our solar system. The combination of sophisticated instrumentation with increasingly powerful computational tools will help overcome some of the current limitations, leading to more accurate and comprehensive characterization of planets across the universe. This, in turn, will significantly enhance our understanding of planetary formation, evolution, and the diversity of planetary systems within our galaxy and beyond.
The quest to accurately determine planetary masses is a continuous endeavor. The techniques described in this guide represent the culmination of centuries of scientific progress, and future innovations will undoubtedly refine our methods further, providing a more detailed and comprehensive picture of the universe's planetary population.
Latest Posts
Latest Posts
-
What Is The Gcf Of 42 And 24
Mar 31, 2025
-
The Least Common Multiple Of 6 And 9
Mar 31, 2025
-
6 1 2 As An Improper Fraction
Mar 31, 2025
-
How Do Sedimentary Rocks Change Into Igneous Rocks
Mar 31, 2025
-
The Sum Of 3 Consecutive Integers
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about How To Find The Mass Of A Planet . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
