How To Convert A Square Root Into A Decimal
listenit
Mar 29, 2025 · 5 min read
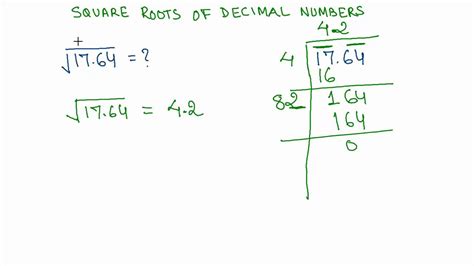
Table of Contents
How to Convert a Square Root into a Decimal: A Comprehensive Guide
Converting a square root into a decimal involves finding the number that, when multiplied by itself, equals the number under the square root symbol (√). While some square roots result in whole numbers (e.g., √9 = 3), most produce irrational numbers—numbers with decimal representations that continue infinitely without repeating. This guide explores various methods for converting square roots into decimals, catering to different levels of mathematical proficiency and technological access.
Understanding Square Roots and Decimals
Before diving into the conversion methods, let's solidify our understanding of the core concepts.
What is a Square Root?
A square root is a number that, when multiplied by itself, gives a specific number. For example, the square root of 25 (√25) is 5 because 5 x 5 = 25. The number under the square root symbol is called the radicand.
What are Decimals?
Decimals are a way of representing numbers that are not whole numbers. They use a decimal point to separate the whole number part from the fractional part. For instance, 3.14 is a decimal number, representing three and fourteen hundredths.
Irrational Numbers and Square Roots
Many square roots, especially those of non-perfect squares (numbers that are not the product of an integer multiplied by itself), result in irrational numbers. Irrational numbers have decimal representations that are non-terminating and non-repeating. This means the decimal goes on forever without ever settling into a repeating pattern. Examples include √2, √3, and √5.
Methods for Converting Square Roots to Decimals
There are several ways to convert square roots to decimals, ranging from manual calculations to using technology. Let's examine the most common approaches:
1. Using a Calculator
The simplest and most efficient method for converting square roots into decimals is using a calculator. Most scientific calculators have a dedicated square root button (√). Simply enter the number under the square root and press the square root button. The calculator will display the decimal approximation.
For instance, to find the decimal representation of √16, input 16 and press the √ button. The calculator will display 4. For √2, the calculator will give an approximation such as 1.41421356. Keep in mind that this is an approximation; the actual decimal representation of √2 is infinitely long.
Advantages: Quick, accurate (to the limits of the calculator's precision), and readily accessible.
Disadvantages: Relies on having a calculator available. Doesn't provide an understanding of the underlying mathematical processes.
2. Manual Estimation and Approximation
For a deeper understanding or when a calculator isn't available, manual estimation and approximation techniques can be employed. This involves identifying perfect squares close to the radicand and using those as a starting point.
Example: Let's approximate √10.
- We know that 3 x 3 = 9 and 4 x 4 = 16. Therefore, √10 lies between 3 and 4.
- Since 10 is closer to 9 than to 16, we can estimate √10 to be slightly more than 3.
- More sophisticated techniques involve using iterative methods like the Babylonian method (also known as Heron's method), which refines the estimate in successive steps.
Advantages: Builds understanding of the mathematical principles.
Disadvantages: Time-consuming, particularly for complex square roots, and prone to error depending on the accuracy of the estimation.
3. Long Division Method (for Perfect Squares)
While not commonly used for irrational square roots, the long division method can be applied to find the decimal representation of perfect squares. This method is essentially a systematic way of finding the square root through repeated subtraction and division. It's a bit complex and generally not as practical as using a calculator for this purpose.
Advantages: Provides a step-by-step understanding of finding square roots.
Disadvantages: Tedious and impractical for most situations, especially for non-perfect squares.
4. Using Logarithms
Logarithms offer a mathematical approach for calculating square roots, albeit a more advanced method. The formula involved is based on the property of logarithms that log(√x) = ½log(x). This means you can find the logarithm of the radicand, divide it by 2, and then find the antilogarithm to obtain the square root.
Advantages: Provides a different mathematical perspective.
Disadvantages: Requires a strong understanding of logarithms and access to logarithm tables or a calculator with logarithmic functions. It's not the most straightforward method for simple square root calculations.
5. Numerical Methods (for Advanced Users)
For those with a strong mathematical background, numerical methods like the Newton-Raphson method provide a powerful tool for approximating square roots to a high degree of accuracy. These methods involve iterative algorithms that refine an initial guess to converge on the true value. These methods are often used in computer programming to calculate square roots efficiently.
Advantages: High accuracy and efficient for complex calculations.
Disadvantages: Requires advanced mathematical knowledge and programming skills.
Tips for Working with Square Roots and Decimals
- Understand perfect squares: Familiarize yourself with common perfect squares (1, 4, 9, 16, 25, 36, etc.) to aid in estimation.
- Use online calculators: Numerous online calculators provide square root calculations, offering an easy alternative to a physical calculator.
- Practice regularly: The more you practice calculating square roots, the better you'll become at estimating and using different methods.
- Learn about significant figures: When working with decimal approximations, pay attention to the concept of significant figures to represent the accuracy of your result.
Conclusion
Converting square roots to decimals is a fundamental mathematical skill. While using a calculator provides the quickest and most accurate result, understanding the underlying principles and alternative methods enhances your mathematical understanding and problem-solving capabilities. Choose the method that best suits your needs and level of mathematical expertise, remembering that even seemingly simple calculations can reveal deeper mathematical concepts. With practice and exploration of different techniques, you will master the conversion of square roots to decimals with confidence.
Latest Posts
Latest Posts
-
What Is The Inverse Of 3 X
Apr 01, 2025
-
Write 2 5 As A Decimal
Apr 01, 2025
-
Is Earth Older Than The Sun
Apr 01, 2025
-
2 Times Square Root Of 5
Apr 01, 2025
-
The Change Of State From Liquid To Gas Is Called
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about How To Convert A Square Root Into A Decimal . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
