How Many Revolutions Are In A Radian
listenit
Apr 02, 2025 · 5 min read
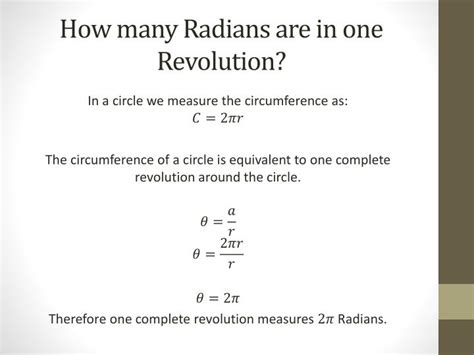
Table of Contents
How Many Revolutions Are in a Radian? Unraveling the Relationship Between Revolutions, Radians, and Degrees
Understanding the relationship between revolutions, radians, and degrees is fundamental to grasping many concepts in mathematics, physics, and engineering. While degrees are a familiar unit for measuring angles, radians are often preferred in higher-level mathematics and science due to their inherent mathematical properties. This article will delve deep into the question: how many revolutions are in a radian? We'll explore the concepts of revolutions, radians, and degrees, highlighting their interconnections and providing practical examples to solidify your understanding.
Understanding Revolutions
A revolution, often shortened to "rev," represents a complete circular rotation. Imagine a point on the circumference of a circle tracing its path back to its starting position. That single complete cycle constitutes one revolution. It's a simple, intuitive concept used frequently in everyday life, from describing the rotation of a wheel to the spinning of a record.
Degrees: A Familiar Measurement of Angles
Degrees are a common unit for measuring angles. A complete circle is divided into 360 degrees (360°). This system, inherited from Babylonian mathematics, is widely used in everyday applications and introductory geometry. Dividing a circle into 360 degrees is arbitrary, but its widespread adoption makes it a convenient standard for many applications.
Radians: The Natural Unit for Angles
Radians, unlike degrees, possess a more fundamental mathematical basis. A radian is defined as the angle subtended at the center of a circle by an arc equal in length to the radius of the circle. This definition directly links the angle measure to the circle's geometry. This seemingly simple definition leads to elegant mathematical properties that make radians the preferred unit in calculus, trigonometry, and many scientific fields.
Visualizing a Radian
Imagine a circle with radius r. Now, consider an arc along the circle's circumference that also has length r. The angle subtended at the center of the circle by this arc is exactly one radian. Since the circumference of a circle is 2πr, there are 2π radians in a full circle (360°).
The Relationship Between Revolutions, Radians, and Degrees
The key to understanding the relationship lies in the fundamental equivalence:
- 1 revolution = 360 degrees = 2π radians
This equation provides the cornerstone for converting between these units. Let's break down the conversions:
Converting Revolutions to Radians
To convert revolutions to radians, you simply multiply the number of revolutions by 2π:
Radians = Revolutions × 2π
For instance, 2 revolutions are equal to 2 × 2π = 4π radians.
Converting Radians to Revolutions
To convert radians to revolutions, divide the number of radians by 2π:
Revolutions = Radians / 2π
For example, 6π radians are equal to 6π / 2π = 3 revolutions.
Converting Degrees to Radians
To convert degrees to radians, use the following formula:
Radians = Degrees × (π/180)
For instance, 90 degrees are equivalent to 90 × (π/180) = π/2 radians.
Converting Radians to Degrees
To convert radians to degrees, use the following formula:
Degrees = Radians × (180/π)
For example, π/4 radians are equal to (π/4) × (180/π) = 45 degrees.
How Many Revolutions Are in One Radian?
Now, we can directly address the central question: how many revolutions are in a radian? Since 2π radians equal one revolution, we can calculate the number of revolutions in one radian by dividing one by 2π:
Revolutions per Radian = 1 / 2π ≈ 0.159
Therefore, there are approximately 0.159 revolutions in one radian. This means that one radian represents a fraction of a complete circle.
Practical Applications and Examples
The understanding of revolutions, radians, and degrees is crucial in various fields:
1. Circular Motion in Physics
In physics, radians are the preferred unit for describing angular velocity, angular acceleration, and other aspects of rotational motion. Using radians simplifies the mathematical expressions involved in these calculations.
Example: A wheel rotates at an angular velocity of 10 radians per second. To find the number of revolutions per second, we divide 10 by 2π, obtaining approximately 1.59 revolutions per second.
2. Trigonometry and Calculus
In trigonometry and calculus, using radians significantly simplifies the formulas and derivations of trigonometric functions. For example, the derivative of sin(x) is cos(x) only when x is measured in radians.
Example: Finding the area under a sine curve requires integration, and the formula simplifies considerably when using radians.
3. Engineering and Robotics
In engineering and robotics, understanding angular measurements is essential for designing and controlling rotating machinery, such as motors, gears, and robotic arms. Radians are often used in control systems for precise angular positioning.
Example: A robotic arm needs to rotate through a specific angle to pick up an object. Using radians ensures accurate positioning, leading to improved efficiency and precision.
Conclusion: Mastering the Units of Angular Measurement
Mastering the conversion between revolutions, radians, and degrees is essential for anyone working with circular motion, trigonometry, or other related fields. While degrees are readily understood in everyday contexts, radians provide a more natural and mathematically elegant framework for more advanced applications. Understanding the relationship between these units – that one revolution equals 2π radians – unlocks a deeper comprehension of the fundamental concepts underlying circular motion and angular measurements. Remember the core conversion formulas, practice applying them to various problems, and you'll be well on your way to confidently tackling challenges involving angles and rotations. This comprehensive understanding will prove invaluable as you progress in your studies and work in fields that involve circular motion and angular measurements. The seemingly simple question of "how many revolutions are in a radian?" opens the door to a vast array of mathematical and scientific concepts.
Latest Posts
Latest Posts
-
Two Lines Intersecting At A Right Angle
Apr 03, 2025
-
What Is 7 Out Of 15 As A Percentage
Apr 03, 2025
-
Which Kingdom Contains Heterotrophs With Cell Walls Of Chitin
Apr 03, 2025
-
In A Solution The Solvent Is
Apr 03, 2025
-
1 4 As An Improper Fraction
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about How Many Revolutions Are In A Radian . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
