1 4 As An Improper Fraction
listenit
Apr 03, 2025 · 5 min read
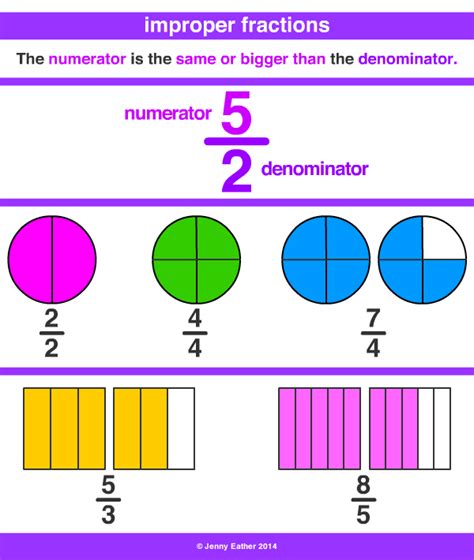
Table of Contents
- 1 4 As An Improper Fraction
- Table of Contents
- 1 4 as an Improper Fraction: A Comprehensive Guide
- Understanding Mixed Numbers and Improper Fractions
- Converting 1 ⁴⁄₅ to an Improper Fraction: The Step-by-Step Process
- Visualizing the Conversion: A Geometric Approach
- Practical Applications of Improper Fractions
- Converting Improper Fractions Back to Mixed Numbers
- Solving Problems Involving Improper Fractions
- Beyond the Basics: Advanced Concepts
- Conclusion
- Latest Posts
- Latest Posts
- Related Post
1 4 as an Improper Fraction: A Comprehensive Guide
Understanding fractions is fundamental to mathematics, and mastering the conversion between mixed numbers and improper fractions is a crucial skill. This comprehensive guide delves into the intricacies of representing the mixed number 1 ⁴⁄₅ as an improper fraction, exploring the underlying concepts and providing practical examples to solidify your understanding. We'll also touch upon the broader applications of improper fractions in various mathematical contexts.
Understanding Mixed Numbers and Improper Fractions
Before diving into the conversion process, let's clarify the definitions of mixed numbers and improper fractions.
Mixed Numbers: A mixed number combines a whole number and a proper fraction. A proper fraction has a numerator (top number) smaller than its denominator (bottom number). For example, 1 ⁴⁄₅ is a mixed number where 1 is the whole number and ⁴⁄₅ is the proper fraction.
Improper Fractions: An improper fraction has a numerator greater than or equal to its denominator. For example, ⁹⁄₅ is an improper fraction because the numerator (9) is larger than the denominator (5). Improper fractions represent values greater than or equal to one.
Converting 1 ⁴⁄₅ to an Improper Fraction: The Step-by-Step Process
The conversion of a mixed number to an improper fraction involves two key steps:
Step 1: Multiply the whole number by the denominator of the fraction.
In our example, 1 ⁴⁄₅, the whole number is 1 and the denominator is 5. Therefore, we perform the multiplication: 1 * 5 = 5.
Step 2: Add the result from Step 1 to the numerator of the fraction.
We take the result from Step 1 (5) and add it to the numerator of the fraction (4): 5 + 4 = 9.
Step 3: Keep the same denominator.
The denominator of the improper fraction remains the same as the denominator of the original proper fraction. In this case, the denominator remains 5.
Step 4: Combine the results to form the improper fraction.
Combining the results from Step 2 (9) and Step 3 (5), we get the improper fraction: ⁹⁄₅.
Therefore, the mixed number 1 ⁴⁄₅ is equivalent to the improper fraction ⁹⁄₅.
Visualizing the Conversion: A Geometric Approach
Imagine a pizza cut into 5 equal slices. The mixed number 1 ⁴⁄₅ represents one whole pizza (5 slices) plus ⁴⁄₅ of another pizza (4 slices). In total, you have 9 slices (5 + 4 = 9). Since each slice represents ⅕ of a pizza, the total number of slices (9) becomes the numerator, and the number of slices in a whole pizza (5) remains the denominator, giving us the improper fraction ⁹⁄₅.
Practical Applications of Improper Fractions
Improper fractions are not simply theoretical constructs; they have numerous practical applications across various fields:
-
Cooking and Baking: Recipes often require fractional amounts of ingredients. Using improper fractions simplifies calculations, particularly when dealing with larger quantities. For instance, a recipe might call for ⁹⁄₅ cups of flour, which is easier to measure than 1 ⁴⁄₅ cups.
-
Construction and Engineering: Precise measurements are paramount in construction and engineering. Improper fractions provide a more accurate representation of measurements than mixed numbers, minimizing errors in calculations.
-
Finance and Accounting: Improper fractions can streamline calculations involving shares of stock or portions of financial instruments.
-
Computer Programming: Many programming languages utilize fractions for representing data or performing calculations, and improper fractions offer a more efficient representation for some operations.
-
Science and Physics: In scientific and physics calculations, improper fractions can be more suitable for certain calculations and simulations.
Converting Improper Fractions Back to Mixed Numbers
While converting mixed numbers to improper fractions is crucial, it's equally important to understand the reverse process. Let's convert ⁹⁄₅ back to a mixed number:
Step 1: Divide the numerator by the denominator.
Divide 9 by 5: 9 ÷ 5 = 1 with a remainder of 4.
Step 2: The quotient becomes the whole number.
The quotient (1) becomes the whole number of the mixed number.
Step 3: The remainder becomes the numerator of the fraction.
The remainder (4) becomes the numerator of the fraction.
Step 4: The denominator remains the same.
The denominator remains 5.
Step 5: Combine the whole number and the fraction.
Combining the whole number (1) and the fraction (⁴⁄₅), we get the mixed number 1 ⁴⁄₅.
Solving Problems Involving Improper Fractions
Let's consider a couple of examples to solidify your understanding:
Example 1: Add the mixed numbers 2 ¾ and 1 ⅛.
First, convert both mixed numbers to improper fractions:
2 ¾ = (2 * 4 + 3) / 4 = 11/4
1 ⅛ = (1 * 8 + 1) / 8 = 9/8
Now add the improper fractions:
11/4 + 9/8 = (22 + 9) / 8 = 31/8
Finally, convert the result back to a mixed number:
31 ÷ 8 = 3 with a remainder of 7. Therefore, the answer is 3 ⅞.
Example 2: Multiply the improper fraction ⁷⁄₃ by the mixed number 2 ½.
First, convert the mixed number to an improper fraction:
2 ½ = (2 * 2 + 1) / 2 = 5/2
Now multiply the improper fractions:
⁷⁄₃ * ⁵⁄₂ = 35/6
Finally, convert the result back to a mixed number:
35 ÷ 6 = 5 with a remainder of 5. Therefore, the answer is 5 ⁵⁄₆.
Beyond the Basics: Advanced Concepts
The conversion between mixed numbers and improper fractions forms the foundation for more complex mathematical operations involving fractions. Understanding this fundamental concept is crucial for tackling more advanced topics such as:
-
Fraction addition and subtraction: Finding a common denominator is easier when working with improper fractions.
-
Fraction multiplication and division: Improper fractions often simplify calculations.
-
Solving algebraic equations: Improper fractions can be a more convenient form in algebraic expressions.
-
Calculus: The concept extends to more complex mathematical operations in calculus.
Conclusion
Mastering the conversion between mixed numbers and improper fractions is essential for anyone seeking a deeper understanding of mathematics. This comprehensive guide has provided a thorough explanation of the process, illustrated with practical examples and real-world applications. By understanding these concepts, you can confidently tackle more complex mathematical problems and apply your knowledge across various fields. Remember to practice regularly to solidify your understanding and build confidence in working with fractions. The more you practice, the easier and more intuitive this process will become.
Latest Posts
Latest Posts
-
Why Were True Breeding Pea Plants Important For Mendels Experiment
Apr 06, 2025
-
How Many Commas Can You Use In A Sentence
Apr 06, 2025
-
What Element Has 7 Protons 8 Neutrons And 10 Electrons
Apr 06, 2025
-
How To Tell If A Molecule Is Planar
Apr 06, 2025
-
Which Kingdom S Include Organisms That Are Autotrophic Or Heterotrophic
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about 1 4 As An Improper Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
