Common Factors Of 8 And 24
listenit
Apr 05, 2025 · 5 min read
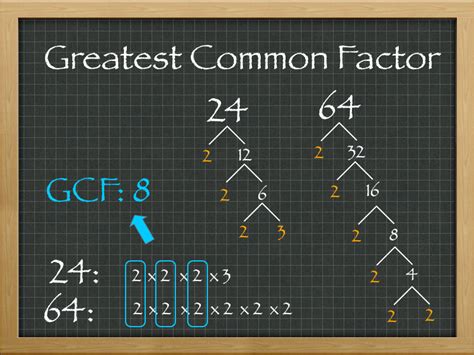
Table of Contents
Unraveling the Common Factors of 8 and 24: A Deep Dive into Number Theory
Finding the common factors of two numbers might seem like a simple arithmetic task, but it's a fundamental concept in number theory with applications far beyond basic calculations. This comprehensive guide delves into the common factors of 8 and 24, explaining the process, exploring related concepts, and demonstrating the practical significance of this seemingly straightforward mathematical operation.
Understanding Factors and Common Factors
Before we delve into the specifics of 8 and 24, let's establish a clear understanding of the terminology.
Factors: A factor of a number is a whole number that divides evenly into that number without leaving a remainder. For example, the factors of 8 are 1, 2, 4, and 8. Each of these numbers divides perfectly into 8.
Common Factors: Common factors are the numbers that are factors of two or more numbers. They are the numbers that divide evenly into each of the given numbers.
Finding the Common Factors of 8 and 24: A Step-by-Step Approach
Several methods can be employed to identify the common factors of 8 and 24. Here are two common approaches:
1. Listing Factors: A Simple, Intuitive Method
This method involves listing all factors of each number individually and then identifying the numbers that appear in both lists.
Factors of 8: 1, 2, 4, 8
Factors of 24: 1, 2, 3, 4, 6, 8, 12, 24
Common Factors: Comparing the two lists, we see that 1, 2, 4, and 8 are present in both. Therefore, the common factors of 8 and 24 are 1, 2, 4, and 8.
2. Prime Factorization: A More Systematic Approach
Prime factorization is a powerful technique in number theory. It involves expressing a number as the product of its prime factors (numbers divisible only by 1 and themselves). This method is particularly useful when dealing with larger numbers.
-
Prime Factorization of 8: 2 x 2 x 2 = 2³
-
Prime Factorization of 24: 2 x 2 x 2 x 3 = 2³ x 3
To find the common factors, we identify the prime factors that are common to both numbers and their lowest powers. In this case, both 8 and 24 share three factors of 2 (2³).
Common Factors from Prime Factorization: Using the lowest power of the common prime factor (2³), we can derive the common factors:
- 2⁰ = 1
- 2¹ = 2
- 2² = 4
- 2³ = 8
Therefore, the common factors are 1, 2, 4, and 8.
Greatest Common Factor (GCF) and Least Common Multiple (LCM)
The process of finding common factors is closely related to finding the Greatest Common Factor (GCF) and the Least Common Multiple (LCM).
Greatest Common Factor (GCF): The GCF is the largest number that divides evenly into two or more numbers. In the case of 8 and 24, the GCF is 8.
Least Common Multiple (LCM): The LCM is the smallest number that is a multiple of two or more numbers. To find the LCM of 8 and 24, we can use the prime factorization method:
- Prime Factorization of 8: 2³
- Prime Factorization of 24: 2³ x 3
The LCM is found by taking the highest power of each prime factor present in either factorization: 2³ x 3 = 24. Therefore, the LCM of 8 and 24 is 24.
Applications of Common Factors and GCF
The concept of common factors, particularly the GCF, finds applications in various areas:
-
Simplifying Fractions: Finding the GCF allows us to simplify fractions to their lowest terms. For example, the fraction 24/8 can be simplified by dividing both the numerator and the denominator by their GCF (8), resulting in the simplified fraction 3/1 or 3.
-
Solving Word Problems: Many word problems involving quantities that need to be divided equally utilize the concept of GCF. For instance, if you have 24 apples and 8 oranges and want to create identical gift bags with the same number of apples and oranges in each, you would use the GCF to determine the maximum number of identical gift bags possible (8 bags).
-
Geometry and Measurement: The GCF is crucial in determining the dimensions of the largest square tile that can be used to cover a rectangular area without any cuts or gaps. For example, if you have a rectangular area measuring 24 meters by 8 meters, the largest square tile you could use without cutting would be 8 meters by 8 meters.
-
Algebra and Equation Solving: The GCF is frequently used in simplifying algebraic expressions and solving equations.
Beyond the Basics: Exploring Related Concepts
The study of factors and common factors opens doors to more advanced concepts in number theory:
-
Euclidean Algorithm: This efficient algorithm is used to find the GCF of two or more numbers, especially when dealing with large numbers where listing factors might be impractical.
-
Modular Arithmetic: This branch of number theory deals with remainders after division, and the concept of factors plays a vital role in understanding congruences and related theorems.
-
Diophantine Equations: These are equations where only integer solutions are sought. The GCF is frequently used to determine whether a Diophantine equation has solutions and to find those solutions.
-
Cryptography: Number theory, including concepts like prime factorization and GCF, forms the bedrock of modern cryptography, securing digital communications and data.
Conclusion: The Significance of Common Factors
While finding the common factors of 8 and 24 may appear elementary, it underscores fundamental principles in number theory that have far-reaching implications. The ability to efficiently identify common factors, calculate the GCF and LCM, and understand their applications is crucial for success in various mathematical fields and problem-solving scenarios. Mastering these concepts provides a solid foundation for exploring more advanced mathematical ideas and their real-world applications. From simplifying fractions to solving complex equations and securing digital communications, the seemingly simple act of finding common factors reveals the beauty and power of mathematical reasoning. This deep dive into the common factors of 8 and 24 serves as a springboard to further exploration of this fascinating area of mathematics. The ability to unravel these seemingly simple numerical relationships unlocks a deeper understanding of the underlying structure and elegance of the mathematical world.
Latest Posts
Latest Posts
-
20 Is What Percent Of 400
Apr 05, 2025
-
How Many Protons Are In A Sulfur Atom
Apr 05, 2025
-
What Is The Square Root Of 2 25
Apr 05, 2025
-
What Is The Least Common Multiple Of 9 And 3
Apr 05, 2025
-
The Horizontal Columns On The Periodic Table Are Called
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about Common Factors Of 8 And 24 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
