Common Factors Of 28 And 42
listenit
Mar 29, 2025 · 6 min read
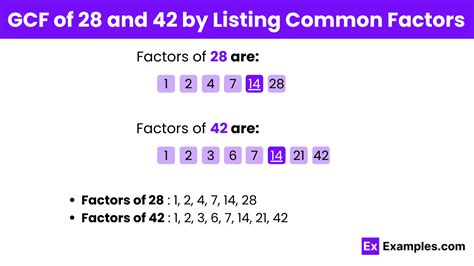
Table of Contents
Unveiling the Secrets of Common Factors: A Deep Dive into 28 and 42
Finding the common factors of two numbers might seem like a simple mathematical exercise, but it unlocks a deeper understanding of number theory and its applications. This article will delve into the fascinating world of common factors, specifically exploring the common factors of 28 and 42. We'll go beyond simply listing the factors and uncover the underlying principles and techniques, illustrating their relevance in various mathematical contexts.
Understanding Factors and Common Factors
Before we dive into the specifics of 28 and 42, let's establish a clear understanding of fundamental concepts.
What are Factors?
Factors, also known as divisors, are whole numbers that divide evenly into a given number without leaving a remainder. For instance, the factors of 12 are 1, 2, 3, 4, 6, and 12, because each of these numbers divides 12 without leaving any remainder.
Identifying Factors
There are several ways to identify the factors of a number:
- Listing Method: This involves systematically checking each whole number from 1 up to the number itself to see if it divides evenly. This method is straightforward but can be time-consuming for larger numbers.
- Prime Factorization: Breaking down a number into its prime factors (numbers divisible only by 1 and themselves) provides a powerful way to find all its factors. We'll explore this method in more detail later.
- Factor Pairs: Factors often come in pairs. For example, in the factors of 12 (1, 2, 3, 4, 6, 12), we see pairs like (1, 12), (2, 6), and (3, 4). This pairing can streamline the process of finding all factors.
What are Common Factors?
Common factors are numbers that are factors of two or more numbers. They represent the values that divide all the numbers in question without leaving a remainder.
Finding Common Factors: A Step-by-Step Approach
Let's apply these concepts to find the common factors of 28 and 42.
-
Find the Factors of 28: The factors of 28 are 1, 2, 4, 7, 14, and 28.
-
Find the Factors of 42: The factors of 42 are 1, 2, 3, 6, 7, 14, 21, and 42.
-
Identify the Common Factors: By comparing the lists, we find the common factors of 28 and 42 are 1, 2, 7, and 14.
Therefore, the common factors of 28 and 42 are 1, 2, 7, and 14.
Exploring Prime Factorization: A More Efficient Method
Prime factorization offers a more elegant and efficient approach to finding common factors, especially for larger numbers.
What is Prime Factorization?
Prime factorization is the process of expressing a number as a product of its prime factors. Every composite number (a number greater than 1 that is not prime) can be uniquely expressed as a product of prime numbers.
Prime Factorization of 28
To find the prime factorization of 28, we can use a factor tree:
28 = 2 x 14 14 = 2 x 7
Therefore, the prime factorization of 28 is 2² x 7.
Prime Factorization of 42
Similarly, the prime factorization of 42 is:
42 = 2 x 21 21 = 3 x 7
Therefore, the prime factorization of 42 is 2 x 3 x 7.
Finding Common Factors using Prime Factorization
Once we have the prime factorizations, finding the common factors becomes straightforward:
-
Identify Common Prime Factors: Both 28 (2² x 7) and 42 (2 x 3 x 7) share the prime factors 2 and 7.
-
Construct Common Factors: To find the common factors, we combine the common prime factors in all possible ways:
- 2¹ = 2
- 7¹ = 7
- 2¹ x 7¹ = 14
- 2⁰ x 7⁰ = 1 (Remember that any number to the power of 0 is 1)
This method confirms our earlier findings: the common factors of 28 and 42 are 1, 2, 7, and 14.
The Greatest Common Factor (GCF)
Among the common factors, the greatest one holds special significance. This is known as the Greatest Common Factor (GCF), also called the Highest Common Factor (HCF). In the case of 28 and 42, the GCF is 14.
Finding the GCF using Prime Factorization
The prime factorization method makes finding the GCF particularly easy:
-
Find the Prime Factorizations: As we've already done, find the prime factorizations of 28 (2² x 7) and 42 (2 x 3 x 7).
-
Identify Common Prime Factors: The common prime factors are 2 and 7.
-
Calculate the GCF: To find the GCF, take the lowest power of each common prime factor and multiply them together. In this case, the lowest power of 2 is 2¹ and the lowest power of 7 is 7¹. Therefore, the GCF is 2¹ x 7¹ = 14.
Importance of the GCF
The GCF has several important applications in mathematics and beyond:
- Simplifying Fractions: The GCF is crucial for simplifying fractions to their lowest terms.
- Solving Problems Involving Ratios and Proportions: GCF helps simplify ratios and proportions.
- Geometry: The GCF is used in problems involving area and perimeter calculations.
- Real-world Applications: GCF plays a role in various real-world situations, such as dividing objects or resources evenly.
Least Common Multiple (LCM) and its Relationship with GCF
While we've focused on GCF, it's important to understand its relationship with the Least Common Multiple (LCM). The LCM is the smallest number that is a multiple of both numbers.
Finding the LCM of 28 and 42
We can find the LCM using the prime factorizations:
-
Prime Factorizations: 28 = 2² x 7 and 42 = 2 x 3 x 7
-
Identify All Prime Factors: The unique prime factors are 2, 3, and 7.
-
Calculate the LCM: Take the highest power of each prime factor and multiply them: 2² x 3 x 7 = 84.
Therefore, the LCM of 28 and 42 is 84.
The Relationship between GCF and LCM
The GCF and LCM are inversely related. Their product is always equal to the product of the original two numbers:
GCF(a, b) x LCM(a, b) = a x b
Let's verify this for 28 and 42:
GCF(28, 42) x LCM(28, 42) = 14 x 84 = 1176 28 x 42 = 1176
The equation holds true, demonstrating the elegant relationship between the GCF and LCM.
Applications and Further Exploration
The concepts of factors, common factors, GCF, and LCM extend far beyond basic arithmetic. They form the foundation for many advanced mathematical concepts and have practical applications in various fields:
- Cryptography: Prime factorization plays a crucial role in modern cryptography, forming the basis of many encryption algorithms.
- Computer Science: GCF and LCM are used in algorithms related to scheduling and resource allocation.
- Music Theory: Understanding factors and multiples is essential in music theory for understanding intervals and harmonies.
This exploration of the common factors of 28 and 42 provides a foundation for deeper understanding of number theory. By mastering these fundamental concepts, one can unlock a wealth of mathematical knowledge and its wide-ranging applications. Further exploration could involve investigating Euclid's algorithm for finding the GCF, exploring the properties of prime numbers, or delving into the fascinating world of modular arithmetic. The journey into the world of numbers is endless, and understanding common factors serves as a crucial stepping stone.
Latest Posts
Latest Posts
-
What Is The Gcf Of 27 And 45
Apr 01, 2025
-
Compounds Containing Only Carbon And Hydrogen Are Called
Apr 01, 2025
-
What Is The Percent Of 5 12
Apr 01, 2025
-
How Many Quarts Is 1 L
Apr 01, 2025
-
Sketch The Region Enclosed By The Given Curves
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about Common Factors Of 28 And 42 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
