What Is The Percent Of 5/12
listenit
Apr 01, 2025 · 5 min read
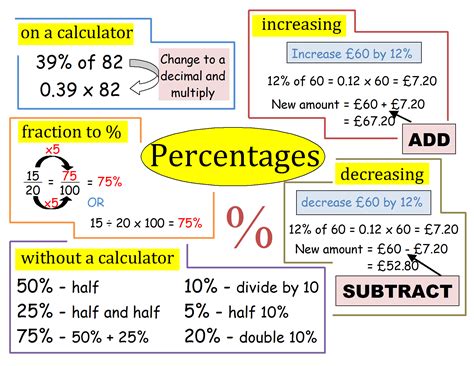
Table of Contents
What is the Percentage of 5/12? A Comprehensive Guide to Fraction-to-Percentage Conversion
Understanding how to convert fractions to percentages is a fundamental skill in mathematics with widespread applications in various fields, from everyday budgeting to advanced statistical analysis. This comprehensive guide will explore the process of converting the fraction 5/12 into a percentage, providing a detailed explanation and illustrating various methods to achieve this conversion. We’ll also delve into the broader context of fraction-to-percentage conversions, highlighting practical examples and offering tips for mastering this essential mathematical skill.
Understanding Fractions and Percentages
Before we delve into the specific conversion of 5/12, let's establish a clear understanding of fractions and percentages.
Fractions: A fraction represents a part of a whole. It consists of a numerator (the top number) and a denominator (the bottom number). The numerator indicates how many parts are being considered, while the denominator indicates the total number of equal parts the whole is divided into. For example, in the fraction 5/12, 5 is the numerator and 12 is the denominator. This means we're considering 5 parts out of a total of 12 equal parts.
Percentages: A percentage represents a fraction of 100. It indicates how many parts out of 100 are being considered. The symbol "%" is used to denote percentages. For example, 25% means 25 parts out of 100, which is equivalent to the fraction 25/100.
Converting 5/12 to a Percentage: The Primary Method
The most straightforward method to convert a fraction to a percentage involves two simple steps:
-
Convert the fraction to a decimal: To do this, divide the numerator (5) by the denominator (12). 5 ÷ 12 ≈ 0.4166666...
-
Convert the decimal to a percentage: Multiply the decimal by 100 and add the "%" symbol. 0.4166666... × 100 ≈ 41.67%
Therefore, 5/12 is approximately equal to 41.67%. Note that we've rounded the result to two decimal places for practicality. The exact decimal representation of 5/12 is a repeating decimal (0.416666...), so rounding is necessary for most practical applications.
Alternative Methods for Conversion
While the primary method is the most straightforward, let's explore some alternative approaches:
Method 1: Using Equivalent Fractions
This method involves finding an equivalent fraction with a denominator of 100. However, this isn't always easily achievable, especially with fractions like 5/12 that don't have a simple relationship with 100. While we can't easily find an equivalent fraction with a denominator of 100, understanding this principle reinforces the core concept of fraction equivalence.
Method 2: Using Proportions
We can set up a proportion to solve for the percentage:
5/12 = x/100
To solve for x (the percentage), cross-multiply:
12x = 500
x = 500/12 ≈ 41.67
This method provides the same result as the primary method, demonstrating the versatility of proportions in solving percentage problems.
Understanding the Significance of Rounding
The result of 41.67% is a rounded figure. The actual decimal representation of 5/12 is a repeating decimal (0.416666...). The level of precision required depends on the context of the problem. For most everyday applications, rounding to two decimal places (41.67%) is sufficient. However, in scientific or engineering contexts, a higher level of precision might be necessary.
Practical Applications of Fraction-to-Percentage Conversion
The ability to convert fractions to percentages has numerous practical applications:
- Calculating Discounts: A store offering a 5/12 discount on an item means a discount of approximately 41.67%.
- Determining Progress: If 5 out of 12 tasks are completed, the progress is approximately 41.67% complete.
- Analyzing Data: In statistical analysis, converting fractions to percentages facilitates easier interpretation and comparison of data.
- Financial Calculations: Understanding percentage changes in investments or budgets requires the ability to convert fractions to percentages.
- Everyday Life: Calculating tips, determining sale prices, or understanding survey results all involve working with fractions and percentages.
Mastering Fraction-to-Percentage Conversions: Tips and Practice
Mastering the conversion of fractions to percentages requires consistent practice. Here are some tips:
- Practice Regularly: Solve a variety of fraction-to-percentage conversion problems to build your skills and confidence.
- Understand the Concepts: Ensure you thoroughly understand the underlying concepts of fractions, decimals, and percentages.
- Use Different Methods: Experiment with different conversion methods to find the approach that best suits your learning style.
- Check Your Work: Always verify your results using alternative methods or calculators to ensure accuracy.
- Seek Help When Needed: Don't hesitate to seek help from teachers, tutors, or online resources if you encounter difficulties.
Beyond 5/12: Expanding Your Understanding
The process of converting 5/12 to a percentage is a specific example of a broader skill: converting any fraction to a percentage. The fundamental steps remain the same: divide the numerator by the denominator and multiply the result by 100. Practice with various fractions to solidify your understanding and build your mathematical fluency. Consider working through examples with different numerators and denominators, including those resulting in repeating decimals, to fully grasp the concept and the importance of appropriate rounding. Understanding these core mathematical concepts provides a strong foundation for tackling more complex mathematical problems in various fields.
Conclusion
Converting the fraction 5/12 to a percentage, approximately 41.67%, is a straightforward process achievable through several methods. This conversion, while seemingly simple, represents a crucial mathematical skill with numerous practical applications across various domains. By understanding the underlying principles and practicing regularly, you can master this essential skill and enhance your mathematical abilities for both academic and real-world situations. Remember that consistent practice is key to mastering this valuable skill. The more you work with fractions and percentages, the more intuitive the conversion process will become.
Latest Posts
Latest Posts
-
5 Converted To A Decimal Is
Apr 02, 2025
-
How Long Does It Take To Get To The Venus
Apr 02, 2025
-
03 Miles Is How Many Feet
Apr 02, 2025
-
Ions How Are Ions Made From Neutral Atoms
Apr 02, 2025
-
How Many Ounces Is 280 Grams
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percent Of 5/12 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
