Calculate The Atomic Mass Of Silicon
listenit
Mar 13, 2025 · 5 min read
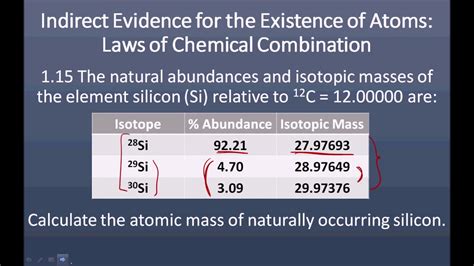
Table of Contents
Calculating the Atomic Mass of Silicon: A Deep Dive
Silicon, a crucial element in the semiconductor industry and Earth's crust, presents a fascinating case study in understanding atomic mass calculations. This comprehensive guide will delve into the intricacies of determining silicon's atomic mass, exploring the underlying principles, the significance of isotopes, and the practical applications of this calculation. We'll unravel the seemingly simple concept of atomic mass, revealing the complex interplay of isotopes and their relative abundances.
Understanding Atomic Mass
The atomic mass (or atomic weight) of an element is the average mass of all the isotopes of that element, weighted by their relative abundances in nature. It's not the mass of a single atom, but rather a weighted average reflecting the isotopic composition found typically in the Earth's crust or in specific samples. This is crucial because most elements exist as a mixture of isotopes.
Isotopes: The Building Blocks of Atomic Mass
Isotopes are atoms of the same element that have the same number of protons but differ in the number of neutrons. This difference in neutron number leads to variations in atomic mass. While all isotopes of an element exhibit the same chemical properties, their physical properties, including mass, can differ significantly.
Silicon, for instance, has three naturally occurring stable isotopes:
- Silicon-28 (²⁸Si): Contains 14 protons and 14 neutrons.
- Silicon-29 (²⁹Si): Contains 14 protons and 15 neutrons.
- Silicon-30 (³⁰Si): Contains 14 protons and 16 neutrons.
The abundance of each isotope varies slightly depending on the source of the silicon sample. However, these variations are typically small and within a well-defined range.
Calculating the Weighted Average Atomic Mass
Calculating the atomic mass involves a weighted average calculation, considering both the mass and relative abundance of each isotope. The formula is straightforward:
Atomic Mass = (Mass of Isotope 1 × Abundance of Isotope 1) + (Mass of Isotope 2 × Abundance of Isotope 2) + ... + (Mass of Isotope n × Abundance of Isotope n)
The abundances are usually expressed as percentages or decimal fractions (percentages divided by 100).
Step-by-Step Calculation for Silicon
Let's perform the calculation for silicon, using commonly accepted isotopic abundances:
1. Identify the Isotopes and their Masses:
- ²⁸Si: Mass ≈ 27.9769 amu (atomic mass units)
- ²⁹Si: Mass ≈ 28.9765 amu
- ³⁰Si: Mass ≈ 29.9738 amu
2. Determine the Isotopic Abundances:
These values can vary slightly based on the source, but generally accepted values are:
- ²⁸Si: Abundance ≈ 92.23% or 0.9223
- ²⁹Si: Abundance ≈ 4.67% or 0.0467
- ³⁰Si: Abundance ≈ 3.10% or 0.0310
3. Perform the Weighted Average Calculation:
Atomic Mass of Silicon = (27.9769 amu × 0.9223) + (28.9765 amu × 0.0467) + (29.9738 amu × 0.0310)
Atomic Mass of Silicon ≈ 25.801 amu + 1.353 amu + 0.929 amu
Atomic Mass of Silicon ≈ 28.083 amu
This calculated value is very close to the standard atomic mass of silicon listed on the periodic table, which typically rounds to 28.0855 amu. Slight variations in the final result may occur due to the use of slightly different isotopic abundances.
Significance of Accurate Atomic Mass Determination
Accurate determination of atomic mass is crucial for various scientific and industrial applications:
- Chemical Stoichiometry: Accurate atomic masses are essential for performing precise stoichiometric calculations, vital in chemistry, chemical engineering, and materials science. Understanding the precise mass of reactants and products allows for accurate predictions of reaction yields and compositions.
- Nuclear Physics and Isotope Analysis: The study of isotopes and their abundances provides insights into nuclear processes, geological history, and even forensic science. Isotope ratio mass spectrometry (IRMS) is a powerful technique used for precise isotopic measurements, often used in environmental studies and archaeology.
- Semiconductor Industry: Silicon's precise atomic mass is critical for the semiconductor industry. The controlled doping of silicon with other elements to achieve specific electrical properties relies on accurate calculations involving atomic masses. Variations in isotopic composition can influence the performance of semiconductor devices.
- Material Science and Engineering: The properties of materials are profoundly influenced by their atomic composition. Accurate atomic mass data helps predict and control the properties of alloys, ceramics, and other materials.
- Analytical Chemistry and Mass Spectrometry: Mass spectrometry techniques are used extensively in analytical chemistry to determine the isotopic composition of samples. This information is then used to calculate the weighted average atomic mass, providing valuable insights into the sample's origin, purity, and composition.
Factors Influencing Isotopic Abundance
The isotopic abundance of an element can vary slightly based on several factors:
- Geological Location: The isotopic composition of elements in geological samples can vary depending on the location and geological history of the sample. This is due to factors such as radioactive decay and geological processes.
- Sample Preparation: The methods used to prepare a sample for analysis can influence the measured isotopic abundances. Contamination or fractionation during sample preparation can lead to inaccurate results.
- Instrumentation: The precision and accuracy of the measurement instruments also play a role. Mass spectrometry techniques, while highly accurate, still have limitations.
Beyond Silicon: Extending the Principles
The principles used to calculate the atomic mass of silicon are applicable to all elements. However, the complexity increases with the number of isotopes and their varying abundances. For elements with many isotopes or radioactive isotopes with short half-lives, the calculation becomes more intricate. The standard atomic weights listed on the periodic table represent the best estimate based on the available data and are periodically revised as new data emerges.
Conclusion: The Importance of Precision
Calculating the atomic mass of silicon, while seemingly simple, reveals a rich interplay of nuclear physics, chemistry, and analytical techniques. This precise calculation is not merely an academic exercise; it forms the foundation for countless scientific and industrial applications. The accuracy of these calculations is paramount, influencing the precision and reliability of results in diverse fields, highlighting the critical role of accurate atomic mass determination in various scientific and technological endeavors. Understanding the underlying principles and the impact of isotopic variations is essential for appreciating the full significance of this seemingly fundamental calculation.
Latest Posts
Latest Posts
-
What Is Mercurys State Of Matter At Room Temperature
May 09, 2025
-
What Does The Graduated Cylinder Measure
May 09, 2025
-
Which Level Of Classification Is The Most Specific
May 09, 2025
-
Log X 4 Solve For X
May 09, 2025
-
How To Make Casio Calculator Show Scientific Notation
May 09, 2025
Related Post
Thank you for visiting our website which covers about Calculate The Atomic Mass Of Silicon . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.