50 Of 30 Is 15 Of What Number
listenit
May 25, 2025 · 4 min read
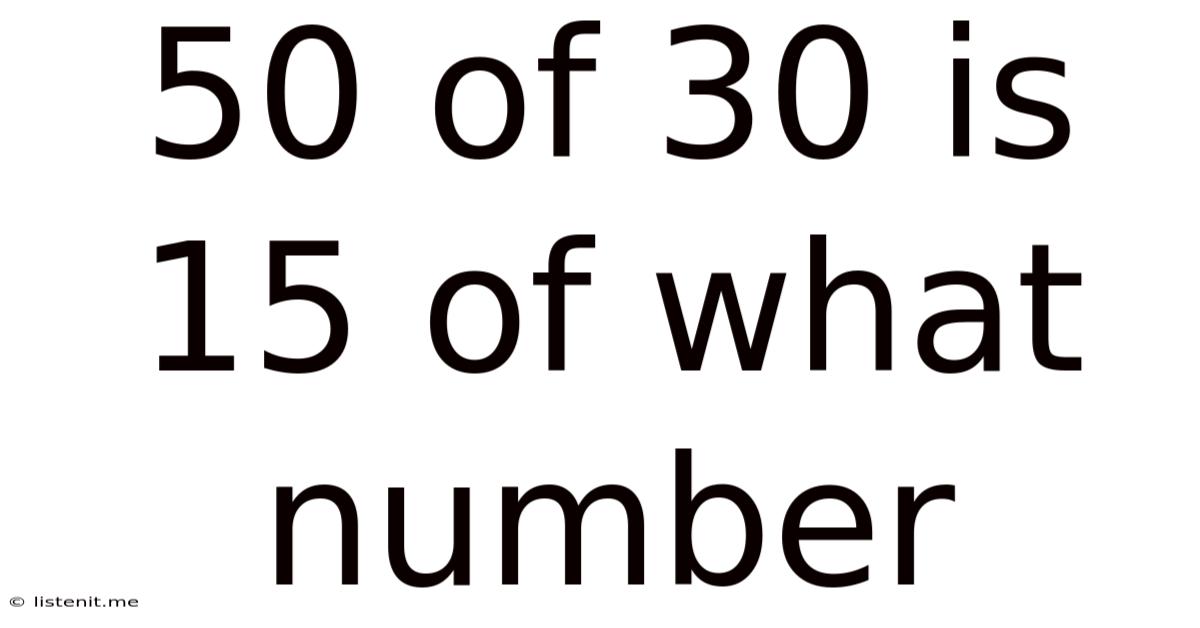
Table of Contents
50 is 15% of What Number? Unraveling Percentage Problems
Many find percentage problems daunting, but with a structured approach, they become manageable. This article delves into the question, "50 is 15% of what number?", providing a comprehensive explanation of the solution, exploring different approaches, and highlighting practical applications. We'll break down the process step-by-step, ensuring you not only understand this specific problem but gain a firm grasp of solving percentage problems in general.
Understanding the Problem: Deconstructing the Question
The core of the problem lies in understanding the relationship between percentages, parts, and wholes. We are given that 50 represents 15% of an unknown number. Our goal is to find this unknown number. This is a classic example of a percentage problem where we know the percentage and the part, but we need to find the whole.
Method 1: Using the Percentage Formula
The most straightforward method involves using the basic percentage formula:
Part / Whole = Percentage / 100
In our problem:
- Part: 50
- Percentage: 15
- Whole: This is what we need to find, let's represent it with 'x'.
Substituting these values into the formula, we get:
50 / x = 15 / 100
Now, we solve for x:
- Cross-multiply: 50 * 100 = 15 * x
- Simplify: 5000 = 15x
- Isolate x: x = 5000 / 15
- Calculate: x ≈ 333.33
Therefore, 50 is approximately 15% of 333.33.
Method 2: Using Decimal Equivalents
Percentages can be easily converted to decimals by dividing by 100. 15% is equivalent to 0.15. We can rewrite the problem as:
0.15 * x = 50
Solving for x:
- Divide both sides by 0.15: x = 50 / 0.15
- Calculate: x ≈ 333.33
This method offers a slightly more concise approach, especially for those comfortable working with decimals.
Method 3: Proportion Method
This method uses ratios to solve the problem. We can set up a proportion:
15/100 = 50/x
This reads as "15 is to 100 as 50 is to x". Cross-multiplying and solving for x gives the same result as the previous methods: x ≈ 333.33
Understanding the Significance of Approximations
Notice that in all methods, we arrived at an approximate answer (333.33). This is because the initial problem involves a percentage that doesn't divide evenly into 100. If the percentage was a whole number divisor of 100 (e.g., 50%, 25%, etc.), the result would be a whole number.
Practical Applications: Real-World Scenarios
Percentage problems are ubiquitous in daily life. Here are some practical applications of understanding this type of calculation:
-
Finance: Calculating interest earned, discounts on purchases, tax amounts, profit margins, and loan repayments all involve percentage calculations. For example, if you receive a 15% discount on a $50 item, you saved $7.50 (50 * 0.15), meaning you paid $42.50. Alternatively, if you want to know the original price of an item marked down 15% to $50, this problem is solved in the same way as the original problem in this article.
-
Sales and Marketing: Analyzing sales figures, conversion rates, market share, and customer acquisition costs frequently uses percentages. Determining the success of a marketing campaign may require comparing the number of sales generated to the number of marketing contacts made - then expressing this as a percentage.
-
Science and Research: Expressing experimental data, statistical analysis, and error margins often rely on percentages. For instance, reporting the percentage of participants in a study who responded positively to a particular treatment.
-
Everyday Life: Calculating tips in restaurants, understanding nutritional labels (e.g., percentage of daily value for nutrients), or determining the sale price of an item after a discount all require understanding percentages.
Expanding Your Percentage Skills: Tips and Tricks
Mastering percentage problems requires practice and a solid understanding of the underlying concepts. Here are some helpful strategies:
- Practice regularly: The more you practice, the more comfortable you’ll become with different problem types.
- Master the formula: Understanding the formula (Part / Whole = Percentage / 100) is crucial.
- Visualize the problem: Drawing diagrams or charts can help visualize the relationships between the parts and the whole.
- Use different methods: Try solving problems using multiple methods to reinforce your understanding.
- Check your work: Always double-check your answers to ensure accuracy.
- Use online resources: Many online calculators and tutorials can help you practice and refine your skills.
Beyond the Basics: More Complex Percentage Problems
While this article focused on a fundamental percentage problem, there are more complex scenarios to explore:
- Calculating percentage increase or decrease: This involves finding the percentage change between two values.
- Compound interest: This accounts for interest earned on both the principal and accumulated interest.
- Percentage points versus percentages: This distinction is crucial in some financial and statistical contexts.
By mastering the basics, you build a strong foundation for tackling more advanced percentage problems.
Conclusion: Unlocking the Power of Percentages
Understanding percentages is a valuable skill applicable across various fields. By grasping the fundamentals and practicing regularly, you can confidently solve percentage problems like "50 is 15% of what number?" and apply this knowledge to numerous real-world scenarios. Remember, the key is to break down the problem into manageable steps and utilize the appropriate method for solving. This empowers you to interpret data effectively, make informed decisions, and confidently navigate the numerical aspects of everyday life. The more you practice, the easier and more intuitive this process will become, ultimately enhancing your problem-solving abilities.
Latest Posts
Latest Posts
-
Calculating Ac Unit Size For House
May 26, 2025
-
How Many 1 5 Are In 3
May 26, 2025
-
What Is The Greatest Common Factor Of 21 And 49
May 26, 2025
-
55 Out Of 100 As A Percentage
May 26, 2025
-
What Is 10 Percent Of 25
May 26, 2025
Related Post
Thank you for visiting our website which covers about 50 Of 30 Is 15 Of What Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.