55 Out Of 100 As A Percentage
listenit
May 26, 2025 · 4 min read
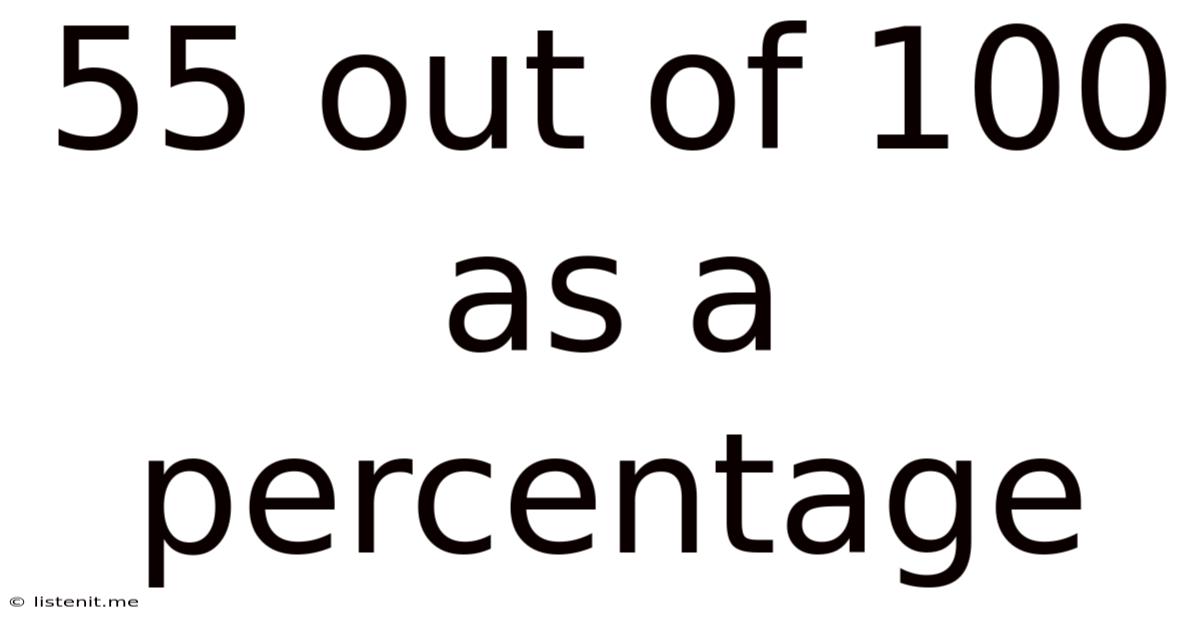
Table of Contents
55 Out of 100 as a Percentage: A Comprehensive Guide
Converting fractions to percentages is a fundamental skill in mathematics with wide-ranging applications in various fields. Understanding how to do this accurately is crucial for everything from calculating grades and discounts to analyzing data and interpreting statistics. This comprehensive guide will delve into the process of determining what 55 out of 100 represents as a percentage, exploring different methods and highlighting the importance of percentage calculations in everyday life and professional settings.
Understanding Percentages
Before we dive into the specifics of 55 out of 100, let's establish a firm grasp of what percentages actually represent. A percentage is simply a fraction expressed as a portion of 100. The word "percent" itself comes from the Latin "per centum," meaning "out of a hundred." Therefore, any percentage can be written as a fraction with a denominator of 100. For example, 25% is equivalent to 25/100, which simplifies to 1/4.
This fundamental understanding is key to converting fractions to percentages and vice-versa. It allows us to easily visualize and compare different proportions.
Calculating 55 Out of 100 as a Percentage: The Basic Method
The simplest method for calculating 55 out of 100 as a percentage involves directly applying the definition of a percentage. We can express 55 out of 100 as a fraction: 55/100.
Since a percentage is a fraction out of 100, this fraction is already in the ideal format. To convert this fraction to a percentage, we simply multiply the fraction by 100%:
(55/100) * 100% = 55%
Therefore, 55 out of 100 is 55%.
Alternative Methods for Percentage Calculation
While the direct method is the most straightforward, several other methods can be used to calculate percentages, particularly useful when dealing with more complex fractions.
Method 1: Using Decimal Conversion
First, convert the fraction 55/100 into a decimal by dividing the numerator (55) by the denominator (100):
55 ÷ 100 = 0.55
Then, multiply the decimal by 100% to express it as a percentage:
0.55 * 100% = 55%
This method is particularly helpful when working with fractions that don't have a denominator of 100.
Method 2: Proportion Method
This method is beneficial for understanding the underlying relationship between fractions and percentages. We set up a proportion:
x/100 = 55/100
To solve for x (the percentage), we cross-multiply:
100x = 55 * 100
100x = 5500
x = 5500 / 100
x = 55
Therefore, x = 55%, confirming our previous calculations.
Real-World Applications of Percentage Calculations: Examples
The ability to calculate percentages is incredibly valuable in a multitude of real-world scenarios. Here are a few examples demonstrating the practical application of these calculations:
1. Academic Performance:
Imagine a student scores 55 out of 100 on a test. Understanding that this equates to 55% allows for easy assessment of their performance and comparison with other students or benchmarks. This provides valuable insights for both students and educators.
2. Sales and Discounts:
Retail stores frequently use percentages to advertise discounts. A "55% off" sale means that the price of an item is reduced by 55% of its original value. Calculating the final price requires applying percentage calculations.
3. Financial Calculations:
Interest rates on loans and investments are expressed as percentages. Calculating the total interest payable or the future value of an investment necessitates a strong understanding of percentage calculations. For example, a 55% return on investment is a significant gain.
4. Data Analysis:
In many professions, data analysis involves working with percentages. For example, market researchers may analyze survey results, expressing responses as percentages to understand trends and consumer preferences. A 55% market share represents a substantial dominance in a particular industry.
5. Statistics and Probability:
Percentages play a crucial role in statistical analysis and probability. For example, expressing the likelihood of an event occurring as a percentage helps in decision-making. A 55% chance of rain suggests a reasonably high probability of precipitation.
Beyond 55%: Mastering Percentage Calculations for Any Fraction
While we've focused on 55 out of 100, the principles discussed are applicable to calculating any fraction as a percentage. The general formula is:
(Numerator / Denominator) * 100% = Percentage
For example, to find what 72 out of 150 represents as a percentage, you would calculate:
(72 / 150) * 100% ≈ 48%
Common Mistakes to Avoid When Calculating Percentages
Even with seemingly simple calculations, common mistakes can occur. Here are some pitfalls to watch out for:
- Incorrect Order of Operations: Remember the order of operations (PEMDAS/BODMAS). Division should be performed before multiplication.
- Decimal Point Errors: When working with decimals, ensure you handle decimal points correctly during multiplication and division.
- Misunderstanding the Question: Always carefully read the question and identify what is being asked. Sometimes, the context requires an understanding of the percentage change rather than simply expressing a fraction as a percentage.
Conclusion: The Importance of Percentage Skills
Understanding how to calculate percentages, even something as seemingly simple as 55 out of 100, is a valuable skill for various aspects of life. From academic success to financial literacy and professional proficiency, the ability to work confidently with percentages opens up a wide range of opportunities. Mastering this fundamental concept ensures greater accuracy, efficiency, and informed decision-making in numerous situations. So, next time you encounter a percentage calculation, remember the simple yet powerful methods outlined in this guide.
Latest Posts
Latest Posts
-
What Is The Gcf Of 96 And 72
May 26, 2025
-
2 5 8 As An Improper Fraction
May 26, 2025
-
How Much Will 401k Pay Per Month
May 26, 2025
-
Calculate The Area Of The Following Figure
May 26, 2025
-
An Hour And 20 Minutes From Now
May 26, 2025
Related Post
Thank you for visiting our website which covers about 55 Out Of 100 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.