18 Out Of 60 As A Percentage
listenit
Mar 29, 2025 · 5 min read
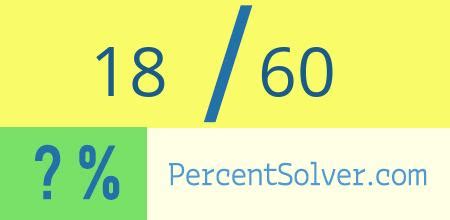
Table of Contents
- 18 Out Of 60 As A Percentage
- Table of Contents
- 18 out of 60 as a Percentage: A Comprehensive Guide
- Understanding Percentages
- Method 1: Using the Fraction Method
- Method 2: Using Proportions
- Method 3: Using a Calculator
- Real-World Applications of Percentage Calculations
- Beyond the Basics: Working with Percentages
- Practical Examples
- Conclusion: Mastering Percentages for Success
- Latest Posts
- Latest Posts
- Related Post
18 out of 60 as a Percentage: A Comprehensive Guide
Calculating percentages is a fundamental skill in various aspects of life, from everyday budgeting to complex financial analysis. Understanding how to convert fractions into percentages is crucial for making informed decisions and interpreting data effectively. This article will thoroughly explain how to calculate "18 out of 60 as a percentage," exploring different methods and providing practical examples to solidify your understanding. We'll also delve into the broader context of percentage calculations and their applications.
Understanding Percentages
A percentage is a way of expressing a number as a fraction of 100. The term "percent" is derived from the Latin "per centum," meaning "out of a hundred." Essentially, a percentage represents a portion or proportion of a whole. For instance, 50% means 50 out of 100, or one-half.
Method 1: Using the Fraction Method
The most straightforward approach to calculating "18 out of 60 as a percentage" is using the fraction method:
-
Express the values as a fraction: The phrase "18 out of 60" can be directly translated into the fraction 18/60.
-
Convert the fraction to a decimal: Divide the numerator (18) by the denominator (60): 18 ÷ 60 = 0.3
-
Convert the decimal to a percentage: Multiply the decimal by 100: 0.3 x 100 = 30%
Therefore, 18 out of 60 is 30%.
Method 2: Using Proportions
This method utilizes the concept of proportions to solve for the percentage. We can set up a proportion:
- 18/60 = x/100
Where:
- 18 represents the part
- 60 represents the whole
- x represents the unknown percentage
- 100 represents the total percentage
To solve for x, cross-multiply:
- 18 * 100 = 60 * x
- 1800 = 60x
Now, divide both sides by 60:
- x = 1800 ÷ 60
- x = 30
Therefore, x = 30%, confirming our result from the previous method.
Method 3: Using a Calculator
Most calculators have a percentage function that simplifies the calculation significantly. Simply enter 18 ÷ 60 and then multiply the result by 100. The calculator will directly display the answer as 30%.
Real-World Applications of Percentage Calculations
Understanding percentage calculations is vital in numerous everyday situations:
-
Financial Literacy: Calculating interest rates, discounts, tax rates, profit margins, and investment returns all rely heavily on percentage calculations. For example, calculating the interest earned on a savings account or understanding the discount offered during a sale requires precise percentage computations.
-
Academic Performance: Grading systems frequently use percentages to represent student performance. Calculating your overall grade point average (GPA) requires understanding and applying percentage calculations to various coursework scores.
-
Data Analysis: Percentages are indispensable for analyzing data and presenting findings in a clear and concise manner. Researchers, statisticians, and business analysts rely on percentage calculations to interpret trends, make comparisons, and draw conclusions from large datasets. For instance, market share analysis uses percentages to illustrate the relative size of competing companies in a particular market.
-
Health and Fitness: Understanding percentages is critical when monitoring health and fitness goals. Tracking progress towards fitness goals like weight loss or increased muscle mass often involves monitoring percentage changes over time. Nutritional information on food labels also uses percentages to show the proportion of daily recommended values of various nutrients.
-
Cooking and Baking: Recipes often express ingredient quantities as percentages of the total weight or volume. Understanding percentages is crucial for scaling recipes up or down while maintaining the desired proportions.
-
Sales and Marketing: Companies use percentages to represent sales growth, conversion rates, customer acquisition costs, and market penetration. These metrics help businesses to measure their performance and make strategic decisions. For instance, tracking the percentage of website visitors who make a purchase is essential for optimizing online sales funnels.
Beyond the Basics: Working with Percentages
While calculating "18 out of 60 as a percentage" is a relatively simple problem, mastering percentage calculations requires understanding how to perform various operations involving percentages. Here are some key concepts:
-
Finding a Percentage of a Number: To find, say, 25% of 80, multiply 80 by 0.25 (which is the decimal equivalent of 25%).
-
Finding the Percentage Increase or Decrease: To calculate a percentage increase or decrease, determine the difference between the two values, divide the difference by the original value, and then multiply by 100. For example, if a price increases from $50 to $60, the percentage increase is ((60-50)/50) * 100 = 20%.
-
Calculating Percentage Change: This involves finding the difference between the new value and the original value, then dividing by the original value and multiplying by 100. A positive percentage change indicates an increase, while a negative percentage change indicates a decrease.
-
Working with Multiple Percentages: When dealing with multiple percentages, remember to account for the compounding effect. For instance, a 10% discount followed by a 20% discount is not equivalent to a 30% discount.
Practical Examples
Let's explore some more practical examples using percentage calculations:
Example 1: Sales Discount
A store offers a 20% discount on a $100 item. What is the final price?
- Discount amount: 20% of $100 = 0.20 * $100 = $20
- Final price: $100 - $20 = $80
Example 2: Tax Calculation
A 5% sales tax is added to a $50 purchase. What is the total cost?
- Tax amount: 5% of $50 = 0.05 * $50 = $2.50
- Total cost: $50 + $2.50 = $52.50
Example 3: Grade Calculation
A student scores 45 out of 50 on a test. What is their percentage score?
- Percentage score: (45/50) * 100 = 90%
Example 4: Profit Margin
A business sells a product for $20 and its cost of production is $10. What is the profit margin?
- Profit: $20 - $10 = $10
- Profit margin: ($10/$20) * 100 = 50%
These examples highlight the versatility and practicality of percentage calculations in various real-life scenarios.
Conclusion: Mastering Percentages for Success
The ability to confidently calculate and interpret percentages is a highly valuable skill with broad applications across many fields. Whether you're managing personal finances, analyzing data, or making informed decisions in any area of life, a solid understanding of percentage calculations is essential. This article has provided a thorough explanation of how to calculate "18 out of 60 as a percentage," along with several practical examples and a broader exploration of percentage calculations and their numerous applications. By mastering these concepts, you'll be well-equipped to navigate various situations that require this fundamental mathematical skill. Remember to practice regularly and apply these concepts to your everyday life to truly solidify your understanding and improve your numerical fluency.
Latest Posts
Latest Posts
-
Least Common Multiple Of 4 And 30
Apr 01, 2025
-
The Principle Of Probability Can Be Used To
Apr 01, 2025
-
Y Varies Jointly As X And Z
Apr 01, 2025
-
What Is The Si Base Unit For Time
Apr 01, 2025
-
Energy Measured In Units Is Called
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about 18 Out Of 60 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
