Y Square Root Of X 3
listenit
Apr 03, 2025 · 6 min read
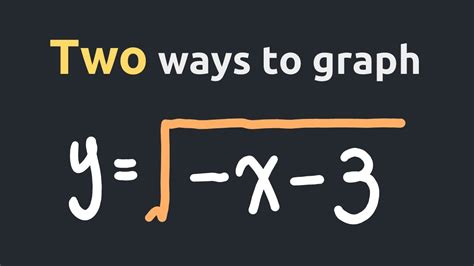
Table of Contents
Y = √(x³): A Deep Dive into the Cube Root Function and its Applications
The equation y = √(x³), also expressible as y = x^(3/2), represents a fascinating mathematical relationship with diverse applications across various fields. This function, a combination of a power function and a square root, exhibits unique properties and behaviors that warrant a thorough examination. This article delves into the intricacies of y = √(x³), covering its graph, domain and range, derivatives, integrals, and real-world applications.
Understanding the Function: y = √(x³)
At its core, y = √(x³) involves two fundamental mathematical operations: cubing and square rooting. Cubing a number (raising it to the power of 3) involves multiplying the number by itself three times. The square root operation finds a number that, when multiplied by itself, yields the original number. Combining these, y = √(x³) signifies taking the cube of x and then finding its principal square root. Importantly, since we are dealing with a square root, we are primarily focused on the positive square root, excluding negative solutions.
Domain and Range
The domain of a function refers to the set of all possible input values (x-values) for which the function is defined. In the case of y = √(x³), the expression under the square root must be non-negative. Since x³ is non-negative only when x ≥ 0, the domain of the function is x ≥ 0.
The range of a function comprises all possible output values (y-values). As x increases from 0, x³ increases, and consequently, √(x³) also increases. Since the square root of any non-negative number is non-negative, the range of y = √(x³) is y ≥ 0.
Graphing the Function: Visualizing y = √(x³)
The graph of y = √(x³) provides a visual representation of the function's behavior. It starts at the origin (0, 0) and increases steadily as x increases. Unlike a simple parabola, the curve is steeper at higher x values due to the nature of the cube root function.
Key Characteristics of the Graph
- Origin as a Point: The graph passes through the origin (0,0).
- Non-negative Values: The graph exists only in the first quadrant (where both x and y are non-negative).
- Increasing Function: The function is monotonically increasing; as x increases, y also increases.
- Concavity: The graph is concave up, meaning its rate of increase accelerates as x increases.
Using graphing tools or software will give you a precise visual representation of this curve, further solidifying your understanding of its behavior.
Calculus and y = √(x³): Derivatives and Integrals
The calculus of the function y = √(x³) provides insights into its rate of change and accumulation.
Derivatives: Rate of Change
The derivative of a function describes its instantaneous rate of change. Finding the derivative of y = √(x³) involves applying the power rule of differentiation:
d/dx (x^(3/2)) = (3/2)x^(3/2 - 1) = (3/2)x^(1/2) = (3/2)√x
This derivative, (3/2)√x, represents the slope of the tangent line to the curve of y = √(x³) at any given point. Observe that the derivative is also non-negative for x ≥ 0, consistent with the fact that the original function is monotonically increasing.
Integrals: Accumulation
The integral of a function represents the accumulation of its values over a given interval. To find the definite integral of y = √(x³) from a to b, we utilize the power rule of integration:
∫(x^(3/2)) dx = (2/5)x^(5/2) + C
Where 'C' is the constant of integration. Evaluating this expression at the limits 'a' and 'b' gives the area under the curve between these two points.
Applications of y = √(x³): Real-World Relevance
The function y = √(x³) isn't just a theoretical concept; it has practical applications in various fields:
Physics: Modeling Physical Phenomena
- Fluid Mechanics: In certain fluid dynamics problems, relationships involving cube roots and square roots appear when dealing with the flow of fluids through pipes or channels. The function can approximate relationships between flow rate, pressure, and pipe diameter.
- Mechanics: In certain mechanical systems, the relationship between power, force, and velocity can sometimes be modeled using similar fractional power functions.
Engineering: Designing and Optimizing Systems
- Civil Engineering: The function can potentially be used in certain structural calculations where stress and strain are non-linearly related.
- Electrical Engineering: Similar fractional power functions may occur when modeling the relationship between current, voltage, and resistance in non-linear electrical components.
Economics and Finance: Analyzing Trends and Data
- Growth Models: While not a direct application, the function's characteristics are analogous to certain growth models where the rate of growth accelerates over time (similar to the concavity of the graph). Modified versions of this function could be used to approximate certain economic growth models.
- Statistical Analysis: The function’s properties can be used as an element in certain statistical calculations, though this might involve transformations or combinations with other functions.
Biology and Medicine: Modeling Biological Processes
- Population Dynamics: In some biological models, relationships between population growth and resource availability could exhibit similar non-linear relationships approximated by functions like this.
It's crucial to understand that while the direct application of y = √(x³) may not be explicitly stated in many real-world problems, its underlying mathematical principles and properties often contribute to more complex models used across different fields.
Expanding the Understanding: Related Concepts
Several related concepts further enhance our understanding of the function y = √(x³):
- Fractional Exponents: The function is expressed using a fractional exponent (3/2), demonstrating how fractional exponents extend the power rule of differentiation and integration.
- Power Functions: The function belongs to a broader class of power functions, where the exponent can be any real number. Studying power functions provides a wider context for understanding y = √(x³).
- Composite Functions: The function is a composite function, combining a cubic function (x³) and a square root function (√x). Understanding composite functions is crucial to grasping its behavior.
- Inverse Functions: Finding the inverse of the function y = √(x³) involves solving for x in terms of y, leading to x = y^(2/3). Understanding inverse functions helps in understanding the relationship between the input and output values.
Conclusion: The Significance of y = √(x³)
The function y = √(x³) may appear simple at first glance, but it exhibits rich mathematical properties and surprisingly diverse applications. Understanding its domain, range, graph, derivatives, and integrals provides a solid foundation for appreciating its role in modeling various real-world phenomena. Through this in-depth analysis, we've highlighted its significance and connected it to broader mathematical concepts. By exploring the function's nuances, we gain a deeper understanding of the power and elegance of mathematical modeling. Further research into specific applications within your chosen field will reveal its unique relevance and potential for problem-solving and innovation.
Latest Posts
Latest Posts
-
12 Out Of 40 Is What Percent
Apr 04, 2025
-
66 2 3 Percent As A Fraction
Apr 04, 2025
-
How Many Ounces Is 68 G
Apr 04, 2025
-
2 Log 4 Log 2 Log 2
Apr 04, 2025
-
What Is The Charge On Cobalt
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about Y Square Root Of X 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
