2 Log 4 Log 2 Log 2
listenit
Apr 04, 2025 · 5 min read
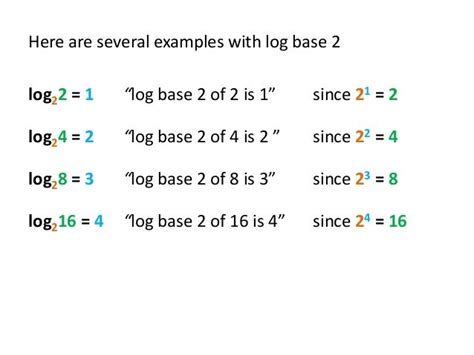
Table of Contents
Decoding the Mathematical Enigma: 2 log 4 log 2 log 2
The seemingly simple expression "2 log 4 log 2 log 2" presents a fascinating challenge in mathematical interpretation. Its ambiguity stems from the lack of explicit parentheses or operators to clarify the order of operations. This article will delve into the various possible interpretations of this expression, exploring the underlying logarithmic properties, and ultimately providing a comprehensive analysis of its potential solutions. We'll also touch upon the importance of clear notation in mathematics and how ambiguous expressions can lead to confusion.
Understanding the Fundamentals: Logarithms and their Properties
Before tackling the core problem, let's refresh our understanding of logarithms. A logarithm is essentially the inverse operation of exponentiation. The expression log<sub>b</sub>(x) = y means that b<sup>y</sup> = x, where 'b' is the base, 'x' is the argument, and 'y' is the logarithm (or exponent). Common bases include 10 (common logarithm) and e (natural logarithm).
Several key properties govern logarithmic operations:
- Product Rule: log<sub>b</sub>(xy) = log<sub>b</sub>(x) + log<sub>b</sub>(y)
- Quotient Rule: log<sub>b</sub>(x/y) = log<sub>b</sub>(x) - log<sub>b</sub>(y)
- Power Rule: log<sub>b</sub>(x<sup>y</sup>) = y log<sub>b</sub>(x)
- Change of Base: log<sub>b</sub>(x) = log<sub>a</sub>(x) / log<sub>a</sub>(b)
These properties are crucial for simplifying and manipulating logarithmic expressions. The absence of explicitly stated bases in "2 log 4 log 2 log 2" necessitates careful consideration of these properties and the potential impact of different base assumptions.
Potential Interpretations and Solutions
The ambiguity of "2 log 4 log 2 log 2" arises from the multiple ways it can be interpreted due to the lack of parentheses or explicit operators. Let's explore several possibilities:
Interpretation 1: Sequential Application
One possible interpretation is to sequentially apply the logarithm operations. Assuming a base of 10 for all logarithms (unless otherwise specified), we could interpret the expression as:
2 * (log 4) * (log 2) * (log 2)
Using a calculator, we can approximate this as:
2 * (0.602) * (0.301) * (0.301) ≈ 0.109
Interpretation 2: Nested Logarithms
Another interpretation involves nested logarithms, possibly implying:
2 * log(4 * log(2 * log 2))
This scenario requires us to carefully determine the order of operations. First we have to find the value of log 2. The common logarithm (base 10) of 2 is approximately 0.301. Then, we multiply that with the next 2; 2 * 0.301 = 0.602. Next, we take the logarithm of 0.602, which is approximately -0.219. Then, we multiply this by 4; 4 * (-0.219) = -0.876. Finally, we take the logarithm of -0.876, which results in a complex number (since the logarithm of a negative number is not a real number). This indicates a limitation of this interpretation.
Interpretation 3: Logarithm of a Product
Another possible interpretation is:
log<sub>2</sub>(4) * log<sub>2</sub>(2) * log<sub>2</sub>(2)
In this scenario, we use base 2 explicitly. Since log<sub>2</sub>(4) = 2, log<sub>2</sub>(2) = 1, this expression simplifies to:
2 * 1 * 1 = 2
This interpretation yields a clear, concise, and real-numbered result.
Interpretation 4: Base Consideration
The base of the logarithm significantly influences the outcome. Had the expression been written with explicit bases, the solution would be unambiguous. For example:
2 log₁₀(4) log₁₀(2) log₁₀(2) would yield a different result from 2 log₂(4) log₂(2) log₂(2). The crucial point here is that without specifying the base, multiple interpretations and solutions become plausible.
The Importance of Clear Mathematical Notation
The analysis of "2 log 4 log 2 log 2" highlights the critical importance of precise mathematical notation. Ambiguity in notation leads to multiple interpretations and potentially incorrect results. Parentheses, brackets, and explicit base specification are essential tools for avoiding confusion and ensuring clarity.
In professional contexts such as scientific papers, engineering projects, and software development, unambiguous notation is paramount to avoid errors and misinterpretations that could have significant consequences. The use of mathematical software and tools that help in verifying the expression and calculating the result are also crucial for avoiding human error.
Expanding on Logarithmic Applications
Logarithms are not merely abstract mathematical concepts; they have wide-ranging applications across various fields:
- Physics: Logarithmic scales (decibels, Richter scale) are used to measure quantities spanning vast ranges.
- Chemistry: pH calculations utilize logarithms to quantify acidity and alkalinity.
- Computer Science: Logarithmic algorithms are crucial in optimizing search and sorting operations.
- Finance: Compound interest calculations often involve logarithms.
- Biology: Modeling population growth often uses logarithmic functions.
Understanding logarithms and their properties is therefore essential for tackling numerous real-world problems. The apparent simplicity of "2 log 4 log 2 log 2" belies the importance of clear notation and the potential for varied interpretations based on different assumptions.
Conclusion: The Value of Precision
The exploration of "2 log 4 log 2 log 2" serves as a powerful lesson in the necessity of precise mathematical notation. While we have explored several potential interpretations and solutions, the lack of explicit parentheses and base specifications makes definitive conclusions difficult. The ambiguity highlights the potential for errors stemming from unclear notation. The importance of meticulousness and precision in mathematical expression cannot be overstated, particularly in fields where accuracy is critical. Always ensure your mathematical expressions are unambiguous and clearly defined to avoid misinterpretations and ensure the correctness of your calculations. Understanding the fundamental properties of logarithms and mastering their applications will equip you with valuable tools for solving a wide array of complex problems across various disciplines. Remember that clear communication, not just correct calculation, is key to effective mathematical work.
Latest Posts
Latest Posts
-
What The Square Root Of 30
Apr 04, 2025
-
Monomer For Nucleic Acids Is Called
Apr 04, 2025
-
16 And 2 3 As A Fraction
Apr 04, 2025
-
Iron Rusting Is A Chemical Change
Apr 04, 2025
-
How Many Unpaired Electrons Does Phosphorus Have
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about 2 Log 4 Log 2 Log 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
