Write A Linear Function F With The Given Values
listenit
Mar 29, 2025 · 5 min read
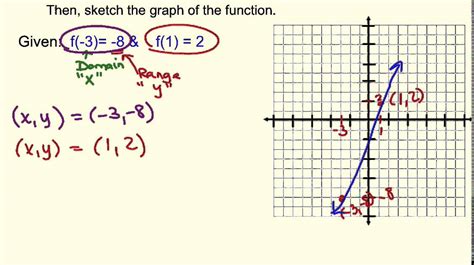
Table of Contents
Writing a Linear Function f with Given Values: A Comprehensive Guide
Understanding how to write a linear function given specific values is a fundamental concept in algebra. Linear functions are characterized by their constant rate of change, represented by the slope, and their ability to be expressed in the form f(x) = mx + b, where 'm' is the slope and 'b' is the y-intercept. This guide will walk you through various methods of determining the linear function, covering different scenarios and providing practical examples to solidify your understanding.
Understanding the Basics: Slope and y-intercept
Before delving into the methods, let's revisit the core components of a linear function:
1. The Slope (m):
The slope represents the rate of change of the function. It indicates how much the y-value changes for every one-unit change in the x-value. A positive slope signifies an increasing function, while a negative slope indicates a decreasing function. The slope is calculated using any two points (x₁, y₁) and (x₂, y₂) on the line using the formula:
m = (y₂ - y₁) / (x₂ - x₁)
Example: If we have points (2, 4) and (4, 8), the slope is (8 - 4) / (4 - 2) = 2. This means for every one-unit increase in x, y increases by 2.
2. The y-intercept (b):
The y-intercept is the point where the line intersects the y-axis. This occurs when x = 0. The y-intercept represents the initial value or the value of the function when the independent variable (x) is zero.
Methods for Determining the Linear Function
We'll explore three primary methods for determining the linear function 'f(x) = mx + b' given specific values:
Method 1: Using Two Points
This is the most common method when given two points on the line.
Steps:
-
Calculate the slope (m): Use the formula m = (y₂ - y₁) / (x₂ - x₁) with your two points (x₁, y₁) and (x₂, y₂).
-
Find the y-intercept (b): Substitute the slope (m) and one of the points (x, y) into the equation
y = mx + b. Solve for 'b'. -
Write the linear function: Substitute the calculated values of 'm' and 'b' into the equation
f(x) = mx + b.
Example: Find the linear function that passes through the points (1, 3) and (4, 9).
-
Calculate the slope: m = (9 - 3) / (4 - 1) = 2
-
Find the y-intercept: Using the point (1, 3) and m = 2, we have: 3 = 2(1) + b => b = 1
-
Write the linear function: f(x) = 2x + 1
Method 2: Using the Slope and a Point
If you're given the slope and one point on the line, the process is slightly simplified.
Steps:
-
Use the point-slope form: The point-slope form of a linear equation is
y - y₁ = m(x - x₁), where 'm' is the slope and (x₁, y₁) is the given point. -
Solve for y: Simplify the equation to isolate 'y' and express it in the form
y = mx + b. -
Write the linear function: Replace 'y' with 'f(x)' to obtain the function notation.
Example: Find the linear function with a slope of 3 and passing through the point (2, 5).
-
Use the point-slope form: y - 5 = 3(x - 2)
-
Solve for y: y - 5 = 3x - 6 => y = 3x - 1
-
Write the linear function: f(x) = 3x - 1
Method 3: Using the y-intercept and a Point
If you know the y-intercept and another point, you can also easily determine the linear function.
Steps:
-
Determine the slope: Use the y-intercept (0, b) and the given point (x, y) to calculate the slope using the slope formula: m = (y - b) / x
-
Write the linear function: Substitute the calculated slope (m) and the y-intercept (b) into the equation
f(x) = mx + b.
Example: Find the linear function with a y-intercept of 4 and passing through the point (3, 10).
-
Determine the slope: m = (10 - 4) / 3 = 2
-
Write the linear function: f(x) = 2x + 4
Handling Special Cases: Vertical and Horizontal Lines
Linear functions also include special cases of vertical and horizontal lines. These do not follow the standard f(x) = mx + b format.
Vertical Lines:
Vertical lines have an undefined slope. Their equation is of the form x = c, where 'c' is a constant. They cannot be represented as a function because for a single x-value, there are multiple y-values.
Example: A vertical line passing through x = 2 is represented as x = 2.
Horizontal Lines:
Horizontal lines have a slope of 0. Their equation is of the form y = c, where 'c' is a constant. These can be represented as a function f(x) = c.
Example: A horizontal line passing through y = 5 is represented as y = 5, or f(x) = 5.
Advanced Applications and Real-World Examples
The ability to construct linear functions finds wide application in various fields:
-
Physics: Modeling motion with constant velocity (distance as a function of time).
-
Economics: Representing supply and demand relationships.
-
Finance: Calculating simple interest growth.
-
Engineering: Designing linear systems and analyzing their behavior.
-
Data Analysis: Creating trend lines from data sets to predict future outcomes.
Consider a scenario where a taxi charges a flat fee of $3 plus $2 per mile. We can model this as a linear function:
- Let x be the number of miles driven.
- Let f(x) be the total cost.
The linear function would be: f(x) = 2x + 3
Error Handling and Troubleshooting
When determining a linear function, it's crucial to be mindful of potential errors:
-
Incorrect slope calculation: Double-check your calculations to ensure you haven't made any arithmetic mistakes.
-
Using incorrect points: Verify that the points used are actually on the line.
-
Misinterpreting the y-intercept: Ensure you correctly identify the point where the line crosses the y-axis.
Conclusion
Mastering the skill of writing linear functions from given values is crucial for success in algebra and various applied fields. By understanding the underlying principles of slope and y-intercept and employing the methods outlined above, you can confidently tackle a range of problems, from simple exercises to complex real-world applications. Remember to always double-check your work and consider potential sources of error to ensure accuracy in your calculations. Through consistent practice and problem-solving, you'll become adept at constructing linear functions and utilizing them to model and understand linear relationships.
Latest Posts
Latest Posts
-
Which Is Denser Oceanic Or Continental Crust
Mar 31, 2025
-
Root 3 Divided By Root 3
Mar 31, 2025
-
The Shoulder Is Blank To The Elbow
Mar 31, 2025
-
Write 2 5 As A Decimal Number
Mar 31, 2025
-
What Is A Characteristic Of A Base
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about Write A Linear Function F With The Given Values . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
