Which Value Is Equal To 1/4 Of 1 360
listenit
May 24, 2025 · 4 min read
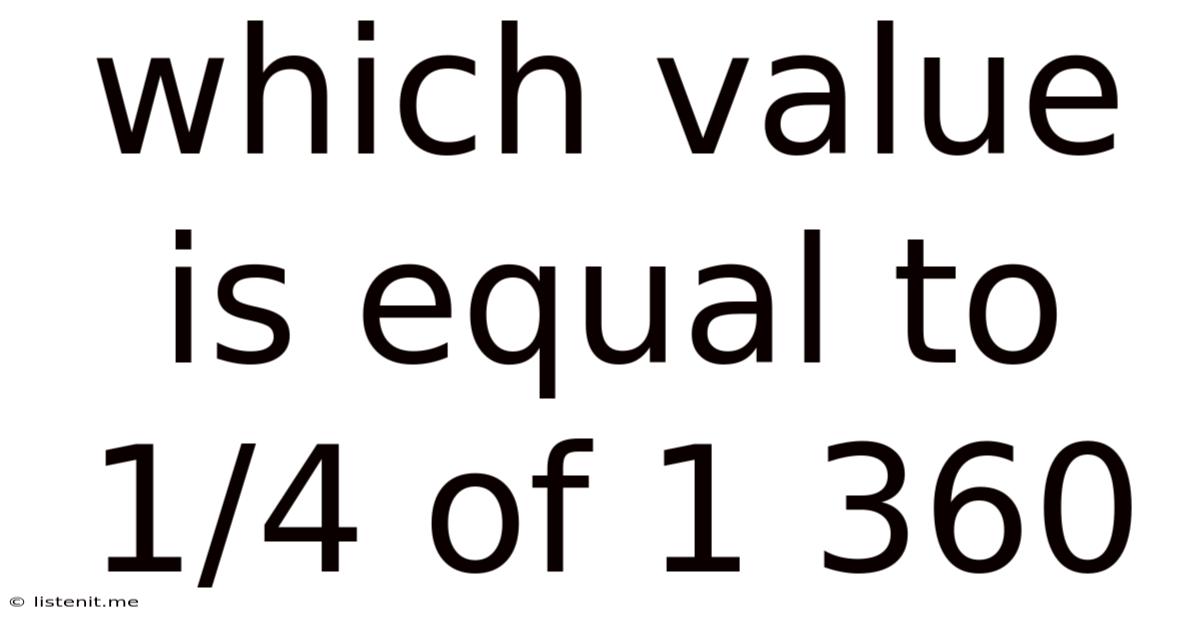
Table of Contents
Which Value is Equal to 1/4 of 1,360? A Deep Dive into Fractions and Percentages
Finding a fraction of a number is a fundamental mathematical concept that appears in countless real-world scenarios. Whether you're splitting a bill, calculating discounts, or tackling more complex problems, understanding fractions is crucial. This article explores the question: "Which value is equal to 1/4 of 1,360?" and delves into the broader context of fractions, percentages, and their applications.
Understanding Fractions
A fraction represents a part of a whole. It consists of two parts: the numerator (the top number) and the denominator (the bottom number). The numerator indicates how many parts you have, while the denominator indicates how many parts the whole is divided into. For example, in the fraction 1/4, the numerator is 1 and the denominator is 4. This means we have 1 out of 4 equal parts.
Calculating 1/4 of 1,360
To find 1/4 of 1,360, we perform the following calculation:
(1/4) * 1360
This can be solved in two primary ways:
Method 1: Division
Since finding a fraction of a number involves division, we can divide 1360 by the denominator (4):
1360 / 4 = 340
Therefore, 1/4 of 1360 is 340.
Method 2: Multiplication
Alternatively, we can multiply 1360 by the numerator (1) and then divide by the denominator (4):
(1360 * 1) / 4 = 340
This method yields the same result: 340.
Practical Applications: Where This Calculation Matters
The ability to calculate fractions of numbers is incredibly useful in various contexts. Here are a few examples:
1. Financial Calculations: Discounts and Interest
Imagine a store offering a 25% discount on an item priced at $1,360. A 25% discount is equivalent to 1/4. Using our calculation, we know the discount amount is $340, meaning the final price after the discount would be $1,020 ($1,360 - $340).
2. Portioning and Sharing: Dividing Resources Equally
If you have 1,360 candies and want to divide them equally among four friends, each friend receives 1/4 of the total, which is 340 candies.
3. Measurement and Conversions: Working with Units
Many conversion problems involve fractions. For example, converting kilometers to meters involves understanding that 1 kilometer is equal to 1000 meters. If you need to find the equivalent of 1/4 of a kilometer in meters, you would perform (1/4) * 1000 = 250 meters.
4. Recipe Scaling: Adjusting Ingredients
If a recipe calls for 1,360 grams of flour and you want to make only 1/4 of the recipe, you'd need 340 grams of flour.
5. Data Analysis: Understanding Proportions
In data analysis, calculating fractions is essential for understanding proportions and trends within datasets. For example, if 1/4 of survey respondents chose a particular option, this represents a significant portion of the overall responses.
Expanding the Concept: Percentages and Their Relationship to Fractions
Percentages are closely related to fractions. A percentage is a fraction expressed as a part of 100. For example, 25% is equivalent to 25/100, which simplifies to 1/4. Therefore, calculating 25% of 1,360 is the same as calculating 1/4 of 1,360.
Converting Fractions to Percentages
To convert a fraction to a percentage, you divide the numerator by the denominator and then multiply by 100. For example:
(1/4) * 100 = 25%
Converting Percentages to Fractions
To convert a percentage to a fraction, write the percentage as a fraction with a denominator of 100 and simplify if possible. For example:
25% = 25/100 = 1/4
Beyond 1/4: Working with Other Fractions
The same principles apply when working with other fractions. For example, to find 2/5 of 1,360, we would perform the calculation:
(2/5) * 1360 = (2 * 1360) / 5 = 2720 / 5 = 544
This demonstrates the versatility of these mathematical concepts. The ability to work fluently with fractions is a crucial skill that extends far beyond simple arithmetic.
Problem-Solving Strategies: Approaching Fraction Problems Effectively
When encountering problems involving fractions, consider these strategies:
- Simplify the fraction: Reduce the fraction to its simplest form before performing calculations to make the arithmetic easier.
- Convert to decimals: If preferred, convert fractions to decimals before calculating. For example, 1/4 is equivalent to 0.25.
- Use visual aids: Draw diagrams or use manipulatives (like blocks or circles) to visualize the problem, particularly when working with younger learners.
- Break down complex problems: Divide complex problems into smaller, more manageable steps.
- Check your work: After completing the calculation, always check your answer to ensure it makes sense within the context of the problem.
Conclusion: The Importance of Mastering Fractions
Mastering the ability to work with fractions is essential for success in mathematics and across various real-world applications. Understanding how to calculate fractions of numbers, convert between fractions and percentages, and apply these concepts to problem-solving strengthens your numerical literacy and enhances your ability to tackle more complex mathematical challenges. The seemingly simple question of finding 1/4 of 1,360 opens a window into a broader world of mathematical understanding, highlighting the importance of this fundamental skill. Remember, practice is key! The more you work with fractions, the more comfortable and confident you'll become.
Latest Posts
Latest Posts
-
6 Weeks And 6 Days Ago From Today
May 24, 2025
-
What Is 4 75 As A Fraction
May 24, 2025
-
2 5 8 As An Improper Fraction
May 24, 2025
-
What Is 5 6 Of 20
May 24, 2025
-
What Percent Is Equal To 0 35
May 24, 2025
Related Post
Thank you for visiting our website which covers about Which Value Is Equal To 1/4 Of 1 360 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.