What Percent Is 1 Out Of 3
listenit
May 09, 2025 · 5 min read
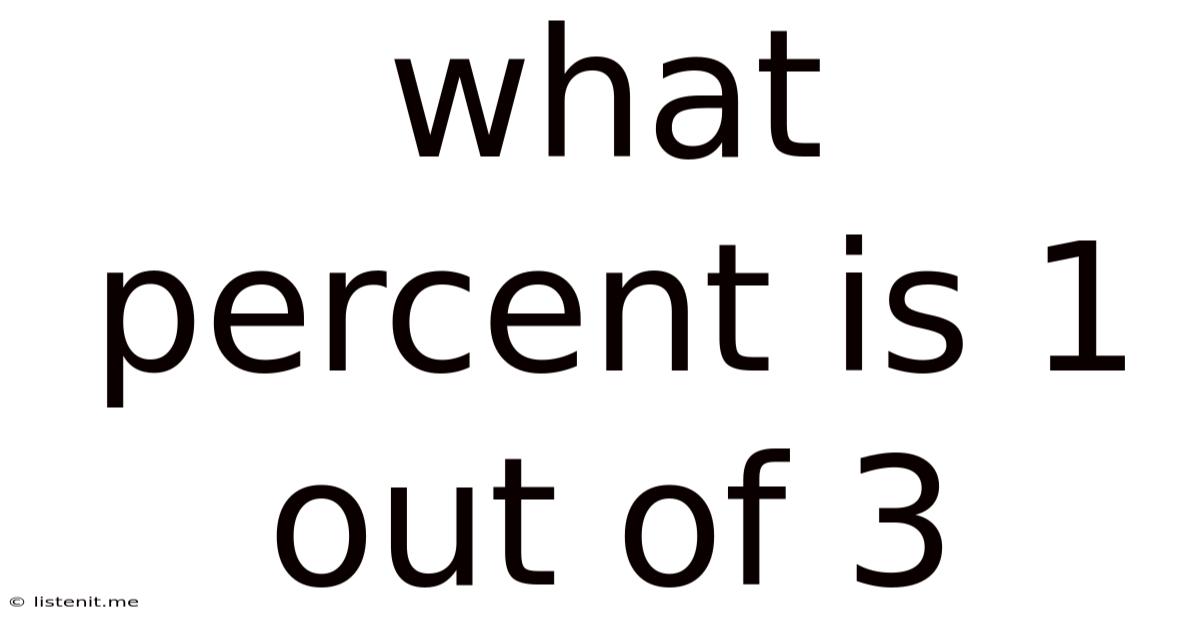
Table of Contents
What Percent is 1 out of 3? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous areas of life, from calculating discounts and taxes to analyzing data and understanding statistics. One common question that arises is: what percent is 1 out of 3? This seemingly simple question opens the door to a broader understanding of percentage calculations and their practical applications. This comprehensive guide will not only answer this specific question but also equip you with the knowledge to tackle similar problems with confidence.
Calculating the Percentage: A Step-by-Step Approach
The core concept behind calculating percentages lies in understanding the relationship between a part and a whole. In this case, 1 is the part, and 3 is the whole. To find the percentage, we follow these simple steps:
-
Divide the part by the whole: We start by dividing the number representing the part (1) by the number representing the whole (3). This gives us: 1 ÷ 3 = 0.333...
-
Multiply the result by 100: To convert the decimal value into a percentage, we multiply it by 100. This is because a percentage represents a fraction of 100. So, 0.333... × 100 = 33.333...
-
Round to the desired precision: The result, 33.333..., is a recurring decimal. For practical purposes, we usually round the percentage to a specific number of decimal places. Rounding to two decimal places, we get 33.33%.
Therefore, 1 out of 3 is 33.33%.
Understanding the Concept: Fractions, Decimals, and Percentages
To truly grasp percentage calculations, it's crucial to understand the interconnectedness of fractions, decimals, and percentages. They are simply different ways of representing the same numerical value.
-
Fraction: A fraction expresses a part of a whole as a ratio of two numbers, the numerator (top number) and the denominator (bottom number). In our example, 1/3 is the fraction representing 1 out of 3.
-
Decimal: A decimal expresses a part of a whole as a number with a decimal point, representing tenths, hundredths, thousandths, and so on. The decimal equivalent of 1/3 is 0.333...
-
Percentage: A percentage expresses a part of a whole as a fraction of 100. It is obtained by multiplying the decimal equivalent by 100 and adding the % symbol.
The ability to convert between these three forms is invaluable in solving various mathematical problems.
Practical Applications of Percentage Calculations
The ability to calculate percentages has wide-ranging applications in various aspects of life:
1. Finance and Budgeting:
-
Discounts: Determining the final price of an item after a percentage discount. For example, a 20% discount on a $100 item means a reduction of $20, resulting in a final price of $80.
-
Interest Rates: Calculating the interest earned on savings accounts or the interest payable on loans. Understanding interest rates is crucial for making informed financial decisions.
-
Tax Calculations: Determining the amount of tax payable on income, goods, or services. Tax calculations are essential for compliance with financial regulations.
-
Investment Returns: Assessing the performance of investments by calculating the percentage return on investment. This helps in evaluating the effectiveness of different investment strategies.
2. Data Analysis and Statistics:
-
Data Representation: Percentages are often used to represent data in graphs and charts, making complex information easier to understand and interpret.
-
Probability and Statistics: Percentages are used to express probabilities and statistical measures, such as the percentage of people who prefer a particular product or the percentage change in a particular variable over time.
-
Survey Results: Analyzing results from surveys and polls. For instance, understanding the percentage of respondents who agree with a particular statement.
3. Everyday Life:
-
Tip Calculations: Calculating the appropriate tip amount in restaurants or for services rendered.
-
Recipe Scaling: Adjusting ingredient quantities in recipes based on the number of servings.
-
Sales and Promotions: Understanding discounts and offers in stores and online.
Advanced Percentage Calculations: Beyond the Basics
While calculating "what percent is 1 out of 3" provides a foundational understanding, let's explore more complex scenarios:
1. Finding the Percentage Increase or Decrease:
Calculating percentage change involves determining the difference between two values and expressing it as a percentage of the original value. The formula is:
[(New Value - Original Value) / Original Value] x 100
For example, if a value increases from 100 to 120, the percentage increase is:
[(120 - 100) / 100] x 100 = 20%
2. Finding the Original Value After a Percentage Change:
If you know the final value and the percentage change, you can work backward to find the original value. This often requires using algebraic techniques.
For example, if a value increased by 15% to reach 115, the original value can be calculated as:
Let x be the original value. Then: x + 0.15x = 115 => 1.15x = 115 => x = 100
3. Dealing with More Complex Fractions:
Calculating percentages with more complex fractions follows the same principles. For example, to find the percentage represented by 5 out of 8, we perform the calculation:
(5 ÷ 8) x 100 = 62.5%
Mastering Percentages: Tips and Tricks
-
Practice Regularly: The more you practice percentage calculations, the more comfortable and efficient you'll become.
-
Use Online Calculators: Many online calculators are available to help with percentage calculations, especially for more complex scenarios.
-
Understand the Underlying Concepts: A thorough grasp of the relationship between fractions, decimals, and percentages will significantly enhance your ability to solve percentage problems.
-
Check Your Work: Always double-check your calculations to ensure accuracy. Even a small error can lead to significant discrepancies in the final result.
-
Break Down Complex Problems: If faced with a complex percentage problem, break it down into smaller, more manageable steps.
Conclusion: The Power of Percentages
Understanding percentages is a valuable skill with far-reaching applications. Whether you're managing your finances, analyzing data, or simply navigating everyday life, the ability to calculate and interpret percentages will empower you to make informed decisions and understand the world around you more effectively. Starting with the basic understanding of how to calculate what percent is 1 out of 3, as shown in this guide, provides a strong foundation for tackling more complex percentage calculations and leveraging this essential mathematical tool to its full potential. Remember to practice regularly and apply your knowledge in various contexts to master this crucial skill.
Latest Posts
Latest Posts
-
What Does Slope Of Velocity Time Graph Indicate
May 10, 2025
-
How Many Moles Are In 25 0 Grams Of Water
May 10, 2025
-
How Are Biomes Related To Ecosystems
May 10, 2025
-
Which Statement Does The Kinetic Theory Explain
May 10, 2025
-
Line Parallel To The X Axis
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Percent Is 1 Out Of 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.