What Is X To The 1 2
listenit
Apr 01, 2025 · 5 min read
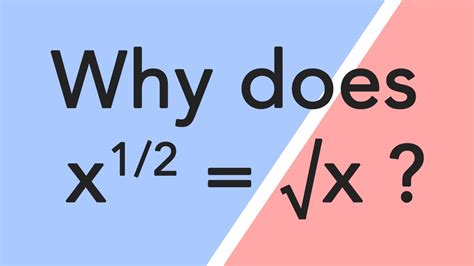
Table of Contents
What is x to the 1/2? Understanding Square Roots and Fractional Exponents
The expression "x to the 1/2" represents a fundamental concept in mathematics: the square root of x. Understanding this seemingly simple expression unlocks a deeper comprehension of exponents, radicals, and their applications across various fields, from basic algebra to advanced calculus and beyond. This comprehensive guide will delve into the meaning of x<sup>1/2</sup>, explore its properties, and showcase its practical uses.
Understanding Exponents
Before diving into x<sup>1/2</sup>, let's refresh our understanding of exponents. An exponent (also called a power or index) indicates how many times a base number is multiplied by itself. For instance:
- x<sup>2</sup> means x * x (x squared)
- x<sup>3</sup> means x * x * x (x cubed)
- x<sup>n</sup> means x multiplied by itself 'n' times.
Introducing Fractional Exponents
The beauty of exponents lies in their ability to extend beyond whole numbers. Fractional exponents introduce a new layer of complexity and power. The denominator of a fractional exponent signifies the root, while the numerator signifies the power. Therefore:
- x<sup>m/n</sup> means the nth root of x raised to the power of m. This can also be written as (<sup>n</sup>√x)<sup>m</sup>.
Deciphering x<sup>1/2</sup>
Now, let's apply this knowledge to x<sup>1/2</sup>. Here, the exponent is 1/2. The denominator is 2, indicating a square root, and the numerator is 1, meaning the root is raised to the power of 1 (which doesn't change its value). Therefore:
x<sup>1/2</sup> = √x (the square root of x)
This means finding a number that, when multiplied by itself, equals x. For example:
- 9<sup>1/2</sup> = √9 = 3 because 3 * 3 = 9
- 16<sup>1/2</sup> = √16 = 4 because 4 * 4 = 16
- 25<sup>1/2</sup> = √25 = 5 because 5 * 5 = 25
Beyond Positive Numbers: Considering Negative Numbers and Complex Numbers
While the examples above use positive integers, the concept extends to negative numbers and even complex numbers. However, this introduces some important nuances:
-
Negative Numbers: The square root of a negative number is not a real number. For example, √-9 is not a real number because no real number multiplied by itself equals -9. This necessitates the introduction of imaginary numbers, where the imaginary unit 'i' is defined as √-1. Therefore, √-9 = 3i.
-
Complex Numbers: Complex numbers are numbers that have both a real and an imaginary part, expressed in the form a + bi, where 'a' and 'b' are real numbers and 'i' is the imaginary unit. The square root of a complex number can also be a complex number.
Properties of Square Roots
Understanding the properties of square roots is crucial for manipulating and simplifying expressions involving x<sup>1/2</sup>:
- Product Rule: √(a * b) = √a * √b
- Quotient Rule: √(a / b) = √a / √b
- Simplification: Often, square roots can be simplified by factoring out perfect squares. For example, √12 = √(4 * 3) = √4 * √3 = 2√3
Applications of x<sup>1/2</sup>
The square root, represented by x<sup>1/2</sup>, appears extensively in various mathematical and real-world applications:
-
Geometry: Calculating the length of the hypotenuse in a right-angled triangle using the Pythagorean theorem (a² + b² = c²) inherently involves square roots. Determining the area or volume of geometric shapes often leads to square root calculations.
-
Physics: Many physical phenomena, such as calculating velocities, accelerations, or energies, involve square roots. For example, the speed of a wave is often represented using square roots.
-
Statistics: Standard deviation, a crucial measure of data dispersion, employs square roots in its calculation.
-
Finance: Calculating the present value of future cash flows often requires the use of square roots, particularly in scenarios involving compound interest.
-
Computer Graphics: Square roots are integral to various algorithms used in computer graphics, especially those involving transformations and calculations of distances and angles.
-
Engineering: Many engineering problems, especially those involving structural mechanics, involve equations that necessitate the calculation of square roots to find the relevant parameters.
Solving Equations with x<sup>1/2</sup>
Equations containing x<sup>1/2</sup> can be solved by applying algebraic manipulation techniques. A common approach involves isolating the square root term and then squaring both sides of the equation to eliminate the square root. However, it's crucial to check for extraneous solutions, which are solutions that arise during the process of solving the equation but do not satisfy the original equation.
Example: Solve the equation √(x + 2) = 3
- Square both sides: (√(x + 2))² = 3² => x + 2 = 9
- Solve for x: x = 9 - 2 = 7
- Check the solution: √(7 + 2) = √9 = 3. The solution is valid.
Higher Order Roots and Fractional Exponents
The concept of fractional exponents extends beyond 1/2. For example:
- x<sup>1/3</sup> represents the cube root of x (∛x), meaning finding a number that, when multiplied by itself three times, equals x.
- x<sup>1/4</sup> represents the fourth root of x, and so on.
- x<sup>m/n</sup> as mentioned previously, represents the nth root of x raised to the power of m.
Understanding fractional exponents provides a unified and elegant way to represent and manipulate roots of various orders. It bridges the gap between exponents and radicals, offering a powerful tool for solving a wide range of mathematical problems.
Conclusion
The expression x<sup>1/2</sup>, representing the square root of x, is a fundamental concept in mathematics with far-reaching implications. From its basic definition to its applications in diverse fields, understanding this concept is essential for anyone pursuing a deeper understanding of mathematics and its practical uses. By mastering the manipulation of fractional exponents and understanding the properties of square roots, one can unlock a world of mathematical possibilities and solve complex problems across various disciplines. This comprehensive guide has explored the intricacies of x<sup>1/2</sup>, laying the groundwork for further exploration of advanced mathematical concepts and their real-world applications. Remember to always check your solutions and be mindful of the potential for extraneous solutions when dealing with equations involving square roots and other fractional exponents.
Latest Posts
Latest Posts
-
A Cell Wall Is The
Apr 02, 2025
-
A Parallelogram Is Always A Rectangle
Apr 02, 2025
-
Is Gasoline Evaporated A Chemical Change
Apr 02, 2025
-
Find The Constant A Such That The Function Is Continuous
Apr 02, 2025
-
How Many Ounces Is 500ml Of Water
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about What Is X To The 1 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
