What Is The Symbol For Population Variance
listenit
Apr 03, 2025 · 5 min read
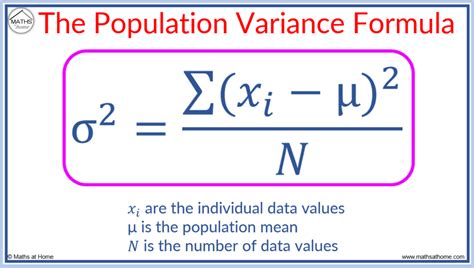
Table of Contents
What is the Symbol for Population Variance? Understanding Variance in Statistics
Understanding statistical concepts like population variance is crucial for anyone working with data analysis, research, or any field that involves interpreting numerical information. This article delves deep into the meaning of population variance, its symbol, its calculation, its importance, and its relationship to other statistical measures. We’ll also explore some common misconceptions and provide clear examples to solidify your understanding.
What is Population Variance?
Population variance is a measure of how spread out a set of data is. More precisely, it quantifies the average squared deviation of each data point from the mean of the population. This means it tells us how much the individual values in a population differ from the overall average. A high variance indicates that the data points are widely scattered around the mean, while a low variance suggests that the data points are clustered closely around the mean.
The Symbol for Population Variance: σ² (Sigma Squared)
The most common symbol used to represent population variance is σ² (sigma squared). This is the lowercase Greek letter sigma, raised to the power of two. The use of sigma stems from its historical use in statistical notation; sigma frequently signifies summation or related operations. Squaring it indicates we're dealing with squared deviations from the mean.
Why Use σ²? The Importance of Notation in Statistics
Consistent notation is vital in statistics to avoid ambiguity and ensure clarity in communication. Using established symbols like σ² helps statisticians and data analysts worldwide understand each other without confusion. The use of σ² immediately signifies that we're discussing the variance of an entire population, differentiating it from the sample variance, which uses a different symbol (s²).
Calculating Population Variance: A Step-by-Step Guide
The formula for calculating population variance (σ²) is:
σ² = Σ(xi - μ)² / N
Let's break down this formula:
- Σ: This is the summation symbol, meaning we'll be summing up a series of values.
- xi: This represents each individual data point in the population.
- μ: This is the population mean (average). Calculated as the sum of all data points divided by the number of data points.
- (xi - μ)²: This is the squared difference between each data point (xi) and the population mean (μ). Squaring the differences ensures that both positive and negative deviations contribute positively to the overall variance. This step is critical because it prevents positive and negative deviations from canceling each other out.
- N: This represents the total number of data points in the population.
Step-by-step example:
Let's say we have a population of five data points: {2, 4, 4, 6, 8}.
-
Calculate the population mean (μ): (2 + 4 + 4 + 6 + 8) / 5 = 4.8
-
Calculate the squared differences (xi - μ)²:
- (2 - 4.8)² = 7.84
- (4 - 4.8)² = 0.64
- (4 - 4.8)² = 0.64
- (6 - 4.8)² = 1.44
- (8 - 4.8)² = 10.24
-
Sum the squared differences: 7.84 + 0.64 + 0.64 + 1.44 + 10.24 = 20.8
-
Divide the sum by N (the number of data points): 20.8 / 5 = 4.16
Therefore, the population variance (σ²) for this dataset is 4.16.
Population Variance vs. Sample Variance
It's crucial to distinguish between population variance (σ²) and sample variance (s²). While both measure the spread of data, they differ in their application and calculation:
-
Population variance (σ²): Calculates the variance of the entire population. It uses the population mean (μ) and the total number of data points in the population (N) in its calculation.
-
Sample variance (s²): Calculates the variance of a sample taken from a larger population. It uses the sample mean (x̄) and the number of data points in the sample (n) in its calculation. The formula for sample variance is slightly different to provide an unbiased estimate of the population variance: s² = Σ(xi - x̄)² / (n-1). Notice the denominator is (n-1) instead of n, which is known as Bessel's correction. This correction helps to adjust for the fact that a sample might not perfectly represent the entire population.
The Importance of Population Variance
Understanding population variance is essential for various reasons:
- Data description: It provides a quantitative measure of data dispersion, helping us understand the spread of values around the mean.
- Statistical inference: It’s a fundamental element in various statistical tests and models, such as hypothesis testing, ANOVA, and regression analysis.
- Risk assessment: In finance and investment, variance is used to measure the risk associated with an investment. Higher variance typically indicates higher risk.
- Quality control: In manufacturing and other industries, variance is used to monitor the consistency of products or processes.
- Predictive modeling: Variance helps in evaluating the accuracy of predictive models by quantifying the spread of predictions around the actual values.
Misconceptions about Population Variance
Several misconceptions frequently surround population variance:
-
Variance and Standard Deviation: While closely related, they are not the same. Variance (σ²) is the average of the squared deviations, while the standard deviation (σ) is the square root of the variance. The standard deviation is often preferred because it's in the same units as the original data, making it easier to interpret.
-
Zero Variance: A population with zero variance indicates that all data points are identical. There is no spread or variation in the data.
-
Negative Variance: Variance can never be negative. This is because the formula involves squaring the deviations, which always results in non-negative values.
Conclusion: Mastering the Symbol and Concept of Population Variance
The symbol σ² (sigma squared) represents population variance – a critical statistical measure for understanding the dispersion of data within an entire population. Understanding its calculation, its relationship to the standard deviation, and its role in various statistical applications is vital for anyone working with data analysis. This article aimed to provide a comprehensive guide, clarifying potential misconceptions and equipping you with the knowledge to confidently utilize population variance in your analytical endeavors. Remember, consistent understanding of statistical concepts like population variance will empower you to extract more meaningful insights from data, whether in research, business, finance, or any other field involving data interpretation.
Latest Posts
Latest Posts
-
What Is 0 05 In A Fraction
Apr 03, 2025
-
2 Pi Over 3 In Degrees
Apr 03, 2025
-
How Much Is 1 3 4
Apr 03, 2025
-
1 Pint Is How Many Lbs
Apr 03, 2025
-
Does Dry Ice Evaporate Or Melt
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about What Is The Symbol For Population Variance . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
