What Is The Square Root Of 841
listenit
Mar 31, 2025 · 5 min read
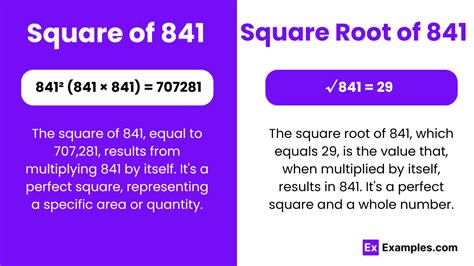
Table of Contents
What is the Square Root of 841? A Deep Dive into Square Roots and Their Applications
The question, "What is the square root of 841?" seems simple enough. A quick calculation reveals the answer: 29. But let's delve deeper than just the immediate answer. This seemingly straightforward mathematical concept opens the door to a fascinating exploration of number theory, its practical applications, and the methods used to solve even more complex problems involving square roots. This article will not only answer the initial question but will also provide a comprehensive understanding of square roots, their properties, and their significance in various fields.
Understanding Square Roots
Before we fully dissect the square root of 841, let's establish a firm foundation in the concept itself. The square root of a number is a value that, when multiplied by itself, equals the original number. In simpler terms, it's the inverse operation of squaring a number.
For example:
- The square root of 9 (√9) is 3, because 3 * 3 = 9.
- The square root of 16 (√16) is 4, because 4 * 4 = 16.
- And, as we've already established, the square root of 841 (√841) is 29, because 29 * 29 = 841.
Methods for Calculating Square Roots
While calculators readily provide the answer, understanding the methods behind calculating square roots is crucial. Several techniques exist, ranging from simple estimation to sophisticated algorithms.
1. Estimation and Trial and Error
For smaller numbers, estimation can be a quick and effective method. Knowing the perfect squares (1, 4, 9, 16, 25, etc.) allows us to narrow down the possibilities. Since 25² = 625 and 30² = 900, we know the square root of 841 lies somewhere between 25 and 30. Trial and error from there would eventually lead to the correct answer of 29.
2. Prime Factorization
Prime factorization is a powerful technique for finding the square root of perfect squares. A perfect square is a number that can be obtained by squaring an integer. To use this method, break down the number into its prime factors. Then, look for pairs of identical prime factors. For each pair, take one factor outside the square root symbol.
Let's try this method on a simpler example first: Finding the square root of 36.
- Prime factorize 36: 2 x 2 x 3 x 3
- Identify pairs: (2 x 2) x (3 x 3)
- Take one factor from each pair: 2 x 3 = 6
- Therefore, √36 = 6
Now, let's attempt this with a larger number (though 841 is better handled using other methods due to the larger prime factors): Finding the square root of 144.
- Prime factorize 144: 2 x 2 x 2 x 2 x 3 x 3
- Identify pairs: (2 x 2) x (2 x 2) x (3 x 3)
- Take one factor from each pair: 2 x 2 x 3 = 12
- Therefore, √144 = 12
This method becomes cumbersome for larger numbers like 841 which has larger prime factors and many more to consider.
3. The Babylonian Method (or Heron's Method)
The Babylonian method is an iterative algorithm that refines an initial guess to get progressively closer to the actual square root. The formula is:
x_(n+1) = 0.5 * (x_n + S/x_n)
Where:
- x_n is the current guess
- x_(n+1) is the next, improved guess
- S is the number whose square root is being sought
Let's apply this to find the square root of 841:
- Initial guess: Let's start with x_0 = 30.
- Iteration 1: x_1 = 0.5 * (30 + 841/30) ≈ 29.01667
- Iteration 2: x_2 = 0.5 * (29.01667 + 841/29.01667) ≈ 29.00000
- Iteration 3: Further iterations will yield even more precise results, rapidly converging towards 29.
This method demonstrates how iterative processes can achieve high accuracy in calculating square roots.
4. Using a Calculator
The most straightforward method is to use a calculator. Most scientific calculators have a dedicated square root function (√). Simply enter 841 and press the square root button to get the answer: 29.
Applications of Square Roots
Square roots are far from a purely theoretical concept; they find wide-ranging applications across various fields:
1. Geometry and Trigonometry
Square roots are fundamental in calculating distances, areas, and volumes. The Pythagorean theorem (a² + b² = c²), used to find the hypotenuse of a right-angled triangle, inherently involves square roots. Calculating the area of a square (side²) or the diagonal of a square requires using square roots. Similarly, many trigonometric calculations depend on square roots.
2. Physics and Engineering
Square roots are integral to solving problems involving velocity, acceleration, and energy. For instance, calculating the speed of an object requires the square root of the sum of the squares of its velocity components (similar to Pythagorean theorem).
3. Statistics and Data Analysis
Standard deviation, a key measure of data dispersion, utilizes square roots to quantify the spread of a dataset around its mean.
4. Computer Graphics and Game Development
Square roots are crucial in rendering 3D graphics and simulating physics in video games. Many calculations involving vectors and transformations require square roots to compute distances, magnitudes, and angles.
5. Finance and Economics
Square roots are used in financial modeling, particularly in portfolio optimization, risk management, and options pricing models.
Beyond the Square Root of 841: Exploring Higher-Order Roots
While we've focused on the square root, it's important to understand that higher-order roots exist as well. These include cube roots (∛), fourth roots (∜), and so on. These roots are defined similarly; for example, the cube root of a number is the value that, when multiplied by itself three times, equals the original number.
Conclusion: The Square Root of 841 and its Broader Significance
The simple question, "What is the square root of 841?" has led us on a journey through various methods of calculating square roots, from estimation and prime factorization to the Babylonian method and using calculators. We have also explored the significance and applications of square roots across diverse fields, demonstrating their importance beyond a simple mathematical concept. Understanding square roots is not just about finding a numerical answer; it's about comprehending the underlying mathematical principles and their role in solving real-world problems. While the answer to the initial question is unequivocally 29, the journey to understanding it has provided a much richer and more comprehensive insight into the world of mathematics.
Latest Posts
Latest Posts
-
How To Find The Mean Of A Probability Distribution
Apr 02, 2025
-
How Do Producers Get Their Energy
Apr 02, 2025
-
The Number Of Protons In An Atom Is That Elements
Apr 02, 2025
-
Is Magnesium Oxide Ionic Or Covalent
Apr 02, 2025
-
What Is The Subunit For Carbohydrates
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about What Is The Square Root Of 841 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
