What Is The Sqaure Root Of 196
listenit
Apr 03, 2025 · 6 min read
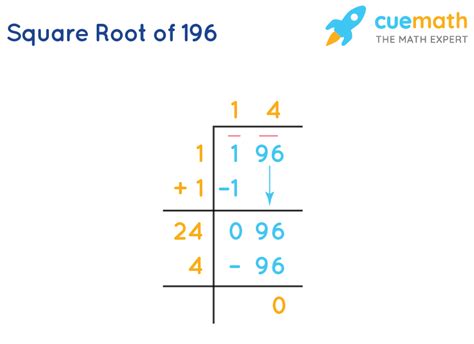
Table of Contents
What is the Square Root of 196? A Deep Dive into Square Roots and Their Applications
The seemingly simple question, "What is the square root of 196?" opens a door to a fascinating world of mathematics, encompassing fundamental concepts, practical applications, and even historical context. While the answer itself is straightforward (14), exploring the broader implications of square roots provides a rich learning experience. This article delves deep into the meaning of square roots, their calculation methods, and their diverse applications across various fields.
Understanding Square Roots: The Fundamentals
The square root of a number is a value that, when multiplied by itself, gives the original number. In simpler terms, it's the inverse operation of squaring a number. For example, the square root of 25 is 5 because 5 multiplied by itself (5 x 5 = 25) equals 25. Therefore, the square root of 196 is the number that, when multiplied by itself, results in 196.
The Symbolism of Square Roots:
The square root is symbolized by the radical symbol (√). Therefore, the square root of 196 is written as √196.
Positive and Negative Square Roots:
It's crucial to understand that every positive number has two square roots: one positive and one negative. While the principal square root (the positive one) is often the focus, it's essential to acknowledge the existence of both. For instance, both 14 and -14, when squared, yield 196 (14 x 14 = 196 and -14 x -14 = 196). However, when asked for the square root of 196, the answer typically refers to the principal square root, which is 14.
Calculating the Square Root of 196: Methods and Approaches
Several methods can be employed to determine the square root of 196. Let's explore some of the most common:
1. Prime Factorization:
This method involves breaking down the number into its prime factors. 196 can be factored as follows:
196 = 2 x 98 = 2 x 2 x 49 = 2 x 2 x 7 x 7 = 2² x 7²
Since the square root involves finding a number that, when multiplied by itself, equals the original number, we can simplify the expression:
√196 = √(2² x 7²) = √2² x √7² = 2 x 7 = 14
This method is particularly useful for larger numbers, offering a systematic way to reduce complexity.
2. Estimation and Trial and Error:
For smaller numbers like 196, estimation can be surprisingly effective. We know that 10² = 100 and 20² = 400. Therefore, the square root of 196 must lie between 10 and 20. Through trial and error (trying 11, 12, 13, and so on), we quickly arrive at 14 as the square root. This method is intuitive but becomes less efficient with larger numbers.
3. Using a Calculator:
The most straightforward approach is using a calculator. Most scientific calculators have a dedicated square root function (√). Simply enter 196 and press the square root button to get the answer: 14.
4. The Babylonian Method (or Heron's Method):
This iterative method provides a highly accurate approximation of square roots. It involves making an initial guess, refining it through successive iterations, and converging towards the correct value. The formula is:
x_(n+1) = 0.5 * (x_n + (N / x_n))
Where:
- x_n is the current approximation
- x_(n+1) is the next approximation
- N is the number for which you're finding the square root (196 in this case)
Let's illustrate with an initial guess of 15:
- x_1 = 15
- x_2 = 0.5 * (15 + (196 / 15)) ≈ 14.033
- x_3 = 0.5 * (14.033 + (196 / 14.033)) ≈ 14.000
As you can see, after just a few iterations, the method converges rapidly to the correct value of 14.
Applications of Square Roots in Various Fields
Square roots are not merely abstract mathematical concepts; they have extensive applications across a multitude of disciplines:
1. Geometry and Physics:
- Calculating Distances: The Pythagorean theorem, a cornerstone of geometry, relies heavily on square roots. This theorem, a² + b² = c², is used to determine the length of the hypotenuse (c) in a right-angled triangle, given the lengths of the other two sides (a and b). The calculation invariably involves taking the square root of the sum of the squares of the other two sides.
- Calculating Area and Volume: Many geometric formulas for calculating areas and volumes involve square roots. For example, the area of a circle (πr²) requires calculating the square root of the area to find the radius.
- Physics: Square roots are crucial in many physics calculations, including those related to velocity, acceleration, and energy. For instance, the formula for calculating the velocity of an object (v) under freefall is v = √(2gh), where 'g' is acceleration due to gravity and 'h' is the height.
2. Engineering and Architecture:
- Structural Design: Engineers use square roots in various calculations related to structural integrity, stress, strain, and stability. This is fundamental to designing safe and reliable structures.
- Construction and Surveying: In construction and surveying, accurate measurements and calculations are paramount, and the use of the Pythagorean theorem (and, thus, square roots) is essential.
3. Finance and Statistics:
- Standard Deviation: In statistics, the standard deviation, a measure of data dispersion, is calculated using square roots. It indicates how spread out the data is from the mean.
- Financial Modeling: Square roots appear in various financial models and calculations, particularly those involving risk assessment and portfolio management.
4. Computer Science:
- Graphics and Game Development: Square roots are fundamental to many algorithms used in computer graphics, 3D rendering, and game development, especially concerning vector calculations and distance computations.
- Search Algorithms: Certain search algorithms utilize square roots for distance calculations and optimization.
5. Everyday Life:
While you might not explicitly calculate square roots daily, their applications are woven into many aspects of modern life. The digital devices you use rely heavily on calculations that involve square roots. Even something as simple as GPS navigation involves complex calculations where square roots are crucial.
The Historical Context of Square Roots
The concept of square roots dates back to ancient civilizations. Evidence suggests that Babylonian mathematicians, as early as 2000 BC, were capable of approximating square roots using iterative methods. The Greeks also made significant contributions, exploring the concept of irrational numbers (numbers that cannot be expressed as a ratio of two integers), which are intrinsically linked to square roots. The modern notation and understanding of square roots evolved over centuries, with contributions from mathematicians across different cultures.
Conclusion: Beyond the Simple Answer
The answer to "What is the square root of 196?" is 14. However, this seemingly simple question unlocks a wealth of knowledge concerning the concept of square roots, their diverse applications, and their rich historical background. Understanding square roots is not just about performing calculations; it's about grasping a fundamental mathematical concept that underpins many aspects of science, engineering, technology, and our daily lives. From the Pythagorean theorem to complex financial models, the seemingly humble square root proves to be an essential tool across numerous fields.
Latest Posts
Latest Posts
-
Question Plane Draw The Skeletal Structures
Apr 04, 2025
-
100 Yards On A Football Field
Apr 04, 2025
-
A Consumer That Eats Only Plants
Apr 04, 2025
-
The Amount Of Energy Required To Raise The Temperature
Apr 04, 2025
-
38 Out Of 40 Is What Percent
Apr 04, 2025
Related Post
Thank you for visiting our website which covers about What Is The Sqaure Root Of 196 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
