What Is The Reciprocal Of 1 3
listenit
Mar 30, 2025 · 5 min read
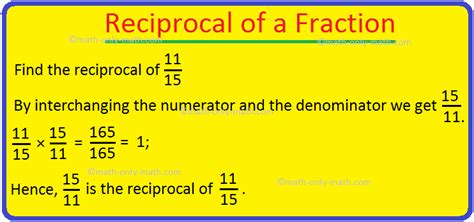
Table of Contents
What is the Reciprocal of 1/3? A Deep Dive into Reciprocals and Their Applications
The seemingly simple question, "What is the reciprocal of 1/3?" opens a door to a fascinating exploration of fundamental mathematical concepts with broad applications across various fields. This article will not only answer this specific question but delve into the broader understanding of reciprocals, their properties, and their practical uses in diverse areas such as algebra, calculus, physics, and even everyday life.
Understanding Reciprocals: The Flip Side of a Number
A reciprocal, also known as a multiplicative inverse, is a number that, when multiplied by the original number, results in the multiplicative identity, which is 1. In simpler terms, it's the number you need to multiply a given number by to get 1.
Finding the Reciprocal: The process of finding the reciprocal is straightforward:
- For a fraction: Simply swap the numerator and the denominator. For example, the reciprocal of 2/5 is 5/2.
- For a whole number: Rewrite the whole number as a fraction with a denominator of 1, then swap the numerator and denominator. The reciprocal of 7 (or 7/1) is 1/7.
- For a decimal: Convert the decimal to a fraction, then find the reciprocal of the fraction. For instance, the reciprocal of 0.25 (or 1/4) is 4/1, or 4.
Calculating the Reciprocal of 1/3
Now, let's address the core question: What is the reciprocal of 1/3?
Following the rule for finding the reciprocal of a fraction, we simply swap the numerator (1) and the denominator (3). Therefore, the reciprocal of 1/3 is 3/1, which simplifies to 3.
This means that 1/3 multiplied by 3 equals 1: (1/3) * 3 = 1. This confirms that 3 is indeed the reciprocal of 1/3.
The Importance of Reciprocals in Mathematics
Reciprocals play a crucial role in various mathematical operations and concepts:
1. Division: The Reciprocal's Role
Division is fundamentally the same as multiplication by the reciprocal. Instead of dividing by a number, you can multiply by its reciprocal. This is particularly useful when working with fractions:
Dividing by 2/5 is the same as multiplying by 5/2.
This equivalence is essential in simplifying complex algebraic expressions and solving equations.
2. Solving Equations: Isolating Variables
Reciprocals are indispensable when solving equations involving fractions or decimals. To isolate a variable multiplied by a fraction or decimal, you multiply both sides of the equation by the reciprocal of that fraction or decimal.
For example, to solve the equation (1/3)x = 5, multiply both sides by 3 (the reciprocal of 1/3):
3 * (1/3)x = 5 * 3
x = 15
3. Simplifying Algebraic Expressions
Reciprocals significantly aid in simplifying complex algebraic expressions. They allow you to rewrite expressions involving division as multiplication, making the simplification process more manageable.
For example, consider the expression: (2/5) / (3/7). This can be rewritten as (2/5) * (7/3), which simplifies to 14/15.
4. Calculus and Derivatives
Reciprocals frequently appear in calculus, especially when working with derivatives and integrals. The derivative of 1/x is -1/x², demonstrating the reciprocal's inherent presence in fundamental calculus concepts.
5. Physics and Engineering
Reciprocals have numerous applications in physics and engineering. For instance, in optics, the reciprocal of focal length is used to calculate magnification. In electronics, the reciprocal of resistance is called conductance, and in mechanics, the reciprocal of spring stiffness is compliance.
Reciprocals of Negative Numbers
The concept of reciprocals extends to negative numbers as well. The reciprocal of a negative number is also negative.
For example:
- The reciprocal of -2 is -1/2.
- The reciprocal of -1/4 is -4.
The same rules for finding reciprocals apply, regardless of whether the number is positive or negative.
Reciprocals of Zero: An Undefined Concept
One important exception is the reciprocal of zero. It's undefined because there is no number that, when multiplied by zero, equals 1. This is a fundamental concept in mathematics and prevents division by zero, which is undefined and leads to inconsistencies in mathematical systems.
Reciprocals in Everyday Life
While reciprocals might seem like an abstract mathematical concept, they are surprisingly prevalent in everyday life, often without us even realizing it. Consider the following examples:
- Cooking: If a recipe calls for 1/3 cup of sugar, and you want to double the recipe, you'll multiply the amount of sugar by 2 (the reciprocal of 1/2).
- Travel: If you know your average speed and the distance you've traveled, you can calculate the time taken by using the reciprocal of your speed (time = distance / speed).
- Finance: Understanding reciprocals can help you calculate interest rates, loan repayments, and investment returns.
Conclusion: The Ubiquity of Reciprocals
The seemingly simple question of finding the reciprocal of 1/3 has led us on a journey exploring a fundamental mathematical concept with far-reaching implications. Reciprocals are not just a tool for solving equations or simplifying expressions; they are integral to our understanding of various mathematical areas and are deeply embedded in numerous applications across science, engineering, and even everyday life. Their importance extends beyond the classroom, showcasing their practical relevance in the real world. Mastering the concept of reciprocals empowers a deeper understanding of mathematics and enhances problem-solving capabilities across diverse fields. By grasping this seemingly simple idea, we gain a more profound appreciation of the interconnectedness and elegance of mathematics. From calculating the reciprocal of 1/3 to understanding its broader implications, the journey underscores the beauty and practicality of mathematical principles.
Latest Posts
Latest Posts
-
How Many Electrons Does O Have
Apr 01, 2025
-
Solve This Inequality J 4 8 4
Apr 01, 2025
-
What Happens When You Cut A Magnet In Half
Apr 01, 2025
-
How Does An Ion Differ From An Atom
Apr 01, 2025
-
A Solution In Which Water Is The Solvent
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about What Is The Reciprocal Of 1 3 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
