What Is The Percentage Of 3 Out Of 10
listenit
May 26, 2025 · 5 min read
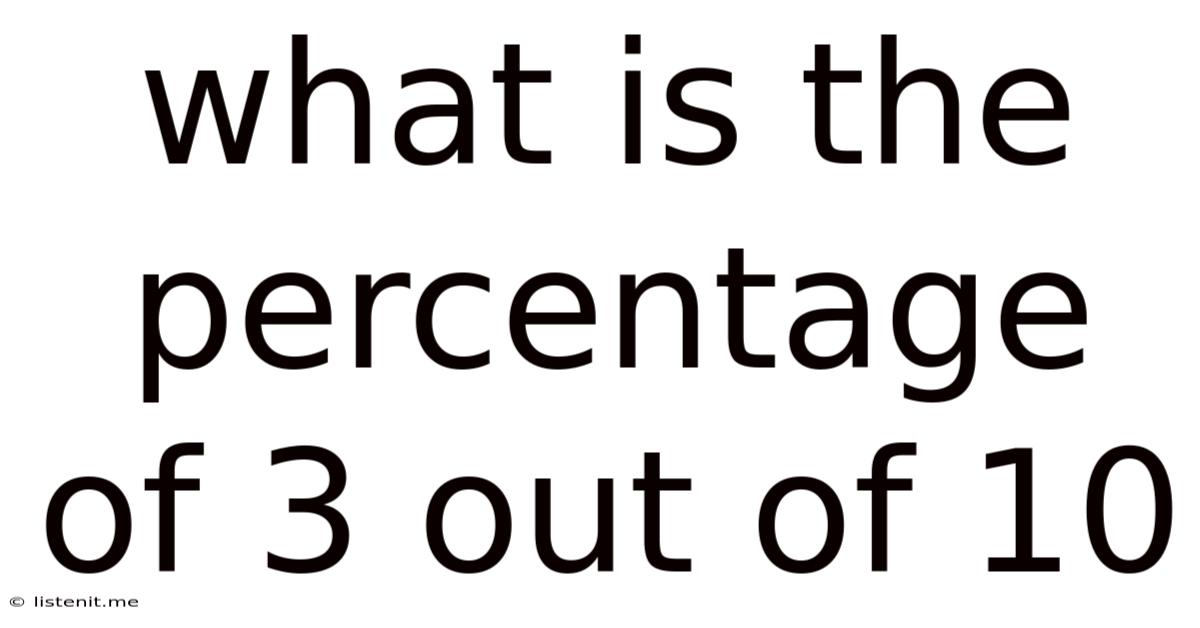
Table of Contents
What is the Percentage of 3 out of 10? A Comprehensive Guide to Percentages
Understanding percentages is a fundamental skill in mathematics with broad applications in daily life, from calculating discounts and sales tax to comprehending statistics and financial reports. This article delves into the seemingly simple question, "What is the percentage of 3 out of 10?", providing a comprehensive explanation not just of the answer but of the underlying concepts and methods involved. We'll explore various approaches to solving this type of problem and expand on how these calculations can be applied in diverse scenarios.
Understanding Percentages: The Basics
A percentage is a way of expressing a number as a fraction of 100. The word "percent" literally means "out of one hundred" (from the Latin per centum). Therefore, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5.
Understanding this fundamental definition is crucial to solving percentage problems. When we ask, "What is the percentage of 3 out of 10?", we are essentially asking: "If we divide 10 into 100 equal parts, how many of those parts represent 3?"
Calculating the Percentage: Method 1 - The Fraction Method
This method involves converting the given ratio into a fraction and then converting that fraction to a percentage.
-
Form the Fraction: The problem "3 out of 10" can be represented as the fraction 3/10.
-
Convert to a Decimal: To convert a fraction to a percentage, we first convert it to a decimal by dividing the numerator (3) by the denominator (10):
3 ÷ 10 = 0.3
-
Convert to a Percentage: To convert a decimal to a percentage, multiply by 100 and add the "%" symbol:
0.3 × 100 = 30%
Therefore, 3 out of 10 is 30%.
Calculating the Percentage: Method 2 - The Proportion Method
This method uses proportions to solve for the unknown percentage. A proportion is an equation that states that two ratios are equal.
-
Set up the Proportion: We can set up a proportion to solve for the percentage (x):
3/10 = x/100
-
Cross-Multiply: To solve for x, we cross-multiply:
10x = 300
-
Solve for x: Divide both sides by 10:
x = 30
Therefore, x = 30%, confirming our previous result.
Calculating the Percentage: Method 3 - Using a Calculator
Most calculators have a percentage function that simplifies the process. Simply divide 3 by 10 (resulting in 0.3) and then multiply by 100 (resulting in 30%). The calculator will automatically display the result as 30%.
Real-World Applications of Percentage Calculations
The ability to calculate percentages is essential in various real-world situations:
1. Finance and Investments:
- Interest Rates: Banks and financial institutions use percentages to calculate interest on loans and savings accounts.
- Investment Returns: Investors track the percentage growth or decline of their investments.
- Taxes: Taxes are often expressed as a percentage of income or the value of goods and services.
- Discounts and Sales: Retailers use percentages to advertise discounts and sales on merchandise.
2. Statistics and Data Analysis:
- Data Representation: Percentages are widely used to represent data in charts, graphs, and reports, making complex information easier to understand.
- Probability and Risk Assessment: Percentages help in expressing the likelihood of events and evaluating risks.
- Surveys and Polls: Survey results are often presented as percentages to show the proportion of respondents who chose particular options.
3. Everyday Life:
- Tip Calculation: Waiters and waitresses often expect a percentage of the bill as a tip.
- Shopping: Understanding sales discounts and taxes requires percentage calculations.
- Recipe Adjustments: Scaling up or down recipes often involves adjusting ingredient quantities by percentages.
- Grading Systems: Many educational institutions use percentages to represent student performance.
Expanding on the Concept: Beyond 3 out of 10
The principles used to calculate the percentage of 3 out of 10 can be applied to any ratio. Let's consider some variations:
What is the percentage of 7 out of 20?
Using the fraction method:
- Form the Fraction: 7/20
- Convert to Decimal: 7 ÷ 20 = 0.35
- Convert to Percentage: 0.35 × 100 = 35%
What is the percentage of 15 out of 50?
Using the proportion method:
- Set up the Proportion: 15/50 = x/100
- Cross-Multiply: 50x = 1500
- Solve for x: x = 30%
What is the percentage of 25 out of 25?
This is a special case where the numerator and denominator are equal. The fraction is 25/25 = 1. Converting this to a percentage: 1 × 100 = 100%. This signifies that 100% of the quantity is represented.
Advanced Percentage Calculations: Finding the Base or the Part
The previous examples focused on finding the percentage. However, percentage problems can also involve finding the base (the total amount) or the part (the specific amount represented by the percentage).
Example: Finding the Base
A store offers a 20% discount on a jacket, and the discount amount is $10. What was the original price of the jacket?
Here, we know the percentage (20%) and the part ($10), and we need to find the base (the original price). Let's use the formula:
Part = (Percentage/100) × Base
10 = (20/100) × Base
Base = 10 × (100/20) = $50
The original price of the jacket was $50.
Example: Finding the Part
A student scored 80% on a test with 50 questions. How many questions did the student answer correctly?
Here, we know the percentage (80%) and the base (50 questions), and we need to find the part (the number of correctly answered questions).
Part = (Percentage/100) × Base
Part = (80/100) × 50 = 40
The student answered 40 questions correctly.
Conclusion
Understanding percentages is a critical skill with wide-ranging applications. This article provided a comprehensive guide to calculating percentages, using various methods to solve the fundamental problem of determining the percentage of 3 out of 10 (which is 30%). We also explored how these principles extend to more complex percentage calculations, including scenarios where you need to find the base or the part. By mastering these techniques, you can confidently tackle various percentage-related problems encountered in daily life, finance, statistics, and more. The key takeaway is to understand the underlying concepts of fractions, decimals, and proportions, and to choose the calculation method best suited to the problem at hand.
Latest Posts
Latest Posts
-
Pill In The Pocket For Atrial Fibrillation
May 27, 2025
-
Which Definition Is The Best For Semipermeable Membrane
May 27, 2025
-
Who Created The First Psychology Lab
May 27, 2025
-
What Does It Mean That Neurons Are Excitable
May 27, 2025
-
Viscoelastic Materials That Lubricate Under Load
May 27, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percentage Of 3 Out Of 10 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.