What Is The Percent Of 4/10
listenit
May 25, 2025 · 5 min read
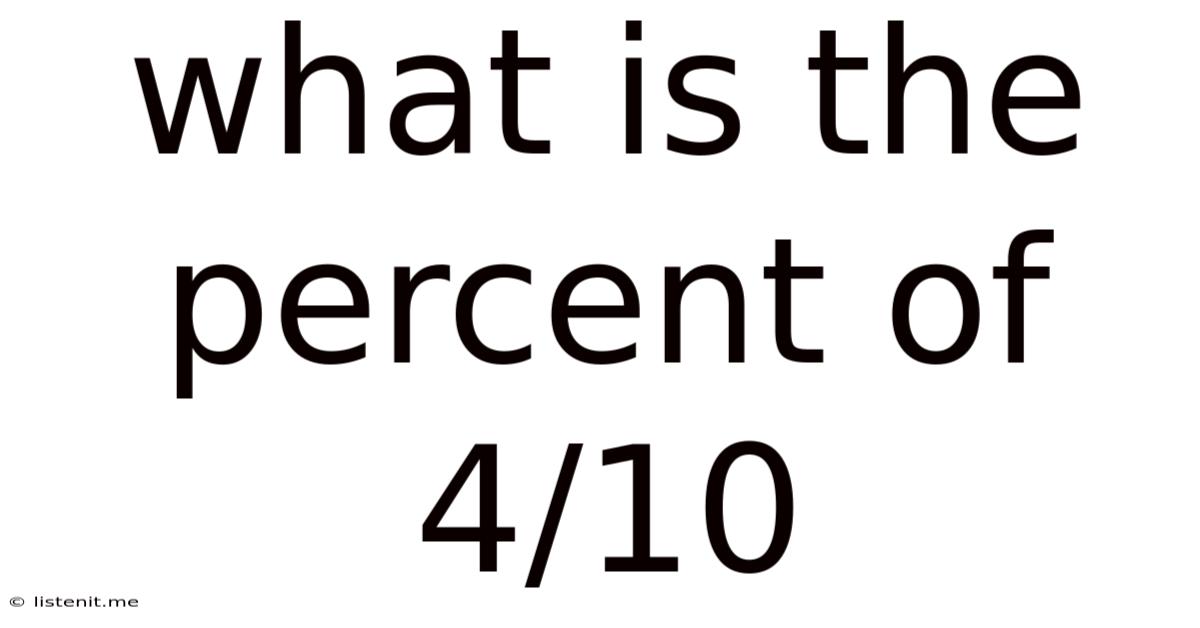
Table of Contents
What is the Percent of 4/10? A Comprehensive Guide to Fractions, Decimals, and Percentages
Understanding fractions, decimals, and percentages is fundamental to many aspects of life, from calculating tips and discounts to analyzing data and understanding financial reports. This comprehensive guide will delve into the question, "What is the percent of 4/10?", providing a thorough explanation that goes beyond a simple answer. We'll explore the underlying concepts, different methods of conversion, and practical applications.
Understanding Fractions, Decimals, and Percentages
Before we tackle the specific problem of converting 4/10 to a percentage, let's establish a solid understanding of these three interconnected concepts:
Fractions
A fraction represents a part of a whole. It consists of two numbers: a numerator (the top number) and a denominator (the bottom number). The numerator indicates how many parts you have, and the denominator indicates how many parts make up the whole. For example, in the fraction 4/10, 4 is the numerator and 10 is the denominator. This means we have 4 parts out of a total of 10 parts.
Decimals
Decimals are another way to represent parts of a whole. They use a base-10 system, with digits to the right of the decimal point representing tenths, hundredths, thousandths, and so on. Decimals are closely related to fractions; for instance, 0.4 is the decimal equivalent of 4/10.
Percentages
A percentage is a way of expressing a fraction or decimal as a portion of 100. The term "percent" literally means "out of 100." Percentages are often used to represent proportions, rates, or changes. For example, 40% means 40 out of 100.
Calculating the Percentage of 4/10
Now, let's directly address the question: what is the percentage of 4/10? There are several ways to solve this:
Method 1: Direct Conversion using a Proportion
We can set up a proportion to solve for the percentage:
4/10 = x/100
To solve for x (the percentage), we cross-multiply:
10x = 400
Divide both sides by 10:
x = 40
Therefore, 4/10 is equal to 40%.
Method 2: Converting the Fraction to a Decimal First
We can first convert the fraction 4/10 to a decimal by dividing the numerator by the denominator:
4 ÷ 10 = 0.4
Then, to convert the decimal to a percentage, we multiply by 100:
0.4 × 100 = 40%
Again, we arrive at the answer: 40%.
Method 3: Simplifying the Fraction
Before converting to a percentage, we can simplify the fraction 4/10 by dividing both the numerator and the denominator by their greatest common divisor (GCD), which is 2:
4 ÷ 2 = 2 10 ÷ 2 = 5
This simplifies the fraction to 2/5. Now we can convert this simplified fraction to a decimal:
2 ÷ 5 = 0.4
And then to a percentage:
0.4 × 100 = 40%
This demonstrates that simplifying the fraction first doesn't change the final percentage.
Real-World Applications of Percentage Calculations
Understanding how to convert fractions to percentages is crucial in many real-world situations:
Finance
- Interest rates: Interest rates on loans and savings accounts are usually expressed as percentages.
- Investment returns: The return on investments is often calculated as a percentage of the initial investment.
- Discounts and sales tax: Discounts are frequently given as percentages, and sales tax is added as a percentage of the purchase price.
- Analyzing financial statements: Financial statements use percentages extensively to compare different aspects of a company's performance over time or against industry benchmarks.
Statistics and Data Analysis
- Representing proportions: Percentages are ideal for representing proportions in data sets, making it easy to compare different groups or categories.
- Calculating rates: Rates of change, such as population growth or inflation, are often expressed as percentages.
- Creating charts and graphs: Percentages are frequently used in charts and graphs to visualize data effectively.
Everyday Life
- Calculating tips: Calculating tips at restaurants usually involves converting a percentage (e.g., 15% or 20%) to a monetary amount.
- Understanding survey results: Survey results are often presented as percentages to show the proportion of respondents who chose a particular answer.
- Comparing prices: Percentages can help compare prices between different stores or products.
Expanding on the Concept: Percentages Greater Than 100%
While 4/10 represents a percentage less than 100%, it's important to note that percentages can also be greater than 100%. This happens when the numerator is larger than the denominator in the fraction. For example, if you have a fraction like 6/5, the percentage would be:
6 ÷ 5 = 1.2
1.2 × 100 = 120%
This indicates that you have 120% of the original amount or that there's been an increase of 20% over the original value.
Advanced Applications: Compound Percentages and Percentage Change
The concept of percentages extends beyond simple conversions. Understanding compound percentages and percentage change is critical in various fields:
Compound Percentages
Compound percentages involve calculating percentages of a changing base amount. A common example is compound interest, where interest earned in one period is added to the principal, and the interest for the next period is calculated on the new total. This leads to exponential growth.
Percentage Change
Percentage change measures the relative change between an old value and a new value. It's calculated as:
[(New Value - Old Value) / Old Value] × 100%
A positive percentage change indicates an increase, while a negative percentage change indicates a decrease.
Conclusion: Mastering Percentages for Success
The seemingly simple question, "What is the percent of 4/10?", opens the door to a vast and practical understanding of fractions, decimals, and percentages. Mastering these concepts is essential for success in various aspects of life, from personal finance to professional endeavors. By understanding the different methods of conversion, applying them to real-world scenarios, and exploring more advanced concepts like compound percentages and percentage change, you can equip yourself with a powerful tool for numerical analysis and problem-solving. Remember to practice regularly, and you will soon find yourself confidently navigating the world of percentages.
Latest Posts
Latest Posts
-
48 Years Old What Year Born
May 25, 2025
-
What Is The Gcf Of 17 And 51
May 25, 2025
-
75 Feet Per Second To Mph
May 25, 2025
-
What Percent Is 4 Of 15
May 25, 2025
-
Interconverting Amount Of Radioactive Decay And Half Life
May 25, 2025
Related Post
Thank you for visiting our website which covers about What Is The Percent Of 4/10 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.